Distribution of energy in the spectrum of a black body can be correctly represented by .
Stefan's law
Kirchoff's law
Planks's law
Wien's law
The correct Answer is:C
To solve the question regarding the distribution of energy in the spectrum of a black body, we need to analyze the different laws related to black body radiation.
1. Understanding Black Body Radiation: A black body is an idealized physical object that absorbs all incident electromagnetic radiation, regardless of frequency or angle of incidence. The energy emitted by a black body is a function of its temperature and the wavelength of the radiation.
2. Reviewing the Relevant Laws:
- Stefan-Boltzmann Law: This law states that the total energy emitted per unit surface area of a black body is proportional to the fourth power of its absolute temperature (T). The formula is given by:
E=σT4
where E is the total energy emitted, σ is the Stefan-Boltzmann constant, and T is the absolute temperature. However, this law does not provide the distribution of energy across different wavelengths.
- Kirchhoff's Law: This law relates the emissive power of a body to its absorptive power at thermal equilibrium. It states that the emissive power of a body is equal to the absorptive power of a black body at the same temperature. This law does not describe the spectral distribution of energy.
- Planck's Law: This law provides a formula for the spectral distribution of energy emitted by a black body at a given temperature. It states that the energy emitted per unit wavelength is given by:
E(λ,T)=2hc2λ5⋅1ehcλkT−1
where E(λ,T) is the energy emitted at wavelength λ, h is Planck's constant, c is the speed of light, k is Boltzmann's constant, and T is the absolute temperature. This law accurately describes the distribution of energy across different wavelengths.
- Wien's Displacement Law: This law states that the wavelength at which the emission of a black body spectrum is maximized is inversely proportional to the temperature. It is given by:
λmax⋅T=b
where b is Wien's displacement constant. This law is also limited to the maximum wavelength and does not provide a complete spectral distribution.
3. Conclusion: Among the laws discussed, Planck's Law is the only one that correctly represents the distribution of energy in the spectrum of a black body across all wavelengths. Therefore, the correct answer to the question is Planck's Law.
Final Answer:
The distribution of energy in the spectrum of a black body can be correctly represented by Planck's Law.
- Stefan-Boltzmann Law: This law states that the total energy emitted per unit surface area of a black body is proportional to the fourth power of its absolute temperature (T). The formula is given by:
E=σT4
where E is the total energy emitted, σ is the Stefan-Boltzmann constant, and T is the absolute temperature. However, this law does not provide the distribution of energy across different wavelengths.
E(λ,T)=2hc2λ5⋅1ehcλkT−1
where E(λ,T) is the energy emitted at wavelength λ, h is Planck's constant, c is the speed of light, k is Boltzmann's constant, and T is the absolute temperature. This law accurately describes the distribution of energy across different wavelengths.
λmax⋅T=b
where b is Wien's displacement constant. This law is also limited to the maximum wavelength and does not provide a complete spectral distribution.
The distribution of energy in the spectrum of a black body can be correctly represented by Planck's Law.
Topper's Solved these Questions
- CALORIMETRY AND HEAT TRANSFERBook:DC PANDEY ENGLISHChapter:CALORIMETRY AND HEAT TRANSFERExercise:Taking it together
Explore 51 Videos
- CALORIMETRY AND HEAT TRANSFERBook:DC PANDEY ENGLISHChapter:CALORIMETRY AND HEAT TRANSFERExercise:Assertion and reason
Explore 17 Videos
- CALORIMETRY AND HEAT TRANSFERBook:DC PANDEY ENGLISHChapter:CALORIMETRY AND HEAT TRANSFERExercise:Check points 16.3
Explore 20 Videos
- CALORIMETRY & HEAT TRANSFERBook:DC PANDEY ENGLISHChapter:CALORIMETRY & HEAT TRANSFERExercise:Level 2 Subjective
Explore 14 Videos
- CENTRE OF MASSBook:DC PANDEY ENGLISHChapter:CENTRE OF MASSExercise:Medical entrances gallery
Explore 27 Videos
Similar Questions
Name the law which helps us to explain the distribution of energy in the spectrum of a black body for
(i) short wavelength only (ii) long wavelength only.
View SolutionState Stefan's law and Wien's displacement law. Draw graphs showing the distribution of energy in the spectrum of a black body. Explain what quantity is plotted against the wavelength . By considering how this energy distribution varies with tempaerature expalin the colour changes which occur when a piece of iron is heated from cold to near the melting point.
View Solution
Knowledge Check
Which of the following reactions are correctly represented?
AR−CH=CH2+HCl→R−CH∣Cl−CH3BR−CH=CH2+HIH2O2−−→hvR−CH2−CH2−ICR−CH=CH2+HBrH2O2−−→hvR−CH2−CH2−BrDR−CH=CH2+HIH2O2−−→hvR−I∣CH−CH3
State Stefan's law. Sketch graphs showing the distribution of energy in the spectrum of black body radiation at three temperatures, and indicate which curve corresponds to the highest temperature.
View SolutionWhat is a black body ?
View SolutionStructure of XeO2F2 is correctly represented by
View SolutionStructure of XeO2F2 is correctly represented by
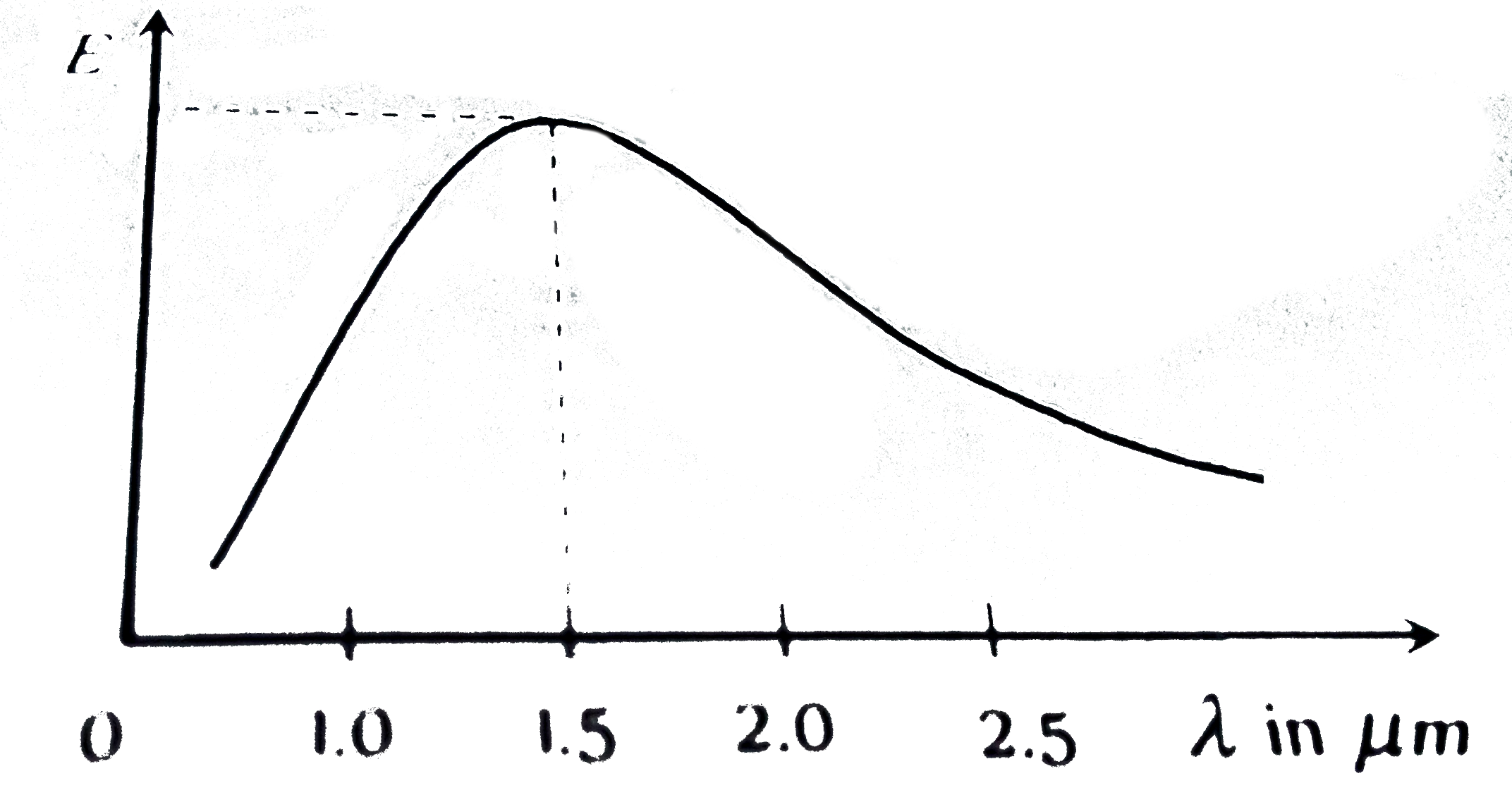
View SolutionIn the figure, the distribution of energy density of the radiation emitted by a black body at a given temperature is shown. The possible temperature of the black body is
View SolutionThe intestinal antiseptic Salol is correctly represented as:
View SolutionThe energy radiated by a black body is directly proportional to :
View Solution