Find the moment of inertia of a uniform cylinder about an axis through its centre of mass and perpendicular to its base. Mass of the cylinder is M and radius is R.
To find the moment of inertia of a uniform cylinder about an axis through its center of mass and perpendicular to its base, we can follow these steps:
Step 1: Understand the Geometry of the Cylinder
We have a uniform cylinder with mass M and radius R. The height of the cylinder is h. We need to find the moment of inertia about an axis that passes through the center of mass and is perpendicular to the base.
Step 2: Set Up the Moment of Inertia Integral
The moment of inertia I is given by the integral:
I=∫r2dm
where r is the distance from the axis of rotation to the mass element dm.
Step 3: Consider a Cylindrical Shell
To compute the moment of inertia, we can consider a thin cylindrical shell of radius r, thickness dr, and height h. The mass of this shell dm can be expressed in terms of the density and volume.
Step 4: Calculate the Mass of the Shell
The volume dV of the cylindrical shell is given by:
dV=2πrdrh
The density ρ of the cylinder is:
ρ=MπR2h
Thus, the mass of the shell dm can be calculated as:
dm=ρ⋅dV=MπR2h⋅(2πrdrh)=2MR2rdr
Step 5: Substitute dm into the Moment of Inertia Integral
Now substitute dm into the moment of inertia integral:
I=∫R0r2(2MR2rdr)
This simplifies to:
I=2MR2∫R0r3dr
Step 6: Evaluate the Integral
Now, we evaluate the integral:
∫R0r3dr=(r44)R0=R44
Substituting this back into the equation for I:
I=2MR2⋅R44=2MR24=MR22
Step 7: Final Result
Thus, the moment of inertia of the uniform cylinder about an axis through its center of mass and perpendicular to its base is:
I=MR22
We have a uniform cylinder with mass M and radius R. The height of the cylinder is h. We need to find the moment of inertia about an axis that passes through the center of mass and is perpendicular to the base.
The moment of inertia I is given by the integral:
I=∫r2dm
where r is the distance from the axis of rotation to the mass element dm.
To compute the moment of inertia, we can consider a thin cylindrical shell of radius r, thickness dr, and height h. The mass of this shell dm can be expressed in terms of the density and volume.
The volume dV of the cylindrical shell is given by:
dV=2πrdrh
The density ρ of the cylinder is:
ρ=MπR2h
Thus, the mass of the shell dm can be calculated as:
dm=ρ⋅dV=MπR2h⋅(2πrdrh)=2MR2rdr
Now substitute dm into the moment of inertia integral:
I=∫R0r2(2MR2rdr)
This simplifies to:
I=2MR2∫R0r3dr
Now, we evaluate the integral:
∫R0r3dr=(r44)R0=R44
Substituting this back into the equation for I:
I=2MR2⋅R44=2MR24=MR22
Thus, the moment of inertia of the uniform cylinder about an axis through its center of mass and perpendicular to its base is:
I=MR22
Topper's Solved these Questions
- ROTATIONBook:DC PANDEY ENGLISHChapter:ROTATIONExercise:Check point 9.1
Explore 20 Videos
- ROTATIONBook:DC PANDEY ENGLISHChapter:ROTATIONExercise:Check point 9.2
Explore 20 Videos
- RAY OPTICSBook:DC PANDEY ENGLISHChapter:RAY OPTICSExercise:Integer type q.
Explore 14 Videos
- ROTATIONAL MECHANICSBook:DC PANDEY ENGLISHChapter:ROTATIONAL MECHANICSExercise:Subjective Questions
Explore 2 Videos
Similar Questions
The moment of inertia of a copper disc, rotating about an axis passing through its centre and perpendicular to its plane
View SolutionMoment of inertia of a uniform quarter disc of radius R and mass M about an axis through its centre of mass and perpendicular to its plane is :
View SolutionMoment of inertia of a uniform quarter disc of radius R and mass M about an axis through its centre of mass and perpendicular to its plane is :
View SolutionThe moment of inertia of a uniform semicircular wire of mass m and radius r, about an axis passing through its centre of mass and perpendicular to its plane is mr2(−kπ2). Find the value of k.
View SolutionThe moment of inertia of a uniform semicircular wire of mass M and radius R about an axis passing through its centre of mass and perpendicular to its plane is xMR210. Find the value of x? (Take π2=10)
View SolutionThe moment of inertia of a solid cylinder of mass M, length 2R and radius R about an axis passing through the centre of mass and perpendicular to the axis of the cylinder is I1 and about an axis passing through one end of the cylinder and perpendicular to the axis of cylinder is I2
View SolutionMoment of inertia of a cylinder of mass M, length L and radius R about an axis passing through its centre and perpendicular to the axis of the cylinder is I=M(R24+L212). If such a cylinder is to be made for a given mass of a material, the ratio L/R for it to have minimum possible I is :
View SolutionFind the moment of inertia of a circular disk or solid cylinder of radius R about the axis through the centre and perpendicular to the flat surface.
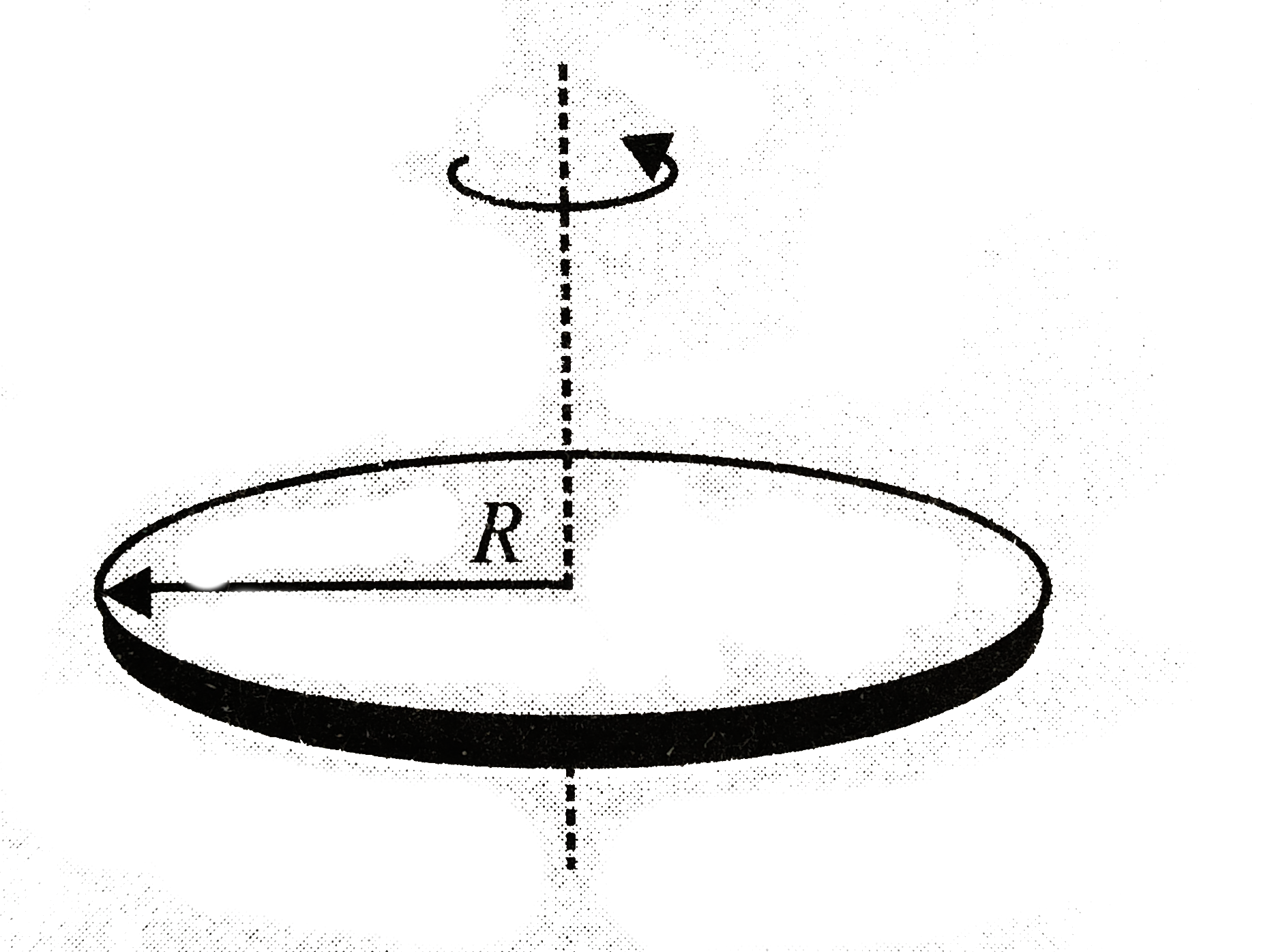
View SolutionMoment of inertia of a ring of mass M and radius R about an axis passing through the centre and perpendicular to the plane is
View SolutionThe moment of inertia of a solid cylinder about its own axis is the same as its moment of inertia about an axis passing through its centre of gravity and perpendicular to its length. The relation between its length L and radius R is :
View Solution