Assertion potential difference across, resistor, capacitor and inductor each is 10V. Then, voltage function and current functions should be in phase.
Reason At this condition current in the circuit should be maximum.
If both Assertion and Reason are true and the Reason is correct explanation of the Assertion.
If both Assertion and Reason are true but Reason is not the correct explanation of Assertion
If Assertion is true, but the Reason is false.
If Assertion is false but the Reason is true.
The correct Answer is:B
To solve the problem, we need to analyze the assertion and reason given in the question step by step.
Step 1: Understand the Assertion
The assertion states that if the potential difference across a resistor (VR), a capacitor (VC), and an inductor (VL) is each 10 V, then the voltage function and current functions should be in phase.
Step 2: Analyze the Condition
Given that:
- VR = VC = VL = 10 V
This implies that the reactance of the capacitor (XC) and the inductance (XL) must be equal for the circuit to be in resonance. Thus, we have:
- XL = XC
Step 3: Resonance Condition
At resonance, the impedance (Z) of the circuit can be expressed as:
- Z = R (where R is the resistance)
This means that the circuit behaves like a purely resistive circuit, and the phase difference (φ) between the voltage and current is zero.
Step 4: Phase Relationship
Since the phase difference φ = 0 degrees, the voltage and current functions are in phase. Therefore, the assertion is true.
Step 5: Analyze the Reason
The reason states that at this condition, the current in the circuit should be maximum.
Step 6: Current Calculation
Using Ohm's Law, the current (I) can be expressed as:
- I = V / Z
Since at resonance:
- Z = R
We can substitute this into the equation:
- I = V / R
Given that V = 10 V (the voltage across the resistor), the current will be maximum when the impedance is minimum, which occurs at resonance.
Step 7: Conclusion
Both the assertion and reason are true, but the reason does not provide a direct explanation for the assertion. Therefore, the correct answer is that both are true, but the reason is not the correct explanation of the assertion.
Final Answer
Both assertion and reason are true, but the reason is not the correct explanation of the assertion.
---
The assertion states that if the potential difference across a resistor (VR), a capacitor (VC), and an inductor (VL) is each 10 V, then the voltage function and current functions should be in phase.
Given that:
- VR = VC = VL = 10 V
- XL = XC
At resonance, the impedance (Z) of the circuit can be expressed as:
- Z = R (where R is the resistance)
Since the phase difference φ = 0 degrees, the voltage and current functions are in phase. Therefore, the assertion is true.
The reason states that at this condition, the current in the circuit should be maximum.
Using Ohm's Law, the current (I) can be expressed as:
- I = V / Z
- Z = R
- I = V / R
Both the assertion and reason are true, but the reason does not provide a direct explanation for the assertion. Therefore, the correct answer is that both are true, but the reason is not the correct explanation of the assertion.
Both assertion and reason are true, but the reason is not the correct explanation of the assertion.
Topper's Solved these Questions
- ALTERNATING CURRENTBook:DC PANDEY ENGLISHChapter:ALTERNATING CURRENTExercise:Level - 1 Objective
Explore 30 Videos
- ALTERNATING CURRENTBook:DC PANDEY ENGLISHChapter:ALTERNATING CURRENTExercise:Level - 1 Subjective
Explore 6 Videos
- ALTERNATING CURRENTBook:DC PANDEY ENGLISHChapter:ALTERNATING CURRENTExercise:Exercise 28.2
Explore 2 Videos
- ATOMSBook:DC PANDEY ENGLISHChapter:ATOMSExercise:MEDICAL ENTRANCES GALLERY
Explore 42 Videos
Similar Questions
Knowledge Check
In a series LCR circuit the voltage across an inductor, capacitor and resistor are 20 V, 20 V and 40 V respectively. The phase difference between the applied voltage and the current in the circuit is
A30∘B45∘C60∘D0∘
The phase difference between voltage and current in series L-C circuit is
View SolutionAssertion : If potential difference across two points is zero, current between these two points should be zero.
Reason : Current passing from a resistor I=VR
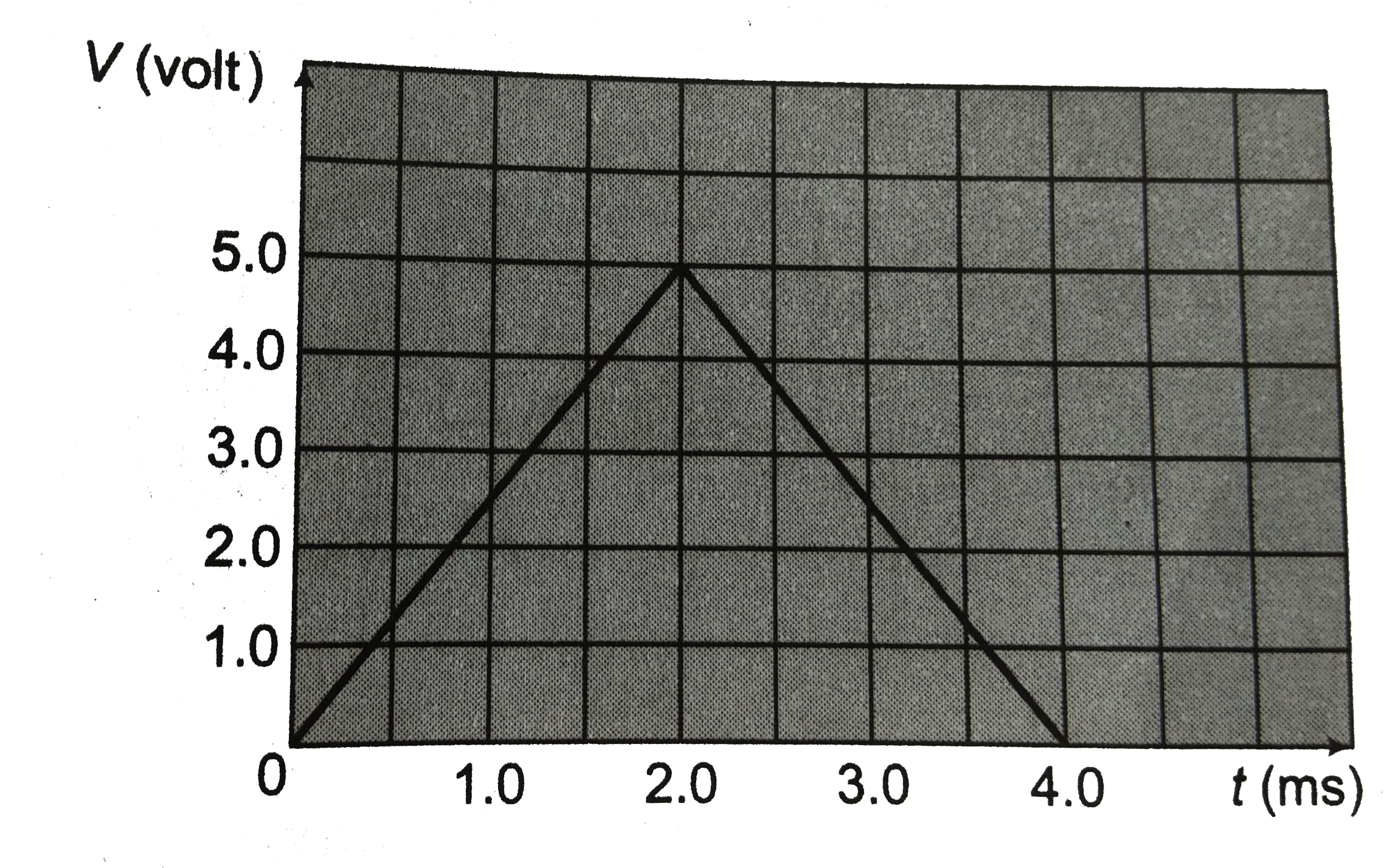
View SolutionThe potential difference across a 150mH inductor as a function of time is shown in figure. Assume that the initial value of the current in the inductor is zero. What is the current when t=2.0ms? and t=4.0ms ?
View SolutionAssertion: At resonance, power factor of series L-C-R circuit is zero.
Reason: At resonance, current function and voltage functions are in same phase.
View SolutionThe potential difference across a 2-H inductor as a function of time is shown in Fig. At time t = 0, current is zero.
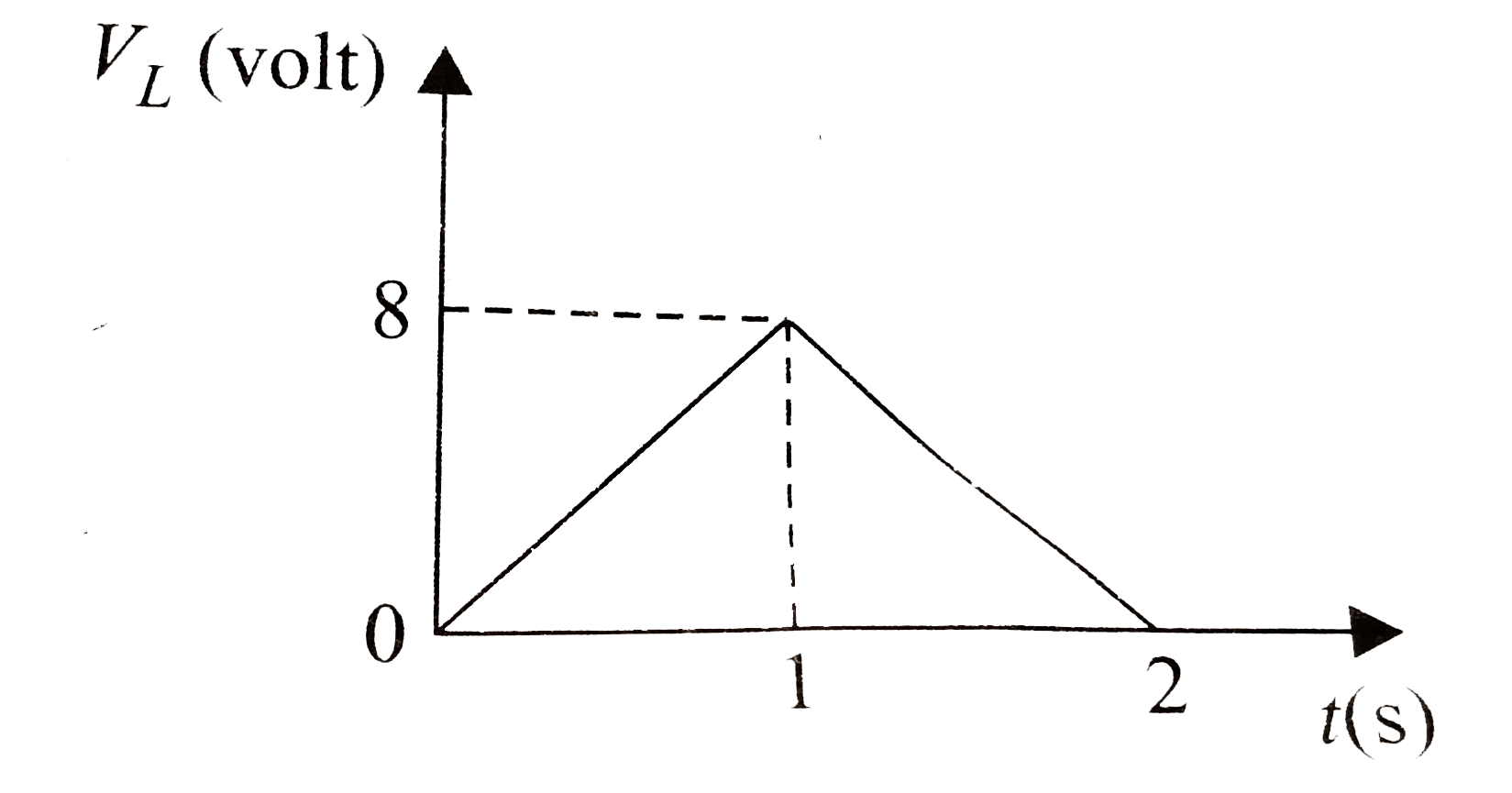
The variation of current versus time is
View SolutionThe potential difference across a 2-H inductor as a function of time is shown in Fig. At time t = 0, current is zero.
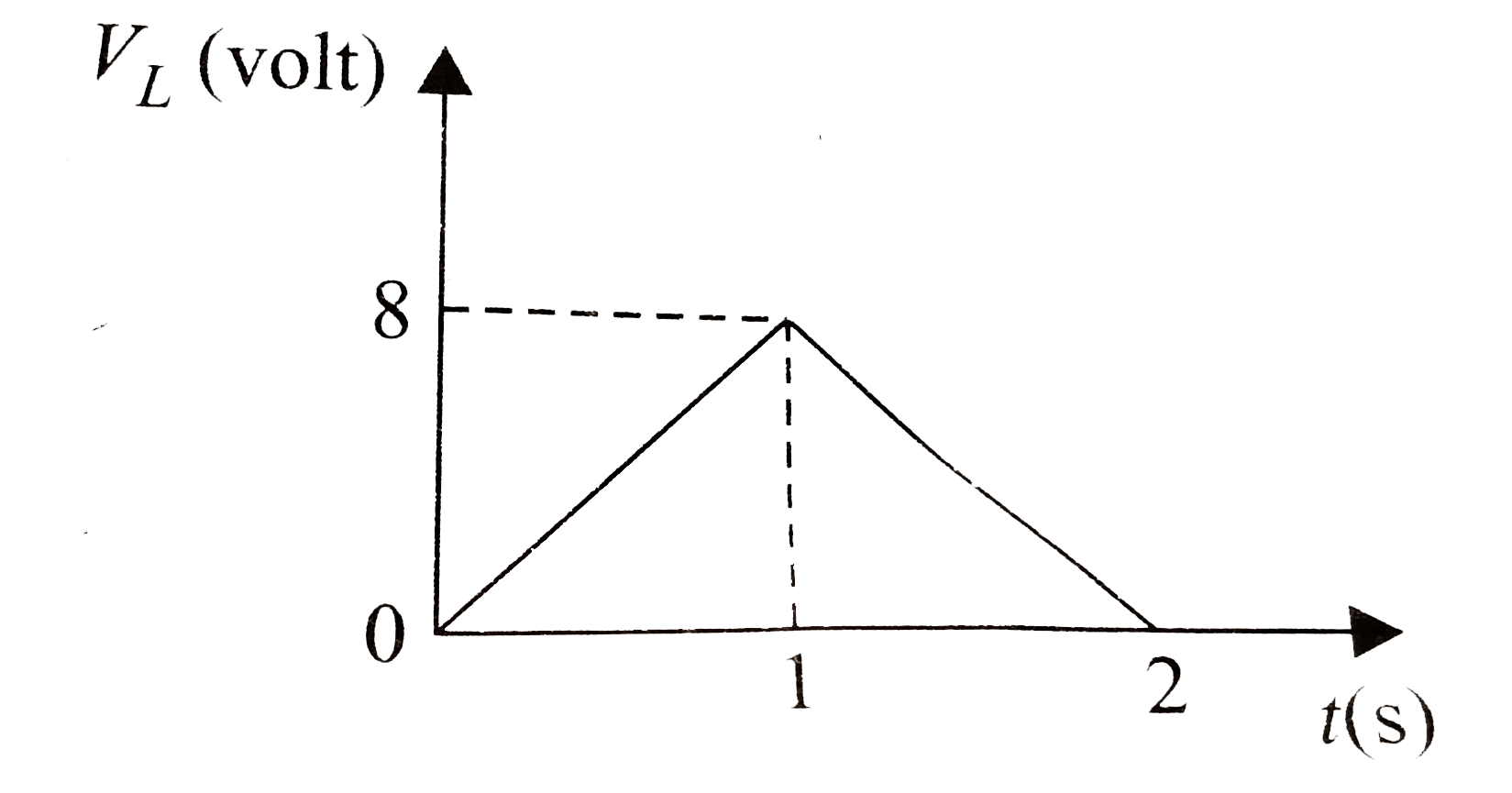
Current at t = 1 s is
View SolutionThe potential difference across a 2H inductor as a function of time is shown in figure. At time t=0, current is zero
Current t=2 second is
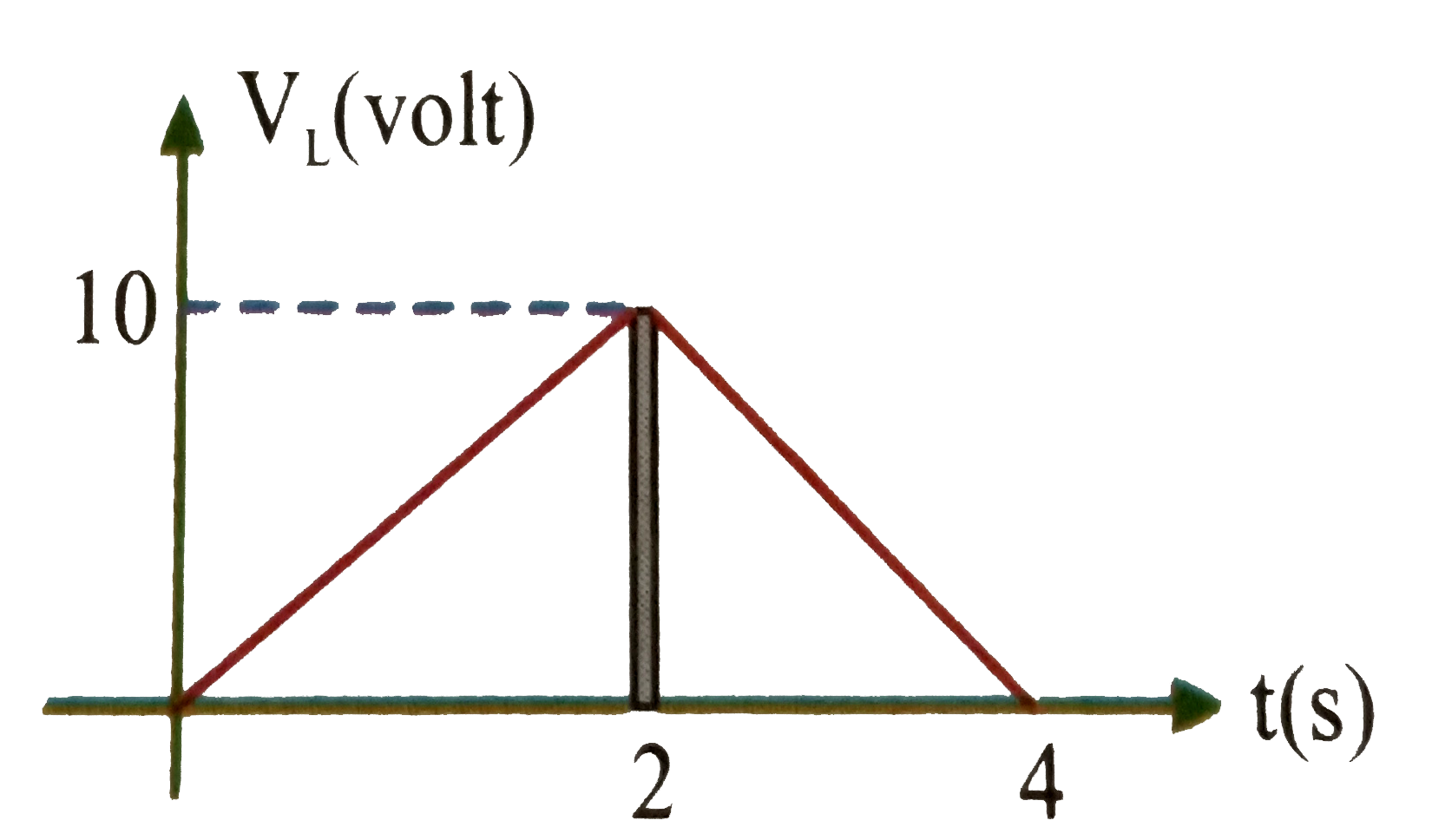
View Solution