A current of 4A flows in a coil when connected to a 12VDC source. If the same coil is connected to a 12V,50rad/sAC source, a current of 2.4A flows in the circuit. Determine the inductance of the coil. Also, find the power developed in the circuit if a2500μF capacitor is connected in series with the coil.
To solve the problem step by step, we will first determine the inductance of the coil and then calculate the power developed when a capacitor is connected in series with the coil.
Step 1: Calculate the Resistance of the Coil
When the coil is connected to a 12V DC source and a current of 4A flows, we can calculate the resistance (R) using Ohm's Law:
R=VI
Where:
- V=12V
- I=4A
Calculating:
R=12V4A=3Ω
Step 2: Calculate the Impedance of the AC Circuit
When the coil is connected to a 12V AC source with a current of 2.4A, we can calculate the impedance (Z) of the circuit:
Z=VrmsIrms
Where:
- Vrms=12V
- Irms=2.4A
Calculating:
Z=12V2.4A=5Ω
Step 3: Relate Impedance, Resistance, and Inductive Reactance
The impedance in an AC circuit can be expressed as:
Z2=R2+X2L
Where XL is the inductive reactance. Substituting the known values:
52=32+X2L
Calculating:
25=9+X2L
X2L=25−9=16
XL=√16=4Ω
Step 4: Calculate the Inductance of the Coil
The inductive reactance is related to the inductance (L) and the angular frequency (ω) by:
XL=ωL
Given that ω=50rad/s:
4=50L
Solving for L:
L=450=0.08H
Step 5: Calculate the Capacitive Reactance
Now, we will find the capacitive reactance (X_C) when a capacitor of 2500μF is connected in series with the coil. The capacitive reactance is given by:
XC=1ωC
Where:
- C=2500×10−6F
Calculating:
XC=150×2500×10−6=10.125=8Ω
Step 6: Calculate the New Impedance of the Circuit
The new impedance (Z') of the circuit with the capacitor in series is given by:
Z′=√R2+(XL−XC)2
Substituting the known values:
Z′=√32+(4−8)2
Calculating:
Z′=√9+(−4)2=√9+16=√25=5Ω
Step 7: Calculate the Average Power Developed
The average power (P) developed in the circuit can be calculated using:
P=I2rmsR
Where Irms=2.4A and R=3Ω:
P=(2.4)2×3
Calculating:
P=5.76×3=17.28W
Final Answers:
- The inductance of the coil is 0.08H.
- The average power developed in the circuit is 17.28W.
When the coil is connected to a 12V DC source and a current of 4A flows, we can calculate the resistance (R) using Ohm's Law:
R=VI
Where:
- V=12V
- I=4A
R=12V4A=3Ω
When the coil is connected to a 12V AC source with a current of 2.4A, we can calculate the impedance (Z) of the circuit:
Z=VrmsIrms
Where:
- Vrms=12V
- Irms=2.4A
Z=12V2.4A=5Ω
The impedance in an AC circuit can be expressed as:
Z2=R2+X2L
Where XL is the inductive reactance. Substituting the known values:
52=32+X2L
Calculating:
25=9+X2L
X2L=25−9=16
XL=√16=4Ω
The inductive reactance is related to the inductance (L) and the angular frequency (ω) by:
XL=ωL
Given that ω=50rad/s:
4=50L
Solving for L:
L=450=0.08H
Now, we will find the capacitive reactance (X_C) when a capacitor of 2500μF is connected in series with the coil. The capacitive reactance is given by:
XC=1ωC
Where:
- C=2500×10−6F
XC=150×2500×10−6=10.125=8Ω
The new impedance (Z') of the circuit with the capacitor in series is given by:
Z′=√R2+(XL−XC)2
Substituting the known values:
Z′=√32+(4−8)2
Calculating:
Z′=√9+(−4)2=√9+16=√25=5Ω
The average power (P) developed in the circuit can be calculated using:
P=I2rmsR
Where Irms=2.4A and R=3Ω:
P=(2.4)2×3
Calculating:
P=5.76×3=17.28W
- The inductance of the coil is 0.08H.
- The average power developed in the circuit is 17.28W.
Topper's Solved these Questions
- ALTERNATING CURRENTBOOK - DC PANDEY ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Example Type 2
1 Videos
- ALTERNATING CURRENTBOOK - DC PANDEY ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Example Type 3
1 Videos
- ALTERNATING CURRENTBOOK - DC PANDEY ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - JEE MAIN
62 Videos
- ATOMSBOOK - DC PANDEY ENGLISHCHAPTER - ATOMSEXERCISE - MEDICAL ENTRANCES GALLERY
42 Videos
Similar Questions
In the pure inductive circuit, the curves between frequency f and reciprocal of inductive reactance 1/XL is
View SolutionA current of 4 A flows in a coil when connected to a 12 V dc source. If the same coil is connected to a 12V,50rads−1 ac source, a current of 2.4 A flows of the coil in the circuit.
The power developed in the circuit of a 2500(μ)F capacitor that is connected in series with the coil is
View SolutionA current of 6 A flows in a coil when connected to a dc source of 18 V. If the same coil is connected to an ac source of 20 V, 50 rad/s, a current of 4 A flows in the circuit. The inductance of the coil is 10x mH. Find out the value of x.
View SolutionA current of 4 A flows in a coil when it is connected to a 12V dc source. When the same coil is connected to an a.c. source (12 V, 8 Hz), a current of 2.4 A flows in the coil. Calculate coefficient of self inductance (L) of the coil.
View SolutionA steady 4A flows in an inductor coil when connected to a 12V source as shown in figure. If the same coil is connected to an ac source of 12V, 50rad/s a currentd of 2.4A flows in the circuit as shown in figure2. Now after these observations , a capacitor fo capacitance 150F is connected in series with the coil and with same AC source as shown in figure 3.
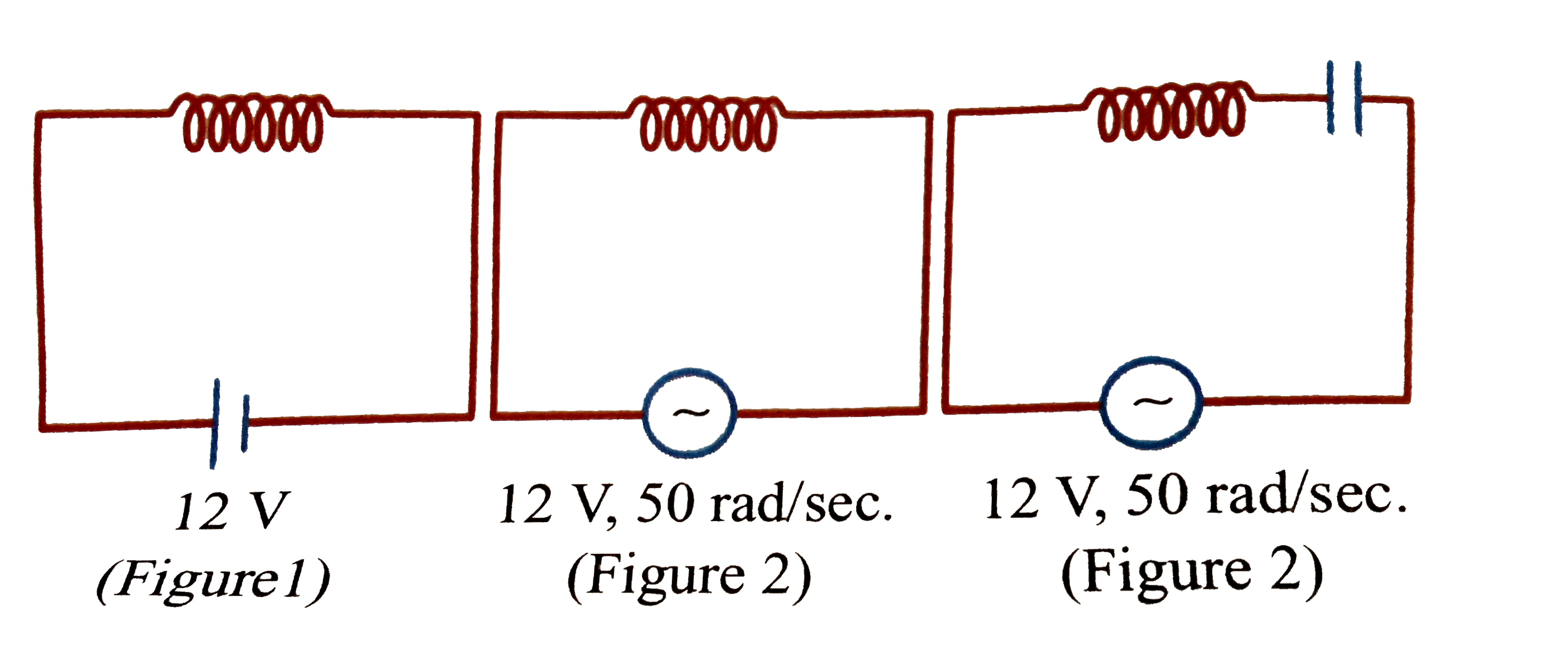
The resistance of the coil is:
View SolutionA long solenoid connected to a 12V DC source passes a steady current of 2 A. When the solenoid is connected to an AC source of 12V at 50 Hz, the current flowing is 1A. Calculate inductance of the solenoid.
View SolutionWhen a coil is connected to a 100 V D.C. supply, the current is 2 A. When the same coil is connected to A.C. source E=100√2sinωt, the current is 1 A. Find the inductive reactance used in the circuit.
View SolutionA student in a lab took a coil and connected it to a 12VDC source. He measures the steady state current in the circuit to be 4A. He then replaced the 12VDC source by a 12V,(ω=50rad/s)AC source and observes that the reading in the AC ammeter is 2.4A. He then decides to connect a 2500μF capacitor in series with the coil and calculate the average power developed in the circuit. Further he also decides to study the variation in current in the circuit (with the capacitor and the battery in series).
The value of resistance of the coil calculated by the student is
View SolutionA student in a lab took a coil and connected it to a 12VDC source. He measures the steady state current in the circuit to be 4A. He then replaced the 12VDC source by a 12V,(ω=50rads)AC source and observes that the reading in the AC ammeter is 2.4A. He then decides to connect a 2500μF capacitor in series with the coil and calculate the average power developed in the circuit. Further he also decides to study the variation in current in the circuit (with the capacitor and the battery in series).
Which of the following graph roughly matches the variation of current in the circuit (with the coil and capacitor connected in the series) when the angulr frequency is decreased from 50 rad/s to 25 rad/s?
View SolutionA student in a lab took a coil and connected it to a 12VDC source. He measures the steady state current in the circuit to be 4A. He then replaced the 12VDC source by a 12V,(ω=50rad/s)AC source and observes that the reading in the AC ammeter is 2.4A. He then decides to connect a 2500μF capacitor in series with the coil and calculate the average power developed in the circuit. Further he also decides to study the variation in current in the circuit (with the capacitor and the battery in series).
The power developed in te circuit when the capcitor of 2500 μF is connected in sereis with the coil is
View Solution
