Consider a capacitor charging circuit. Let Q1 be the charge given to the capacitor in time interval of 20ms and Q2 be the charge given in the next time interval of 20ms. Let 10μC charge be deposited in a time interval t1 and the next 10μC charges is deposited in the next time interval t2. Then
Q1>Q2,t1>t2
Q1>Q2,t1<t2
Q1<Q2,t1>t2
Q1<Q2,t1<t2
The correct Answer is:B
Step-by-Step Solution:
1. Understanding the Charging of a Capacitor:
- When a capacitor is connected to a voltage source, it charges up over time. The rate of charging is not constant; it starts off quickly and slows down as the capacitor approaches its maximum charge.
2. Defining the Charges:
- Let Q1 be the charge deposited in the first time interval t1=20ms.
- Let Q2 be the charge deposited in the next time interval t2=20ms.
- According to the problem, Q1=10μC and Q2=10μC as well.
3. Analyzing the Charging Behavior:
- Initially, the capacitor charges rapidly, meaning that during the first time interval t1, the capacitor receives a significant amount of charge quickly.
- As time progresses and the capacitor charges, the rate of charge deposition decreases, leading to a longer time required to deposit the same amount of charge.
4. Comparing Charges and Time Intervals:
- Since the first charge Q1 is deposited quickly, it can be inferred that Q1>Q2 because the second charge Q2 takes longer to deposit due to the slowing rate of charging.
- Therefore, t1<t2 since it takes more time to deposit the same amount of charge as the capacitor approaches its maximum charge.
5. Conclusion:
- From the analysis, we conclude that:
- Q1>Q2
- t1<t2
Final Result:
- The correct options are:
- Q1 is greater than Q2
- t1 is less than t2
- When a capacitor is connected to a voltage source, it charges up over time. The rate of charging is not constant; it starts off quickly and slows down as the capacitor approaches its maximum charge.
- Let Q1 be the charge deposited in the first time interval t1=20ms.
- Let Q2 be the charge deposited in the next time interval t2=20ms.
- According to the problem, Q1=10μC and Q2=10μC as well.
- Initially, the capacitor charges rapidly, meaning that during the first time interval t1, the capacitor receives a significant amount of charge quickly.
- As time progresses and the capacitor charges, the rate of charge deposition decreases, leading to a longer time required to deposit the same amount of charge.
- Since the first charge Q1 is deposited quickly, it can be inferred that Q1>Q2 because the second charge Q2 takes longer to deposit due to the slowing rate of charging.
- Therefore, t1<t2 since it takes more time to deposit the same amount of charge as the capacitor approaches its maximum charge.
- From the analysis, we conclude that:
- Q1>Q2
- t1<t2
- The correct options are:
- Q1 is greater than Q2
- t1 is less than t2
Topper's Solved these Questions
- CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - CAPACITORSEXERCISE - OBJECTIVE_TYPE
1 Videos
- CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - CAPACITORSEXERCISE - OBJECTIVE_TYPE
1 Videos
- ATOMSBOOK - DC PANDEY ENGLISHCHAPTER - ATOMSEXERCISE - MEDICAL ENTRANCES GALLERY
42 Videos
- COMMUNICATION SYSTEMBOOK - DC PANDEY ENGLISHCHAPTER - COMMUNICATION SYSTEMEXERCISE - Subjective
11 Videos
Similar Questions
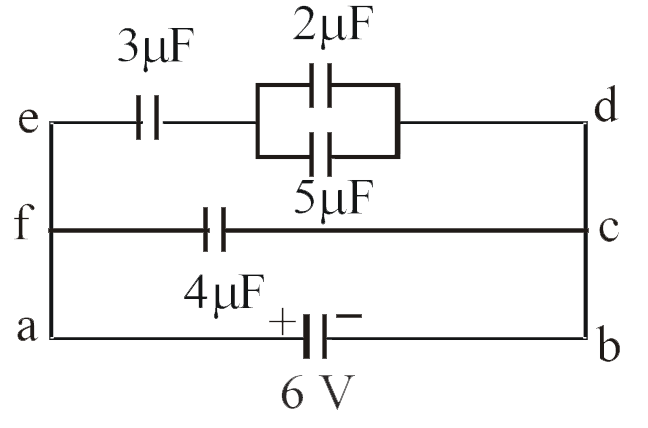
In the circuit given below, the charge in μC, on the capacitor having 5μF is
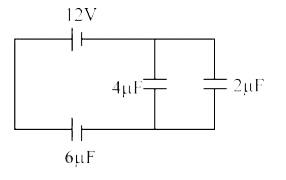
View SolutionThe charge deposited on 4μF capacitor in the given circuit is:
View SolutionThere is no resistance in the capacitive circuit shown. Then charge on the capacitor at an time t

View SolutionThe charge on 4μFcapacitor in the given circuit is (inμC)

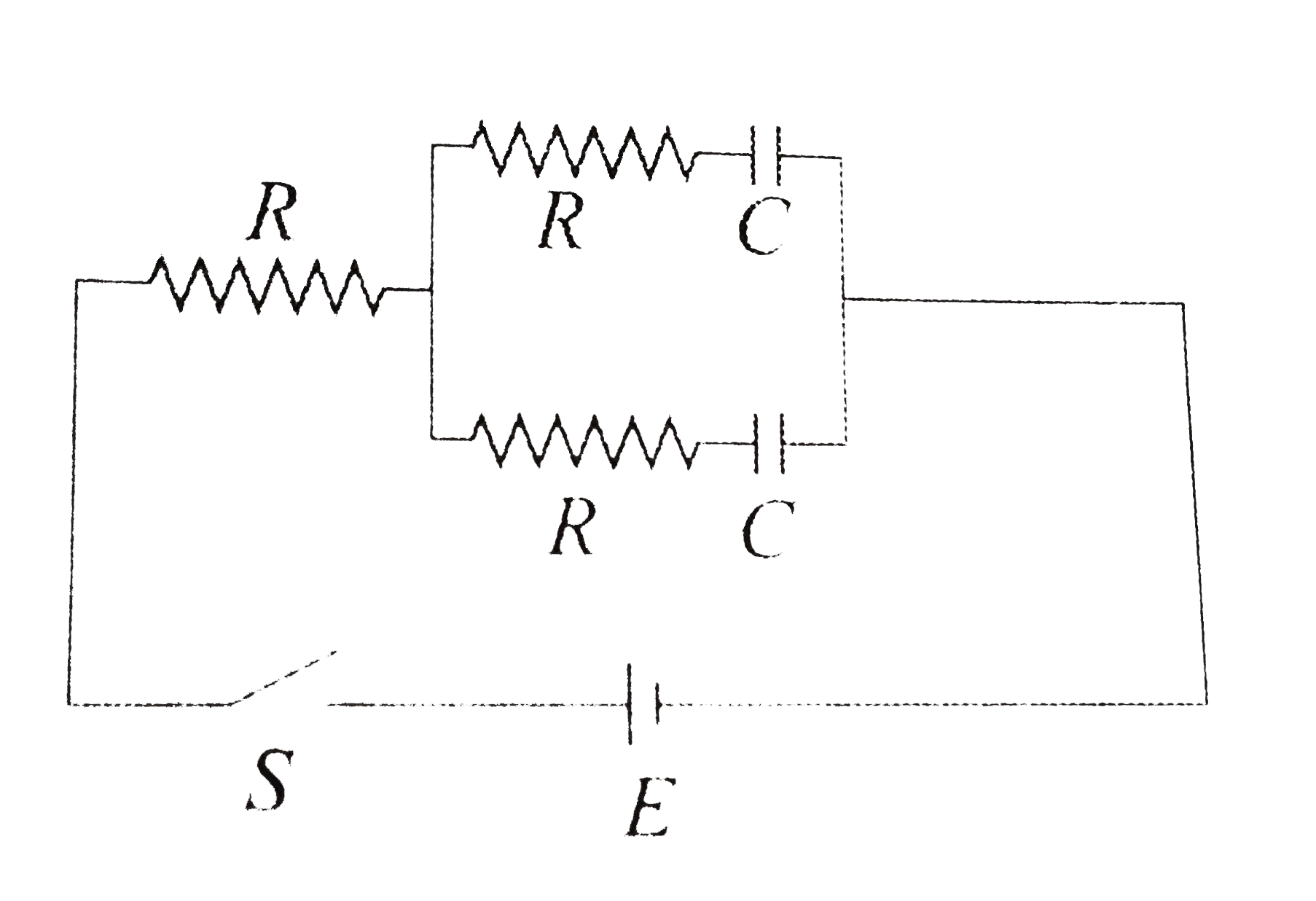
View SolutionThe switch S in the circuit diagram is closed at t = 0. The charge on capacitors at any time is
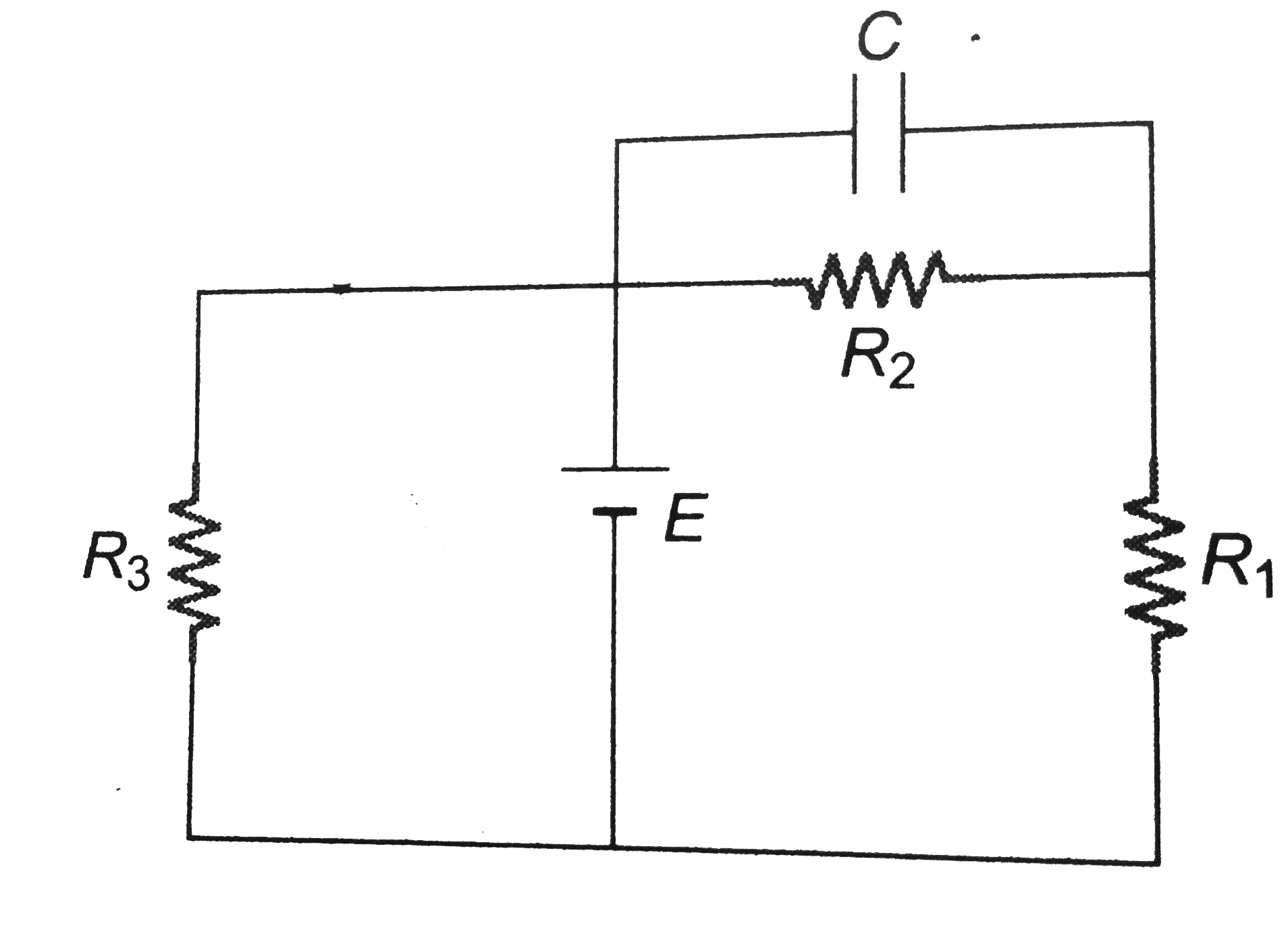
View SolutionThe charge on the capacitor is initially zero. Find the charge on the capacitor as a function of time t. All resistors are of equal value R.
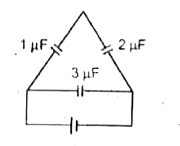
View SolutionIn given circuit, ratio of charge on 2 μF capacitor to the charge of 3 μ F capacitor will be
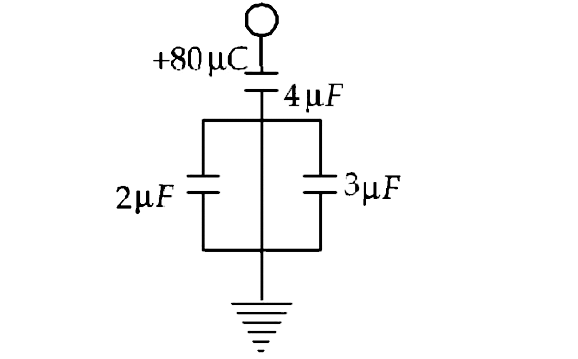
View SolutionIn the given circuit, a charge of +80μC is given to the upper plate of the 4μF capacitor. Then in the steady state, the charge on the upper plate of the 3μF capacitor is
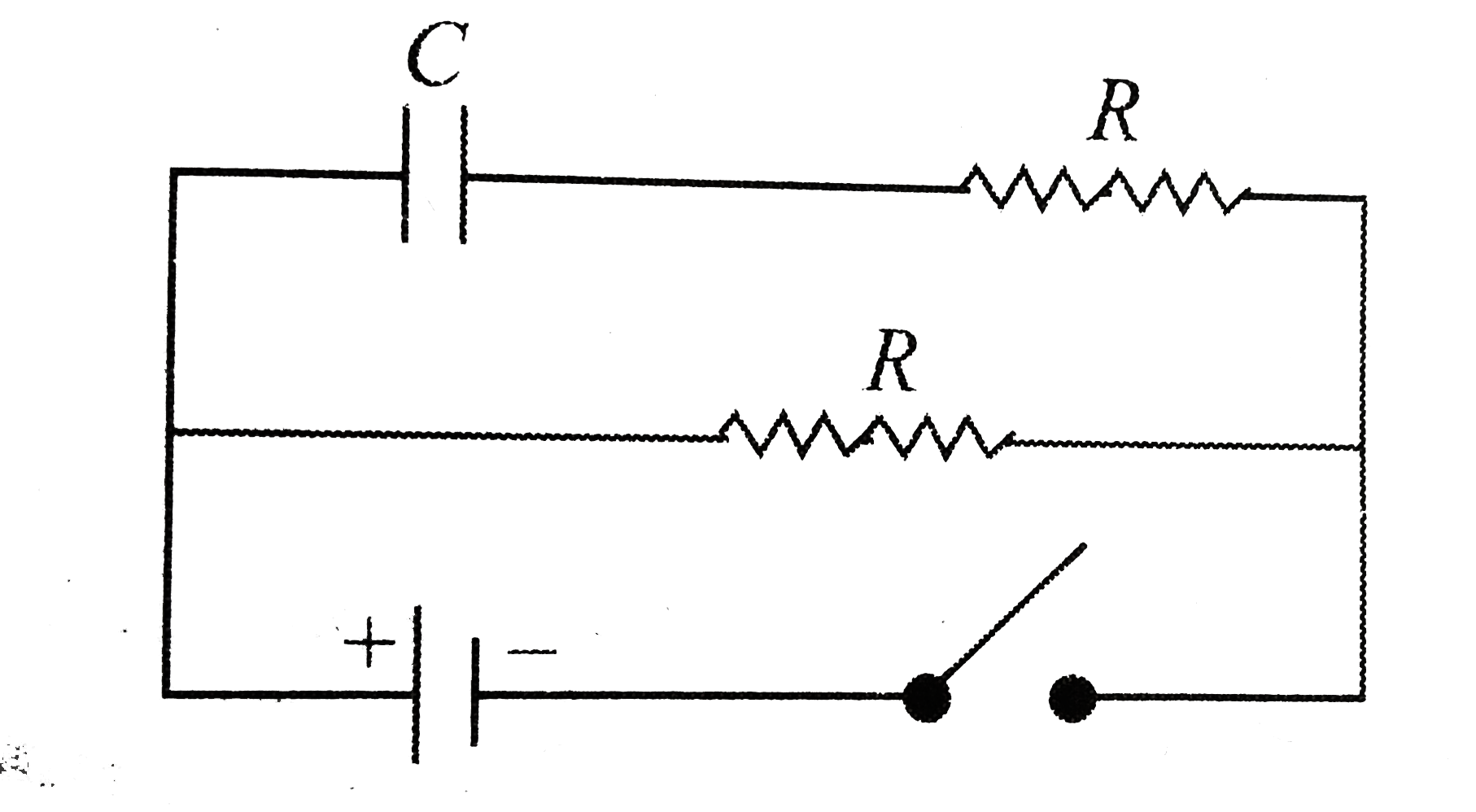
View SolutionIn the circuit shown in fig. when the switch is closed, the capacitor charges with a time constant
View SolutionIn the circuit shown, the capacitor C1 is initially charged with charge q0. The switch S is closed at time t = 0 . The charge on C2 after time t is

View Solution
