Two, capacitors A and B are connected in series across a 100V supply and it is observed that the potential difference across them are 60V and 40V. A capacitor of 2pF capacitance is now connected in parallel with A and the potential difference across B rises to 90V. Determine the capacitance of A and B
The correct Answer is:B, D
In series, potential difference distributes in the inverse ratio of capacitance.
∴VAVB=CBCA=C2C1=6040=32
∴C2=1.5C1………i
Now, V′AV′B=C′BC′A
or 1090=C2(C1+2)
or C1+2=9C2……..ii
Solving eqn i and ii we get
C1=0.16μF
and C2=0.24μF
Related Playlists
- BOOK - DC PANDEY ENGLISHCHAPTER - CAPACITORSEXERCISE - OBJECTIVE_TYPE1videos
CAPACITORS
- BOOK - DC PANDEY ENGLISHCHAPTER - CAPACITORSEXERCISE - OBJECTIVE_TYPE1videos
CAPACITORS
- BOOK - DC PANDEY ENGLISHCHAPTER - ATOMSEXERCISE - MEDICAL ENTRANCES GALLERY42videos
ATOMS
- BOOK - DC PANDEY ENGLISHCHAPTER - COMMUNICATION SYSTEMEXERCISE - Subjective11videos
COMMUNICATION SYSTEM
Similar Questions
Potential difference across AB , i.e., VA−VB is
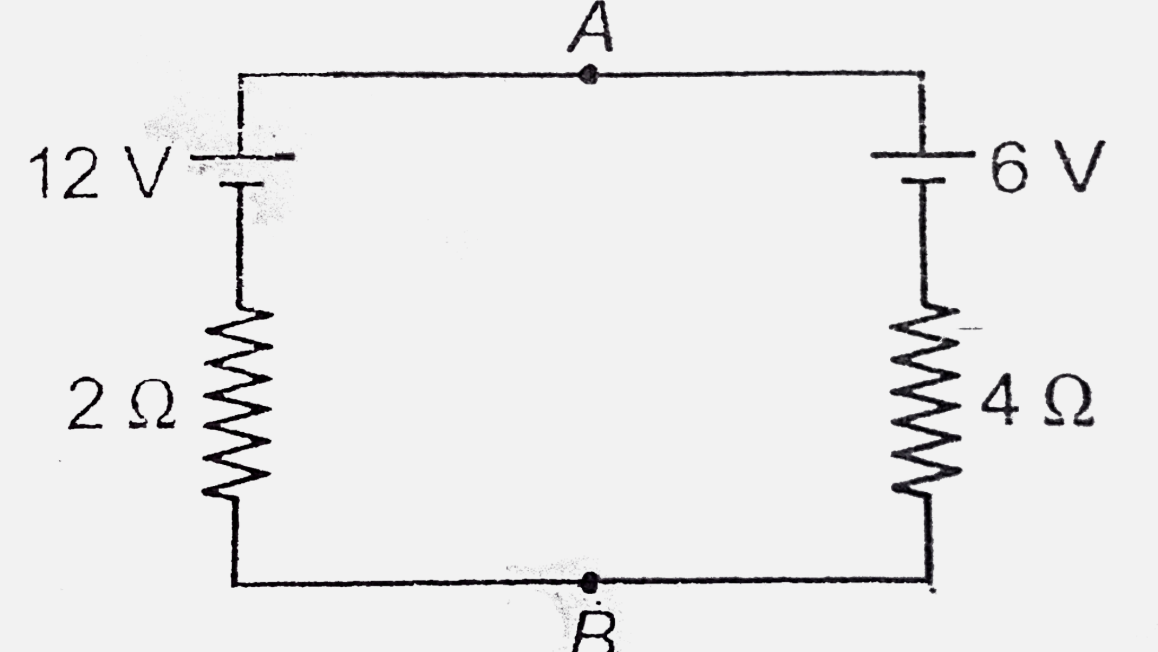 View Solution
View SolutionTwo capacitors of capacitance 6µF and 4 µF are put in series across a 120 V battery. What is the potential difference across the 4 µF capacitor. (a) 72 V (b) 60 V (c) 48 V (d) zero
View SolutionTwo capacitors of capacitance 6µF and 4 µF are put in series across a 120 V battery. What is the potential difference across the 4 µF capacitor. (a) 72 V (b) 60 V (c) 48 V (d) zero
View SolutionA 220 V AC supply is connected between points A and B . What will be the potential difference V across the capacitor ?
 View Solution
View SolutionA 220 V AC supply is connected between points A and B . What will be the potential difference V across the capacitor ?
 View Solution
View SolutionA 220 V AC supply is connected between points A and B . What will be the potential difference V across the capacitor ?
 View Solution
View SolutionRows of capacitors 1,2,4,8…..∞ capacitors, each of capacitance 2μF, are connected in parallel as shown in figure. The potential difference across AB = 10 V, then
 View Solution
View SolutionThree capacitors 3μF,6μF and 6μF are connected in series to source of 120 V. The potential difference in volt, across the 3μF capacitor will be
View SolutionTwo capacitors of capacitances 20.0pF and 50.0pF are connected in series with a 6.00V bettery . Find (a )the potential difference across each capacitor and (b) the energy stored in each capacitor .
View SolutionIf A is connerted with D and B is connected with C find the potential difference a across each capacitor and the final charge in each capacitor.
View Solution


