The separation between the plates of a charged parallel-plate capacitor is increased. The force between the plates
increases
decreases
remains same
first increases then decreases
The correct Answer is:C
To solve the problem regarding the effect of increasing the separation between the plates of a charged parallel-plate capacitor on the force between the plates, we can follow these steps:
1. Understand the Concept of Force in a Capacitor:
The force F between the plates of a parallel-plate capacitor can be expressed using the formula:
F=Q22ϵ0A
where:
- Q is the charge on the plates,
- ϵ0 is the permittivity of free space,
- A is the area of the plates.
2. Identify Variables:
In this equation, we notice that the force F depends on the charge Q, the permittivity ϵ0, and the area A of the plates. Importantly, there is no term that includes the distance D (the separation between the plates).
3. Analyze the Effect of Increasing Separation:
When the separation D between the plates is increased, we need to determine how this affects the force F. Since D does not appear in the equation for force, it indicates that the force is independent of the distance between the plates.
4. Conclusion:
Therefore, if the separation between the plates is increased, the force between the plates remains unchanged. The correct answer is that the force remains the same.
Final Answer:
The force between the plates remains the same when the separation is increased.
---
The force F between the plates of a parallel-plate capacitor can be expressed using the formula:
F=Q22ϵ0A
where:
- Q is the charge on the plates,
- ϵ0 is the permittivity of free space,
- A is the area of the plates.
In this equation, we notice that the force F depends on the charge Q, the permittivity ϵ0, and the area A of the plates. Importantly, there is no term that includes the distance D (the separation between the plates).
When the separation D between the plates is increased, we need to determine how this affects the force F. Since D does not appear in the equation for force, it indicates that the force is independent of the distance between the plates.
Therefore, if the separation between the plates is increased, the force between the plates remains unchanged. The correct answer is that the force remains the same.
The force between the plates remains the same when the separation is increased.
Topper's Solved these Questions
- CAPACITORSBook:DC PANDEY ENGLISHChapter:CAPACITORSExercise:OBJECTIVE_TYPE
Explore 1 Video
- CAPACITORSBook:DC PANDEY ENGLISHChapter:CAPACITORSExercise:OBJECTIVE_TYPE
Explore 1 Video
- ATOMSBook:DC PANDEY ENGLISHChapter:ATOMSExercise:MEDICAL ENTRANCES GALLERY
Explore 42 Videos
- COMMUNICATION SYSTEMBook:DC PANDEY ENGLISHChapter:COMMUNICATION SYSTEMExercise:Subjective
Explore 11 Videos
Similar Questions
Knowledge Check
As the distance between the plates of a parallel plate capacitor decreased
AIf both assertion and reason are ture and reason is the correct explanation of assertion.BIf both assertin and reason are ture but reason is not the correct explanation of assertion .CIf assertion is true but reason is false.DIf both assertion and reason are false.
STATEMENT-1 : If distance between plates of charged and isolated parallel plate capacitor increases then force between plates decreases.
and
STATEMENT-2 : Force between two point charges is inversely proportional to the square of distance of separation.
View SolutionThe separation between the plates of a parallel-plate capacitor is 2 mm and the area of its plates is 5cm2. If the capacitor is charged such that it has 0.01J energy stored in it, the electrostatic force of attraction between its plates is _______N.
View SolutionA slab of copper of thickness y is inserted between the plates of parallel plate capacitor as shown in figure. The separation between the plates is d. If y=d4, then the ratio of capacitance of the capacitor after nd before inserting the slab is

View SolutionWhat is the area of the plates of a 2 farad parallel plate air capacitor, given that the separation between the plates is 0.5 cm?
View SolutionWhat is the area of the plates of a 2 farad parallel plate air capacitor, given that the separation between the plates is 0.5 cm?
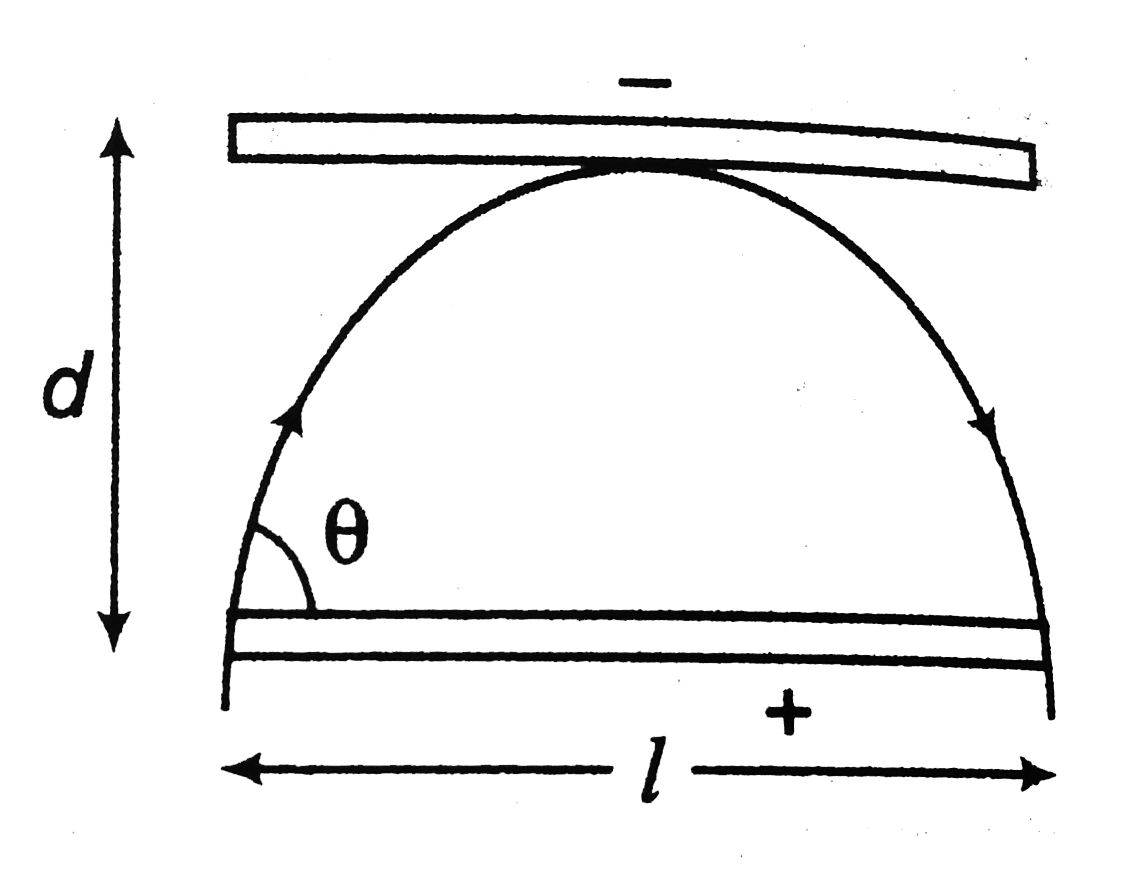
View SolutionAn electron enters the region between the plates of a parallel plate capacitor at an angle θ to the plates. The plate width is l, the plate separation is d. The electron follows the path shown just missing the upper plate. Neglect gravity. Then,
View SolutionSeparation between the plates of a parallel plate capacitor is d and the area of each plates is A. When a slab of material of dielectric constant k and thickness t(t<d) is introduced between the plates. Its capacitance becomes
View Solution