Question

A particle of mass m and charge $-q$ is projected from the origin with a horizontal speed v into an electric field of intensity E directed downward. Choose the wrong statement. Neglect gravity

Options

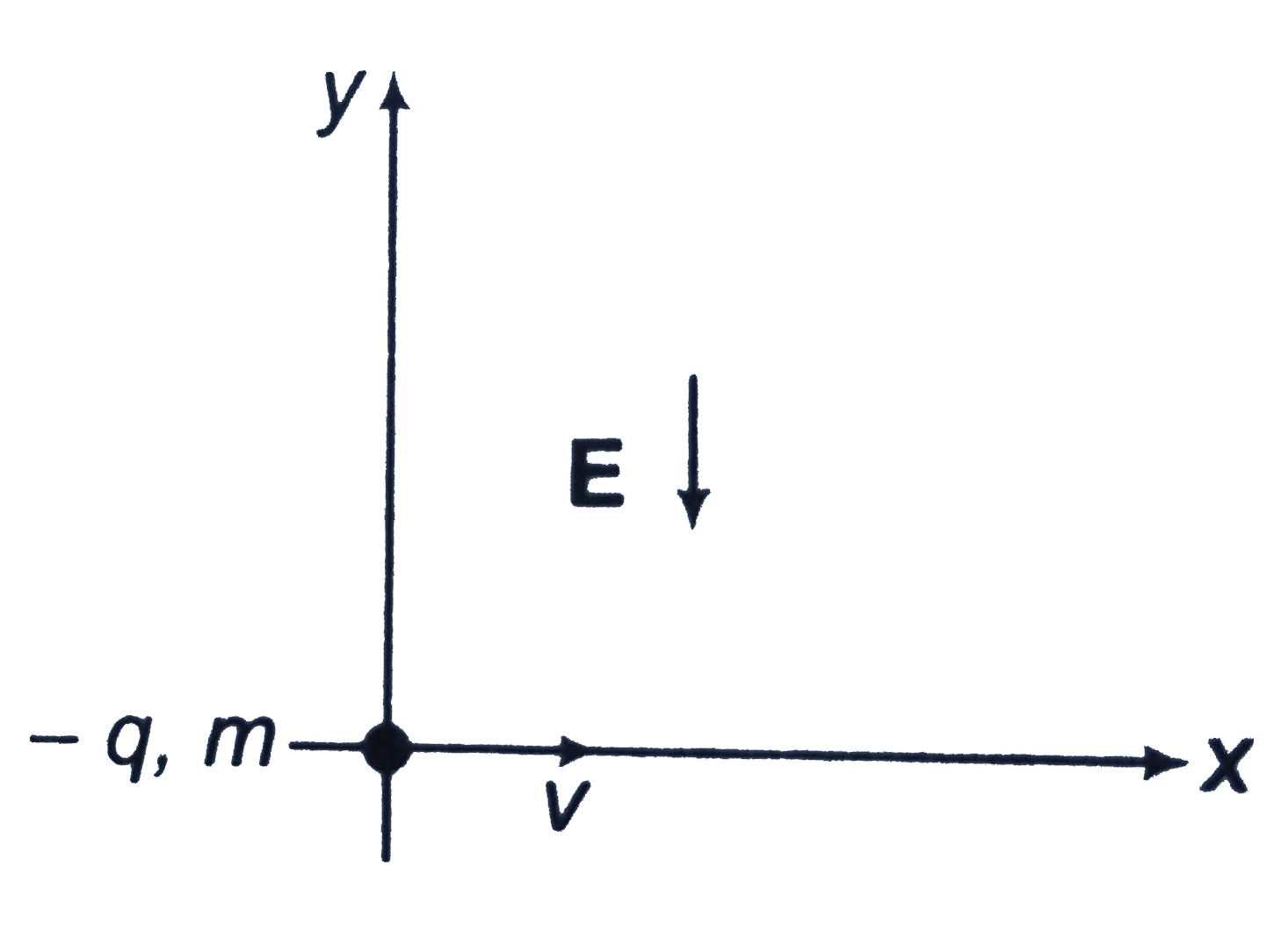
A. The kinetic energy after a displacement y is `qEy`
B. The horizontal and vertical components of acceleration of `a_x=0,a_y=(qE)/m`
C. the equation of trajectory is `y=1/2((qEx^2)/(mv^2))`
D. The horizontall and verticasl displacements x and y after a time t x=vt and `y=1/2a_yt^2`

Answer

A

Explanation

`F_x=0`
`:. a_=0`
`F_y=qE`
`:. a_y=(qE)/m`
`x=vt` and `y=1/2a_yt^2=1/2((qE)/m)t^2`
Substituting `t=x/v` in expression of `y` we get
`y=1/2((qEx^2)/(mv^2))`
`KE=1/2 m(v_x^2+v_y^2)`
where `v_x=v` and `v_h=a_yt=(qE)/mt`