A point charge q is placed at the origin. Calculate the electric flux through the open hemispherical surface (x−a)2+y2+z2=a2,x≥a
The correct Answer is:A, B
To calculate the electric flux through the open hemispherical surface defined by the equation (x−a)2+y2+z2=a2 for x≥a, we can follow these steps:
1. Understanding the Geometry:
- A point charge q is placed at the origin (0,0,0).
- The open hemispherical surface is centered at (a,0,0) with a radius a and extends in the positive x direction.
2. Applying Gauss's Law:
- According to Gauss's Law, the total electric flux Φ through a closed surface is given by:
Φ=Qencϵ0
- Here, Qenc=q (the charge at the origin) and ϵ0 is the permittivity of free space.
3. Calculating the Total Flux:
- The total flux through a closed surface surrounding the charge q (like a full sphere) is:
Φtotal=qϵ0
4. Finding the Flux through the Open Hemisphere:
- The open hemisphere only covers half of the total solid angle of a sphere. The solid angle for a full sphere is 4π steradians, so the solid angle for a hemisphere is 2π steradians.
- Therefore, the flux through the open hemisphere is half of the total flux:
Φhemisphere=12⋅qϵ0=q2ϵ0
5. Considering the Orientation:
- Since the hemisphere is open and oriented towards the positive x direction, the flux through the flat circular base of the hemisphere does not contribute to the total flux calculation, as it does not enclose any charge.
Final Answer:
The electric flux through the open hemispherical surface is:
Φ=q2ϵ0
- A point charge q is placed at the origin (0,0,0).
- The open hemispherical surface is centered at (a,0,0) with a radius a and extends in the positive x direction.
- According to Gauss's Law, the total electric flux Φ through a closed surface is given by:
Φ=Qencϵ0
- Here, Qenc=q (the charge at the origin) and ϵ0 is the permittivity of free space.
- The total flux through a closed surface surrounding the charge q (like a full sphere) is:
Φtotal=qϵ0
- The open hemisphere only covers half of the total solid angle of a sphere. The solid angle for a full sphere is 4π steradians, so the solid angle for a hemisphere is 2π steradians.
- Therefore, the flux through the open hemisphere is half of the total flux:
Φhemisphere=12⋅qϵ0=q2ϵ0
- Since the hemisphere is open and oriented towards the positive x direction, the flux through the flat circular base of the hemisphere does not contribute to the total flux calculation, as it does not enclose any charge.
The electric flux through the open hemispherical surface is:
Φ=q2ϵ0
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Level 1 Subjective
15 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - SUBJECTIVE_TYPE
6 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Level 1 Assertion And Reason
19 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions
135 Videos
Similar Questions
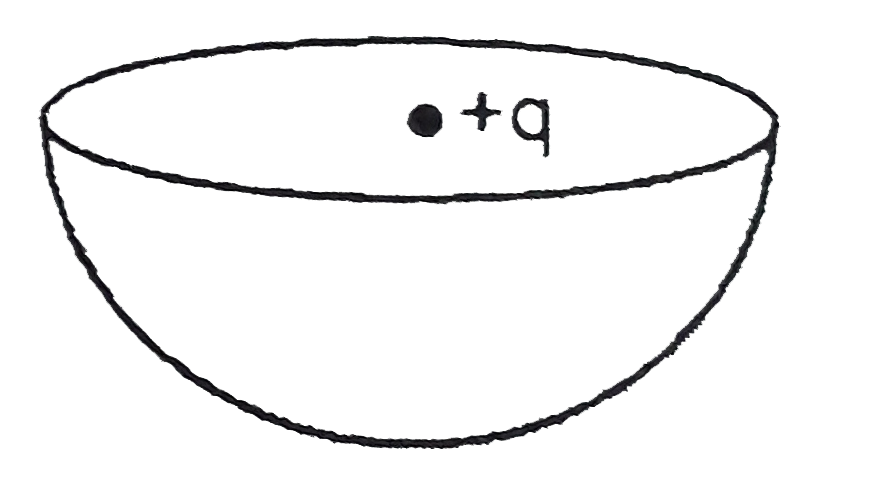
A point charge +q is placed at the centre of curvature of a hemisphere. Find flux through the hemispherical surface.
View SolutionA charge q is placed at the centre of a cube. What is the electric flux passing through the cube?
View SolutionCharge of 2 C is placed at the centre of a cube. What is the electric flux passing through one face ?
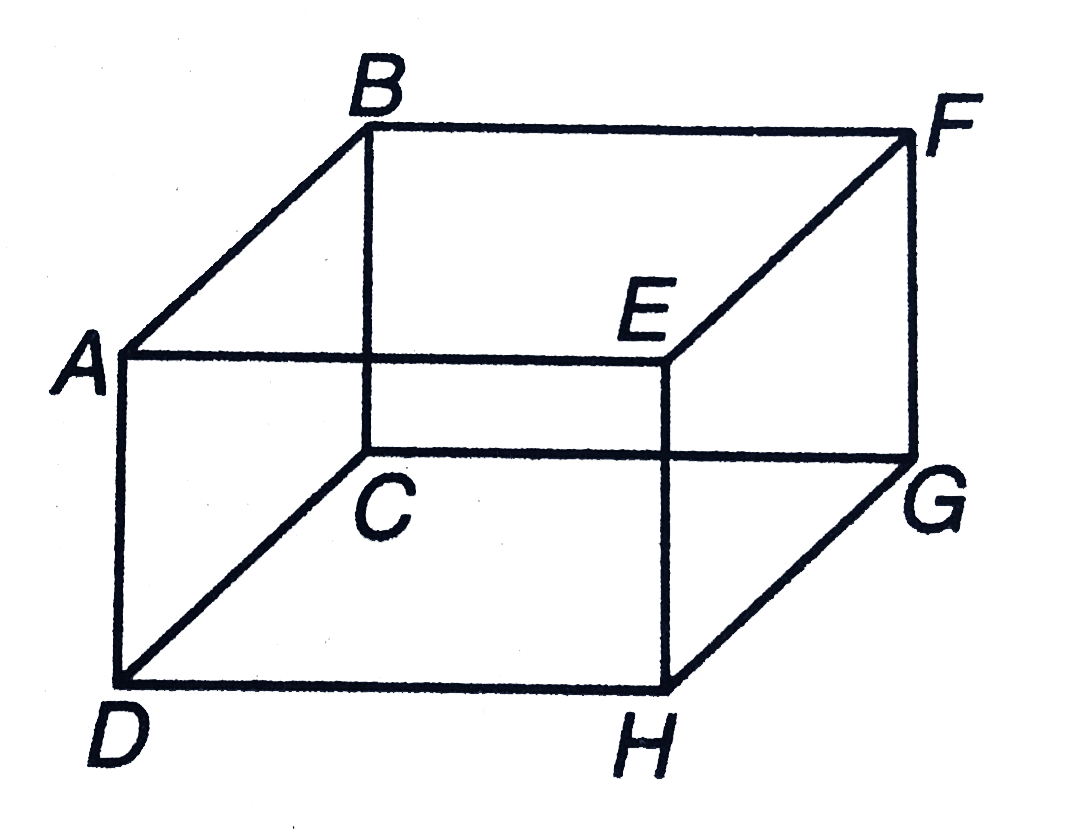
View SolutionA charge q is placed at point D of the cube. Find the electric flux passing through the EFGH and face AEHD.
View SolutionA point charge q is placed at the origin . How does the electric field due to the charge very with distance r from the origin ?
View SolutionA charge Q is placed at the centre of a cube. Find the flux of the electric field through the six surfaced of the cube.
View SolutionA charge +q is placed at the mid point of a cube of side L. The electric flux emerging from cube is
View SolutionA charge q=−2.0μC is placed at origin. Find the electric field at (3m,4m,0).
View SolutionA point charge of 0.009μC is placed at origin.
Calculate intensity of electric field due to this point charge at point (√2,√7,0).
View SolutionA point charge q is placed near hemisperical suface without base, cylindrical surface with one end open sperical surface and hemispherical surface with base as shown in the figure in (a) (b) (c) and (d) respectively In wihich of the following cases m the flux crossing through the surface is zero ?

View Solution
