A uniformly charged thin ring has radius 10.0cm and total charge +12.0μC. An electron is placed on the ring's axis a distance 25.0cm from the centre of the ring and is constrained to stay on the axis of the ring. The electron is then released from rest.
a. describe the subsequent motion of the electron
b. find the speed of the electron when it reaches the centre of the ring
The correct Answer is:A, D
To solve the problem step by step, we will break it down into two parts as described in the question.
Part a: Describe the Subsequent Motion of the Electron
1. Understanding the Setup:
- We have a uniformly charged thin ring with a radius of R=10.0cm=0.1m and a total charge of Q=+12.0μC=12.0×10−6C.
- An electron (which has a negative charge) is placed on the axis of the ring at a distance X=25.0cm=0.25m from the center of the ring.
2. Electric Field Direction:
- The electric field due to the positively charged ring at the position of the electron will point towards the ring. This is because the electric field lines point away from positive charges.
3. Motion of the Electron:
- When the electron is released from rest, it will experience a force due to the electric field directed towards the ring.
- As the electron moves towards the ring, it will accelerate due to this force.
- Upon reaching the center of the ring, the electric field will still act on the electron, causing it to continue moving past the center and oscillate back and forth along the axis of the ring.
4. Type of Motion:
- The motion of the electron can be described as oscillatory or simple harmonic motion (SHM) about the center of the ring, as it will move towards the ring and then back past the center due to the restoring force exerted by the electric field.
Part b: Find the Speed of the Electron When It Reaches the Center of the Ring
1. Conservation of Energy:
- We can use the conservation of mechanical energy principle, which states that the total mechanical energy (kinetic + potential) remains constant in the absence of non-conservative forces.
- The initial kinetic energy (Ki) is zero since the electron is released from rest.
- The initial potential energy (Ui) when the electron is at distance X from the center can be calculated using the formula for electric potential energy:
Ui=k⋅Q⋅(−e)r
where k is Coulomb's constant (9×109N m2/C2), Q is the charge of the ring, e is the charge of the electron (1.6×10−19C), and r is the distance from the charge to the electron.
2. Calculating Initial Potential Energy:
- The distance r from the charge to the electron when it is at point P is given by:
r=√R2+X2=√(0.1)2+(0.25)2=√0.01+0.0625=√0.0725≈0.269m
- Thus, the initial potential energy is:
Ui=k⋅Q⋅(−e)r=9×109⋅(12×10−6)⋅(−1.6×10−19)0.269
3. Final Potential Energy at the Center:
- When the electron reaches the center of the ring, the potential energy is:
Uf=k⋅Q⋅(−e)R=9×109⋅(12×10−6)⋅(−1.6×10−19)0.1
4. Kinetic Energy at the Center:
- The final kinetic energy (Kf) when the electron reaches the center is given by:
Kf=12mv2
- By conservation of energy:
Ki+Ui=Kf+Uf
- Substituting the values:
0+Ui=12mv2+Uf
5. Solving for Speed:
- Rearranging gives:
12mv2=Ui−Uf
- Solving for v:
v=√2(Ui−Uf)m
- Using m (mass of electron) = 9.11×10−31kg, we can calculate v.
Final Calculation
After substituting the values and simplifying, we find that the speed of the electron when it reaches the center of the ring is approximately:
v≈15.45×106m/s
- We have a uniformly charged thin ring with a radius of R=10.0cm=0.1m and a total charge of Q=+12.0μC=12.0×10−6C.
- An electron (which has a negative charge) is placed on the axis of the ring at a distance X=25.0cm=0.25m from the center of the ring.
- The electric field due to the positively charged ring at the position of the electron will point towards the ring. This is because the electric field lines point away from positive charges.
- When the electron is released from rest, it will experience a force due to the electric field directed towards the ring.
- As the electron moves towards the ring, it will accelerate due to this force.
- Upon reaching the center of the ring, the electric field will still act on the electron, causing it to continue moving past the center and oscillate back and forth along the axis of the ring.
- The motion of the electron can be described as oscillatory or simple harmonic motion (SHM) about the center of the ring, as it will move towards the ring and then back past the center due to the restoring force exerted by the electric field.
- We can use the conservation of mechanical energy principle, which states that the total mechanical energy (kinetic + potential) remains constant in the absence of non-conservative forces.
- The initial kinetic energy (Ki) is zero since the electron is released from rest.
- The initial potential energy (Ui) when the electron is at distance X from the center can be calculated using the formula for electric potential energy:
Ui=k⋅Q⋅(−e)r
where k is Coulomb's constant (9×109N m2/C2), Q is the charge of the ring, e is the charge of the electron (1.6×10−19C), and r is the distance from the charge to the electron.
- The distance r from the charge to the electron when it is at point P is given by:
r=√R2+X2=√(0.1)2+(0.25)2=√0.01+0.0625=√0.0725≈0.269m
- Thus, the initial potential energy is:
Ui=k⋅Q⋅(−e)r=9×109⋅(12×10−6)⋅(−1.6×10−19)0.269
- When the electron reaches the center of the ring, the potential energy is:
Uf=k⋅Q⋅(−e)R=9×109⋅(12×10−6)⋅(−1.6×10−19)0.1
- The final kinetic energy (Kf) when the electron reaches the center is given by:
Kf=12mv2
- By conservation of energy:
Ki+Ui=Kf+Uf
- Substituting the values:
0+Ui=12mv2+Uf
- Rearranging gives:
12mv2=Ui−Uf
- Solving for v:
v=√2(Ui−Uf)m
- Using m (mass of electron) = 9.11×10−31kg, we can calculate v.
After substituting the values and simplifying, we find that the speed of the electron when it reaches the center of the ring is approximately:
v≈15.45×106m/s
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Exercise 24.1
4 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Exercise 24.2
9 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Example Type 8
3 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions
135 Videos
Similar Questions
A uniformly charged thin ring has radius 10.0 cm and total charge +12.0nC. An electron is placed on the ring's axis a distance 25.0cm from the centre of the ring and is constrained to stay on the axis of the ring. The electron is then released from rest, then
(i) describe the subsequent motion of the electron
(ii) find the speed of the electron when it reaches the centre of the ring.
View SolutionElectric charge Q is uniformly distributed around a thin ring of radius a. find the potential a point P on the axis of the ring at a distance x from the centre of the ring .

View SolutionA ring of radius R carries a uniformly distributed charge +Q. A point charge −q is placed on the axis of the ring at a distance 2R from the centre of the ring and released from rest. The particle executes a simple harmonic motion along the axis of the ring. State True or False.
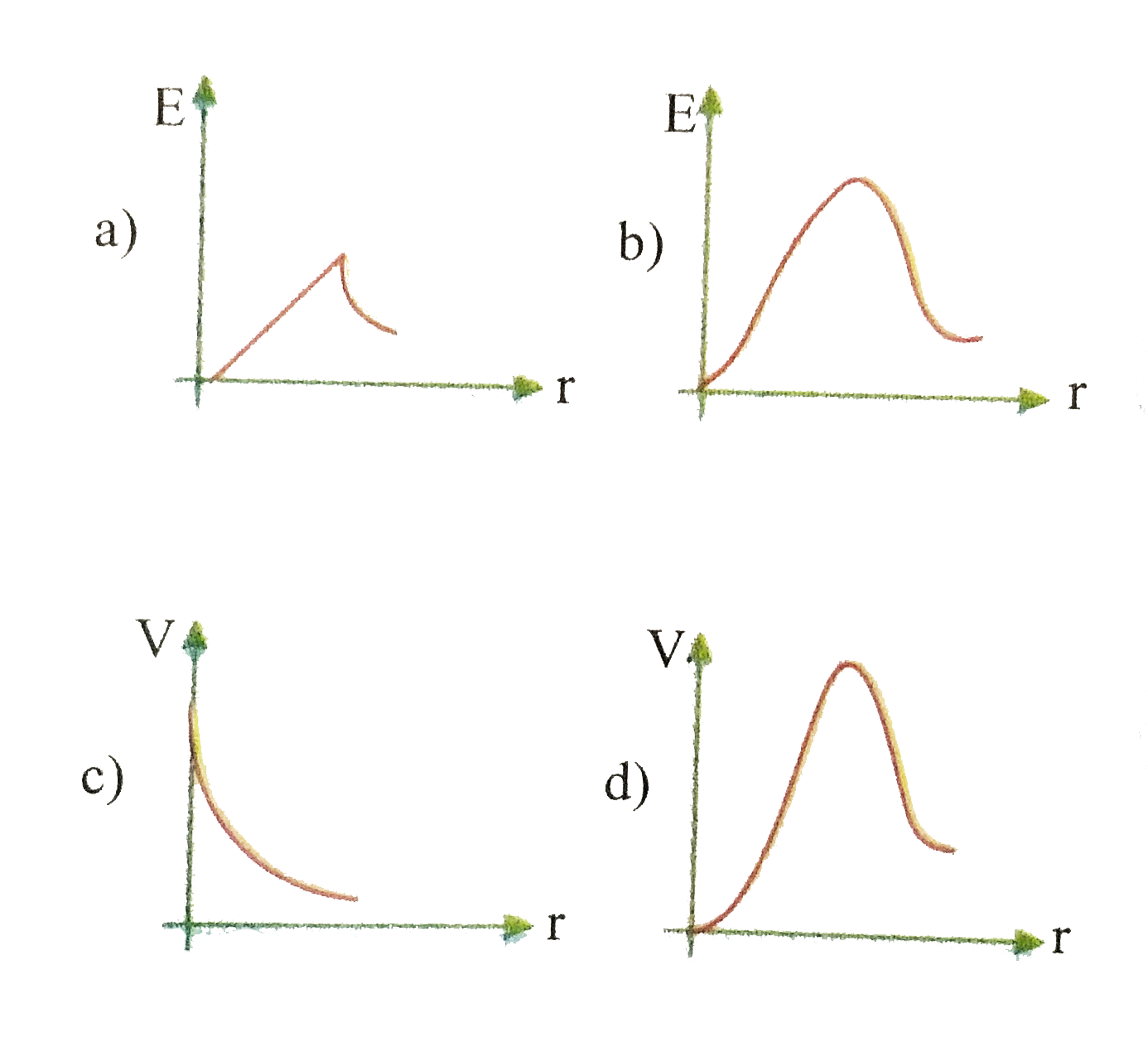
View SolutionA circular ring carries a uniformly distributed positive charge .The electric field(E) and potential (V) varies with distance (r ) from the centre of thr ring along its axis as
View SolutionAn electric dipole is kept on the axis of a uniformly charged ring at distance from the centre of the ring. The direction of the dipole moment is along the axis. The dipole moment is p, charge of the ring is Q & radius of the ring is R. The force on the dipole is
View SolutionTwo identicaln thin rings each of radius 10 cm carrying charges 10 C and 5 C are coaxially placed at a distance 10 cm apart. The work done in moving a charge q from the centre of the first ring to that of the second is
View SolutionFor a uniformly charged ring of radius R, the electric field on its exis has the largest magnitude at a distance h from its centre. Then value of h is :
View SolutionA particle of mass m is placed at a distance R from centre of a uniformly dense thin ring of mass m rand radius R on a axis passing through its centre and perpendicular to its plane. Find the speed of the particle when it reaches at the centre fo the ring due to their mutual gravitation force.
View SolutionFind the intensity of gravitational field at a point lying at a distance x from the centre on the axis of a ring of radius a and mass M.
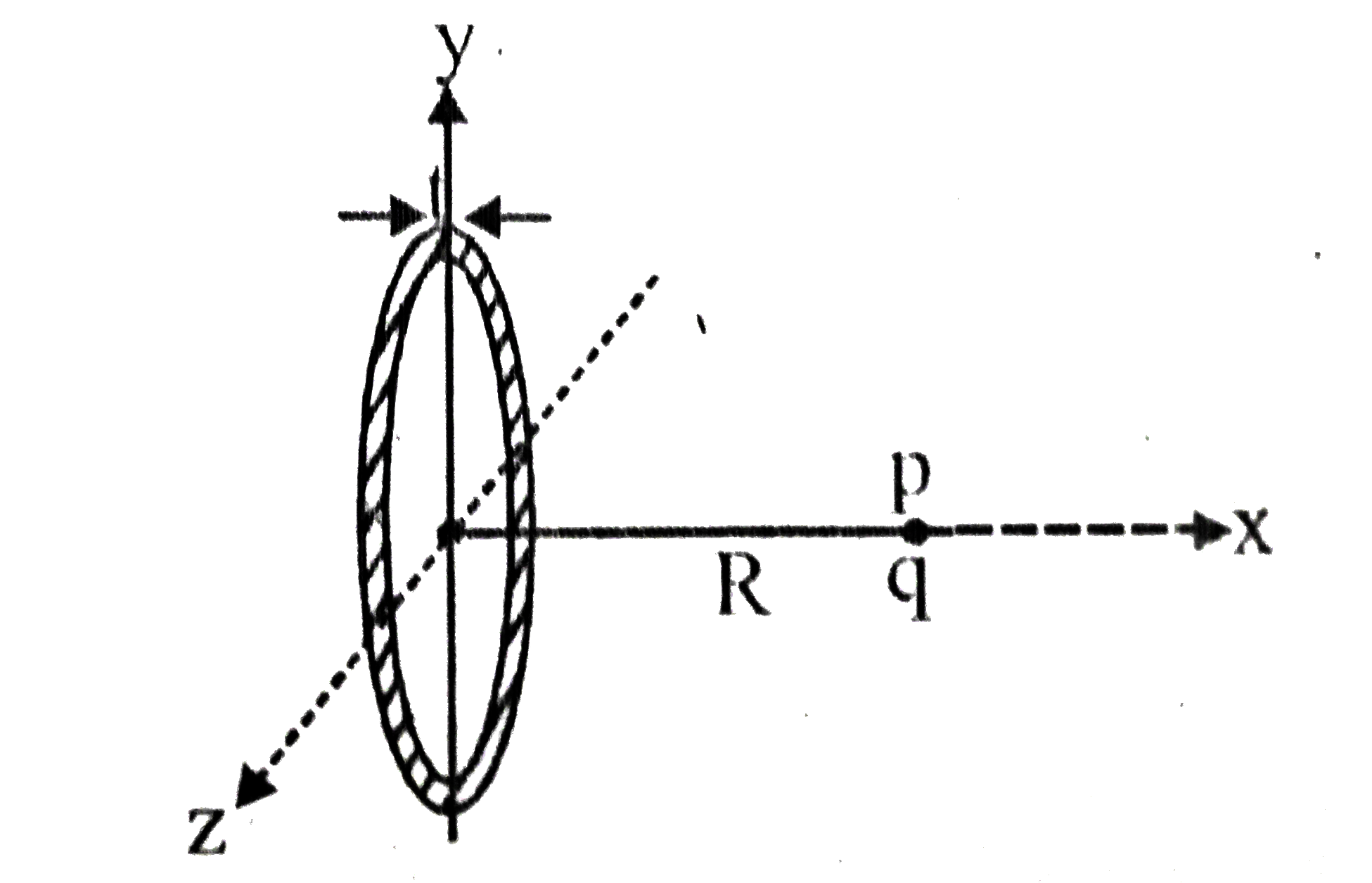
View SolutionA charge q is placed at the point P as shown in figure on the axis of the ring of elemental thickness 't' and radius R then flux through the ring is :-
View Solution
