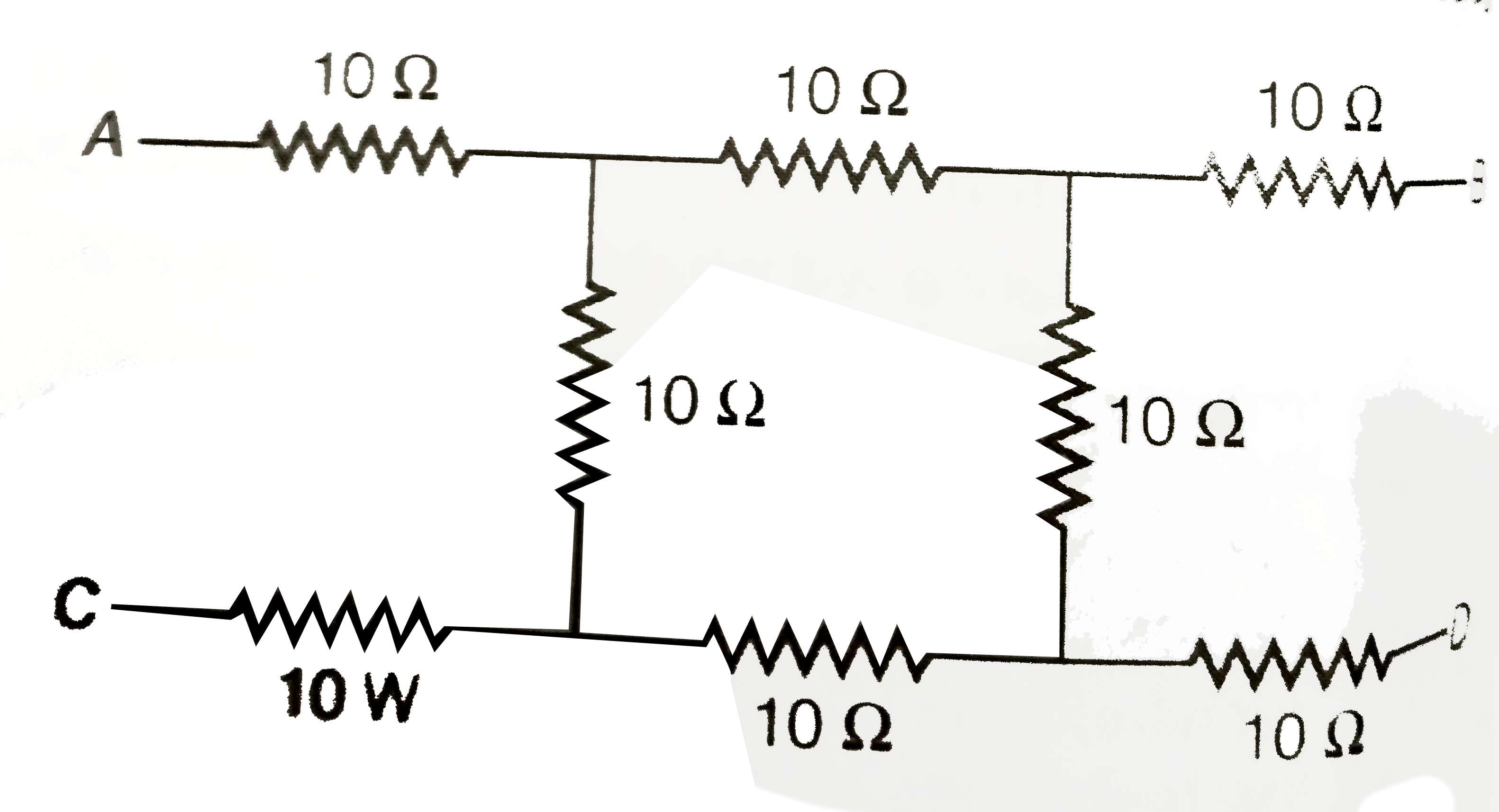
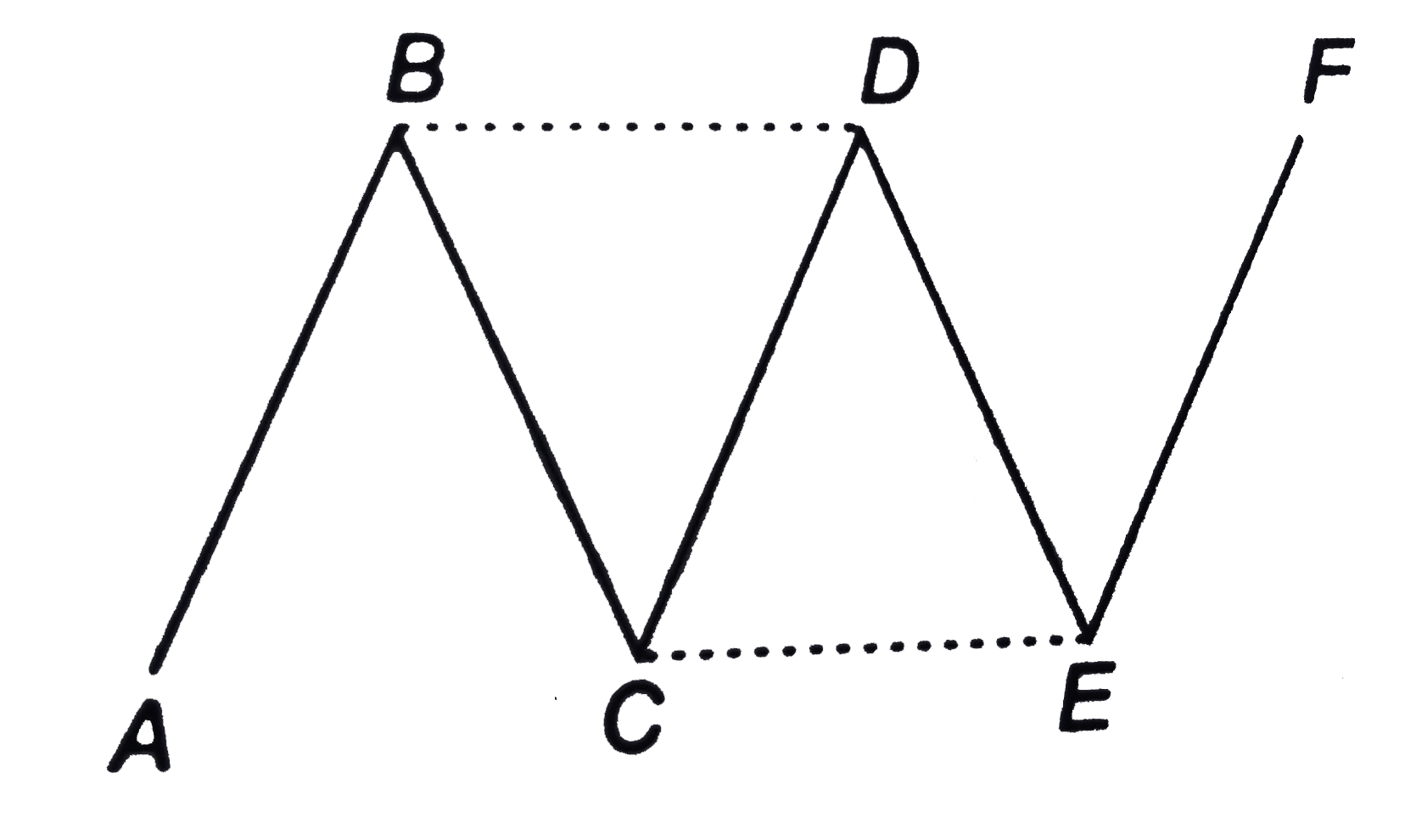
What will be the change in the resistance of a circuit between A and F consisting of five identical conductors, if two similar conductors are added as shown by the dashed line in figure?
The correct Answer is:A, B, C
Ri+5R
if connected by two equal resistors betwene B and D and between C and E the combination is a balanced wheatstone bridge and two resistors in series.
∴Rf=R+R+R=3R=0.6Ri
Topper's Solved these Questions
- CURRENT ELECTRICITYBook:DC PANDEY ENGLISHChapter:CURRENT ELECTRICITYExercise:Level 2 Single Correct
Explore 26 Videos
- CURRENT ELECTRICITYBook:DC PANDEY ENGLISHChapter:CURRENT ELECTRICITYExercise:Level 2 More Than One Correct
Explore 10 Videos
- CURRENT ELECTRICITYBook:DC PANDEY ENGLISHChapter:CURRENT ELECTRICITYExercise:OBJECTIVE_TYPE
Explore 1 Video
- COMMUNICATION SYSTEMBook:DC PANDEY ENGLISHChapter:COMMUNICATION SYSTEMExercise:Subjective
Explore 11 Videos
- ELECTROMAGNETIC INDUCTIONBook:DC PANDEY ENGLISHChapter:ELECTROMAGNETIC INDUCTIONExercise:Medical entrances gallery
Explore 25 Videos
Similar Questions
Knowledge Check
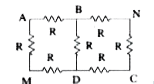
The equivalent resistance between A and B for . the circuit shown in this figure is
 A13.1ΩB15.1ΩC17.1ΩD19.1Ω
A13.1ΩB15.1ΩC17.1ΩD19.1ΩFive equal resistances of 10Ω are connected between A and B as shown in figure. The resultant resistance
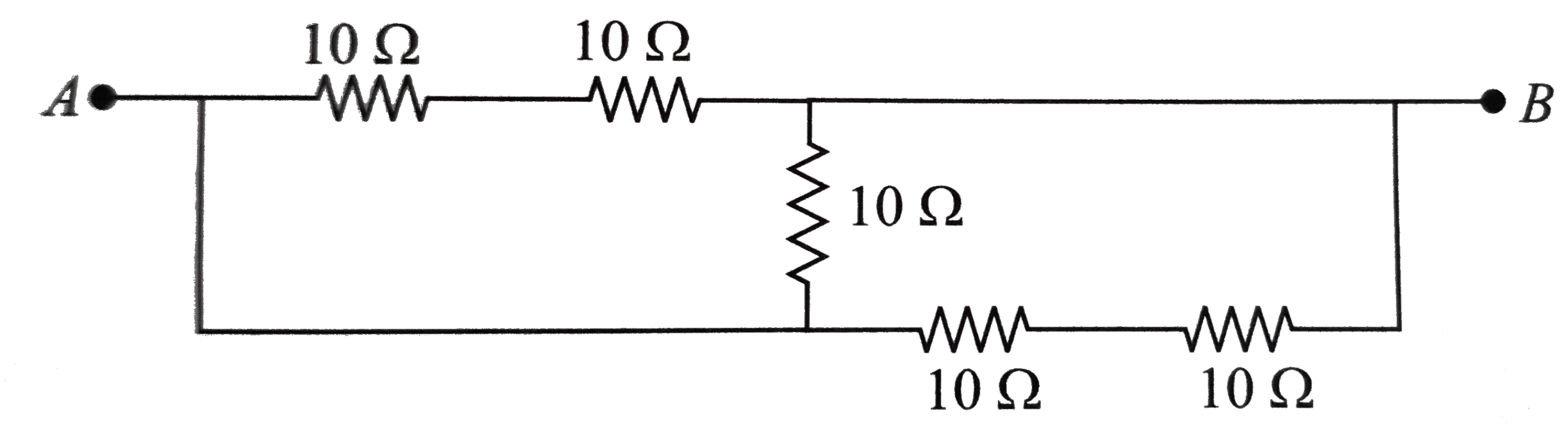 A10ΩB5ΩC15ΩD6Ω
A10ΩB5ΩC15ΩD6Ω
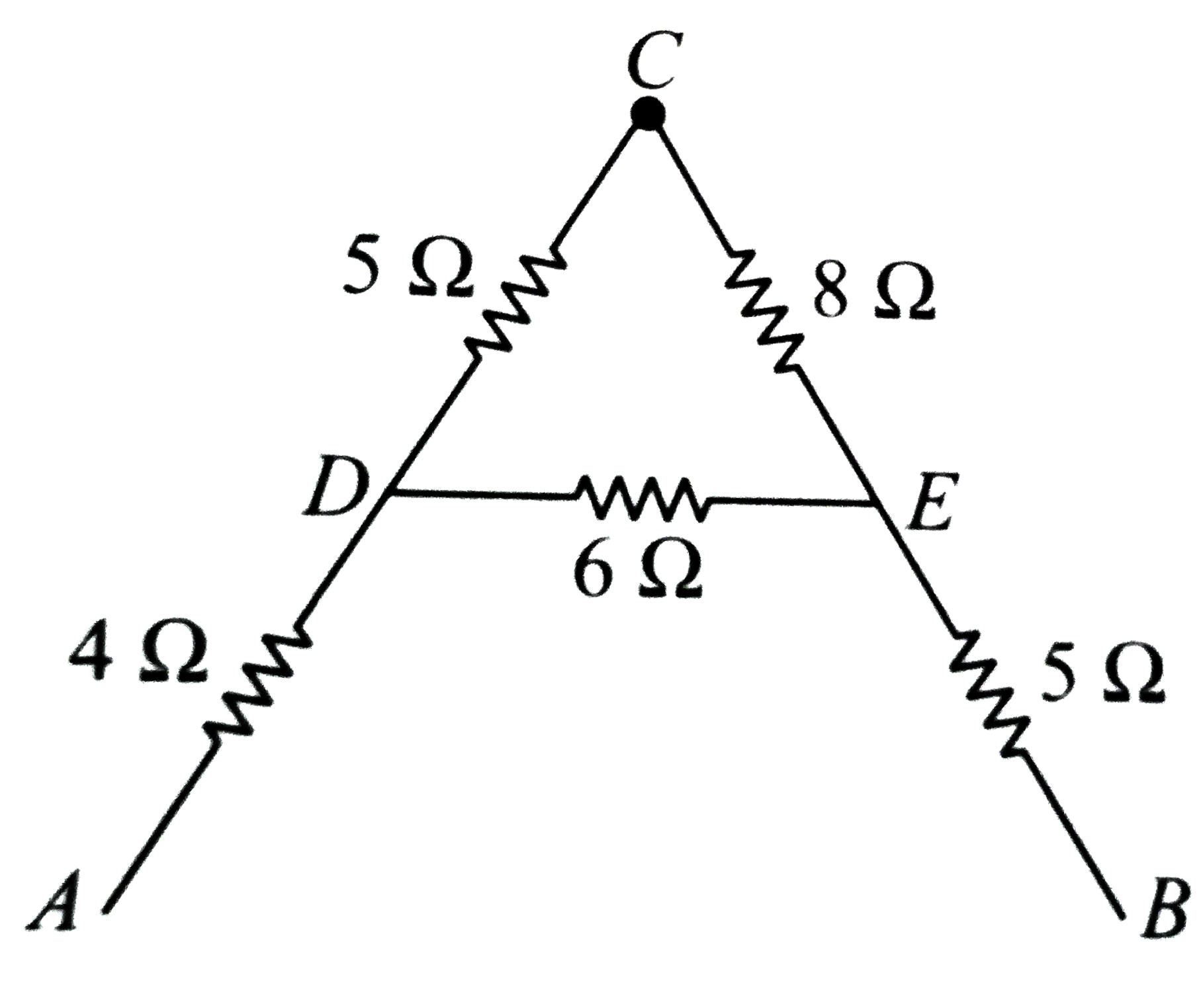
The equivalent resistance between point A and B in the circuit shown in figure is
_PHY_E05_041_Q01.png)
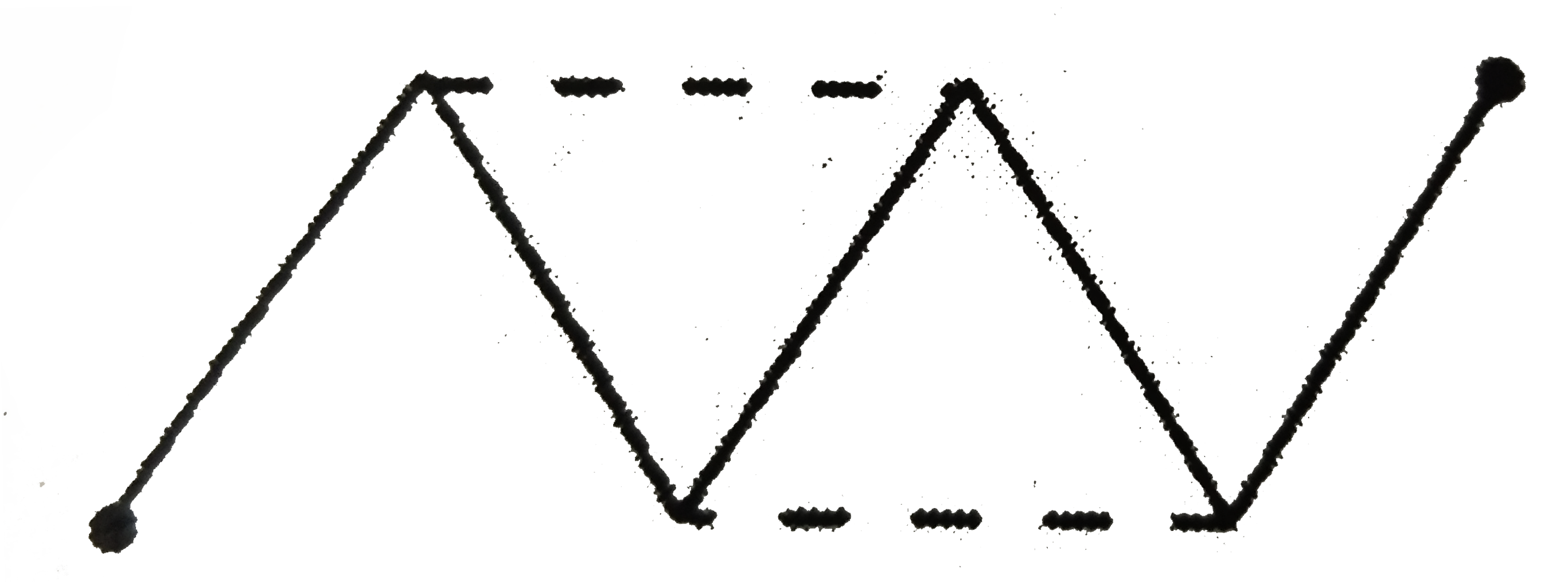
View SolutionFive identical resistors each of resistance 1Ω are initially arranged as shown in the figure by clear lines. If two resistances are added as shown by the dashed lines then change in resistance in final and initial arrangement is
View SolutionThe equivalent resistance between points A and B in the circuit shown in figure is
_PHY_E05_095_Q01.png)
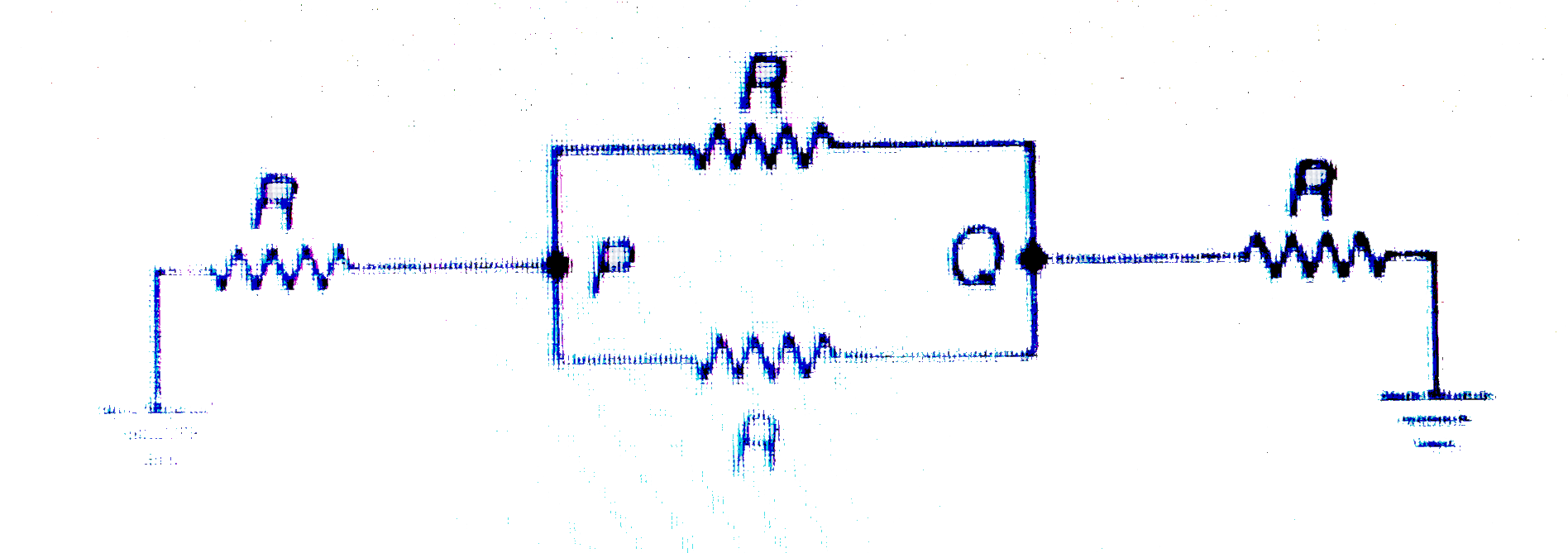
View SolutionThe net resistance between points P and Q in the circuit shown in figure is
View SolutionThe effective resistance of the infinite resistance ladder circuit between terminais A and B as shown in the figure is
View SolutionIn the circuit shown in figure, each resistance is Net resistance between points A and B is
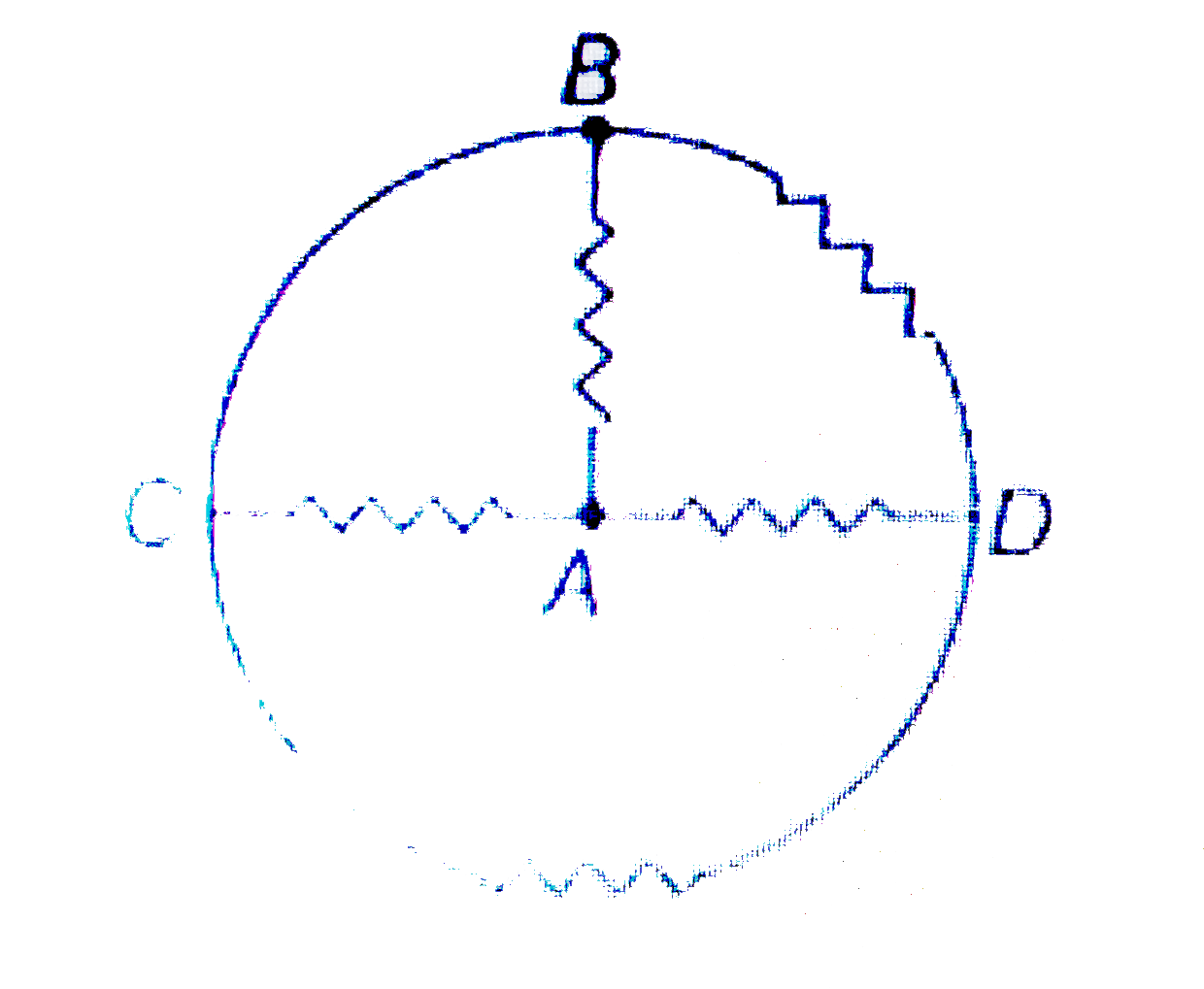
View Solution