Two identical conducting rods are first connected independently to two vessels, one containing water at and the other containing ice at . In the second case, the rods are joined end to end and connected to the same vessels. Let gram per second be the rate of melting of ice in the two cases respectively. The ratio is
(a) (b) (c) (d)
The correct Answer is:A
To solve the problem, we need to analyze the heat transfer through the conducting rods in both scenarios and determine the ratio of the rates of melting of ice, q1 and q2.
1. Understanding the Setup:
- In the first case, we have two identical conducting rods connected independently to two vessels: one at 100∘C and the other at 0∘C (ice).
- In the second case, the rods are joined end to end and connected to the same vessels.
2. Heat Transfer in the First Case:
- The heat transfer Q1 through each rod can be expressed using Fourier's law of heat conduction:
Q1=k⋅A⋅ΔTL
where:
- k is the thermal conductivity of the rods,
- A is the cross-sectional area of the rods,
- ΔT is the temperature difference (which is 100∘C−0∘C=100∘C),
- L is the length of each rod.
- Since there are two rods, the total heat transfer rate q1 will be:
q1=2⋅k⋅A⋅100L
3. Heat Transfer in the Second Case:
- In this case, the two rods are connected end to end, effectively doubling the length. The heat transfer Q2 can be expressed as:
Q2=k⋅A⋅ΔT2L
- The temperature difference remains the same at 100∘C and 0∘C, so:
q2=k⋅A⋅1002L
4. Finding the Ratio q1q2:
- Now we can find the ratio of the rates of melting of ice:
q1q2=2⋅k⋅A⋅100Lk⋅A⋅1002L
- Simplifying this gives:
q1q2=2⋅1001002=2⋅100⋅2100=4
5. Conclusion:
- Thus, the ratio q1q2 is 4, which means the correct answer is:
q1q2=4(Option C)
- In the first case, we have two identical conducting rods connected independently to two vessels: one at 100∘C and the other at 0∘C (ice).
- In the second case, the rods are joined end to end and connected to the same vessels.
- The heat transfer Q1 through each rod can be expressed using Fourier's law of heat conduction:
Q1=k⋅A⋅ΔTL
where:
- k is the thermal conductivity of the rods,
- A is the cross-sectional area of the rods,
- ΔT is the temperature difference (which is 100∘C−0∘C=100∘C),
- L is the length of each rod.
q1=2⋅k⋅A⋅100L
- In this case, the two rods are connected end to end, effectively doubling the length. The heat transfer Q2 can be expressed as:
Q2=k⋅A⋅ΔT2L
- The temperature difference remains the same at 100∘C and 0∘C, so:
q2=k⋅A⋅1002L
- Now we can find the ratio of the rates of melting of ice:
q1q2=2⋅k⋅A⋅100Lk⋅A⋅1002L
- Simplifying this gives:
q1q2=2⋅1001002=2⋅100⋅2100=4
- Thus, the ratio q1q2 is 4, which means the correct answer is:
q1q2=4(Option C)
Topper's Solved these Questions
- CALORIMETRY & HEAT TRANSFERBOOK - DC PANDEY ENGLISHCHAPTER - CALORIMETRY & HEAT TRANSFEREXERCISE - Miscellaneous Examples
13 Videos
- CALORIMETRY & HEAT TRANSFERBOOK - DC PANDEY ENGLISHCHAPTER - CALORIMETRY & HEAT TRANSFEREXERCISE - Exercise 22.1
7 Videos
- CALORIMETRY & HEAT TRANSFERBOOK - DC PANDEY ENGLISHCHAPTER - CALORIMETRY & HEAT TRANSFEREXERCISE - Example Type 3
1 Videos
- BASIC MATHEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - BASIC MATHEMATICSEXERCISE - Exercise
13 Videos
- CALORIMETRY AND HEAT TRANSFERBOOK - DC PANDEY ENGLISHCHAPTER - CALORIMETRY AND HEAT TRANSFEREXERCISE - Medical entrance s gallery
38 Videos
Similar Questions
Choose the correct option:
Two identical conducting rods are first connected independently to two vessels, one containing water at 100∘C and the other containing ice at 0∘C. In the second case, the rods are joined end to end and connected to the same vessels. Let q1andq2 gram per second be the rate of melting of ice in the two cases respectively. The ratio q1q2 is:
View SolutionTwo identical conducting rods are first connected independently to two vessels, one containing water at 100∘C and the other containing ice at 0∘C. In the second case, the rods are joined end to end connected to the same vessels. Let m1andm2 g/s be the rate of melting of ice in the two cases respectively, the ratio m1m2 is
View SolutionSix identical conducting rods are connected as shown in figure. In steady state temperature of point ϕ1 is fixed at 100∘C and temperature of ϕ6 at −80∘C
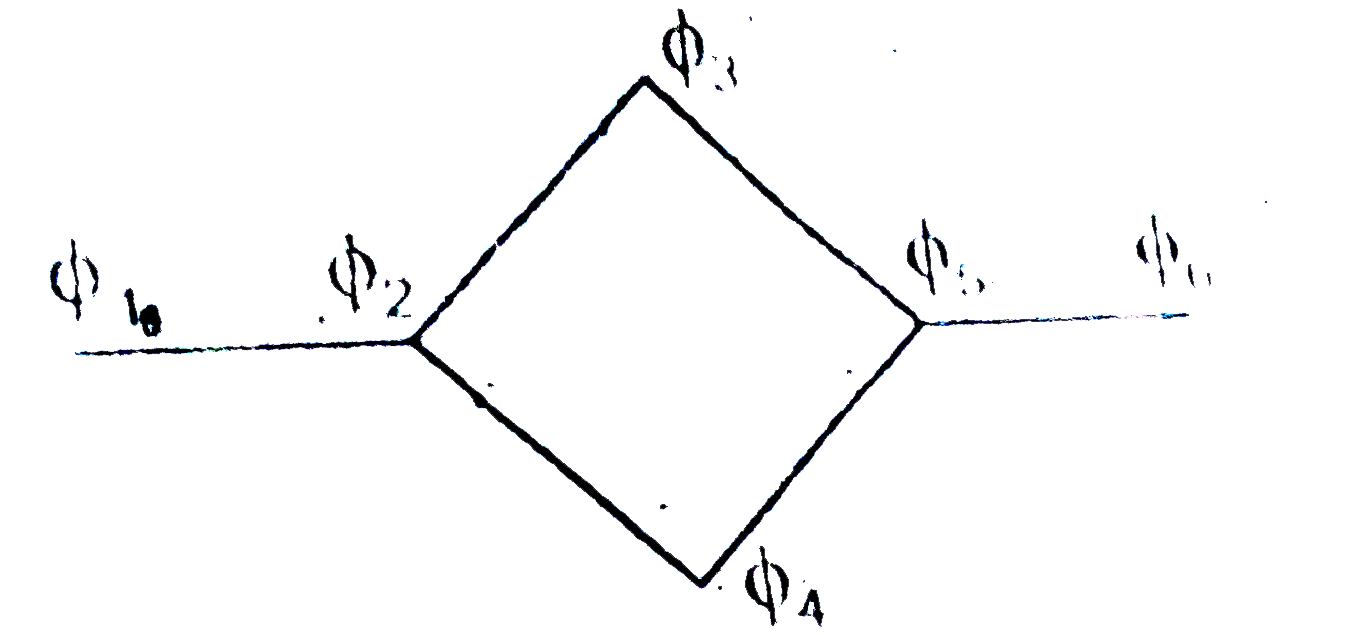
Q. What is the temperature of ϕ2?
View SolutionSix identical conducting rods are connected as shown in figure. In steady state temperature of point ϕ1 is fixed at 100∘C and temperature of ϕ6 at −80∘C
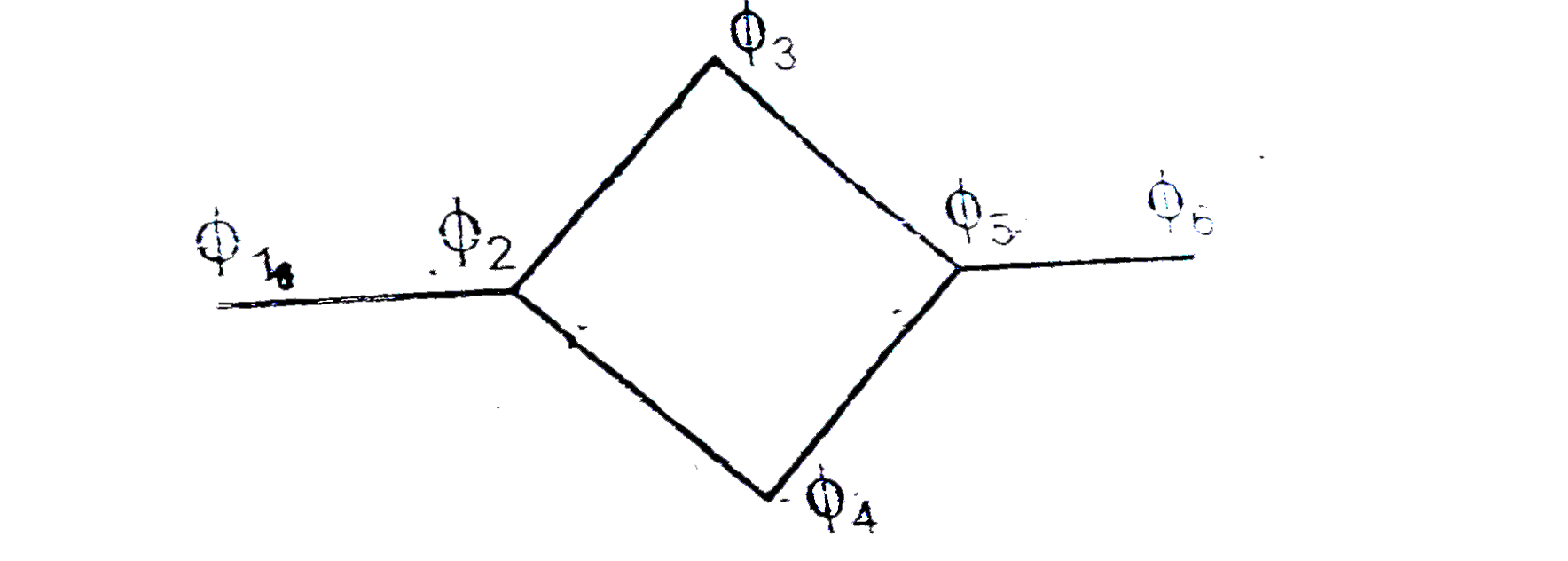
Q. What is the temperature of ϕ5?
View SolutionSix identical conducting rods are connected as shown in figure. In steady state temperature of point ϕ1 is fixed at 100∘C and temperature of ϕ6 at −80∘C

Q. What is the temperature of ϕ3?
View SolutionTwo identical rods are connected between two containers. One of them is at 100∘C containing water and another is at 0∘C containing ice. If rods are connected in parallel then the rate of melting of ice is q1g/s . If they are connected in series then teh rate is q2g/s . The ratio q2/q1 is
View SolutionTwo conducting rods when connected between two points at constant but different temperatures separately, the rate of heat flow through them is q1andq2.
View SolutionOne end of a brass rod of length 2.0 m and cross section 1cm2 is kept in steam at 100∘C and the other end in ice at 0∘C. The lateral surface of the rod is covered by heat insulator. Determine the amount of ice melting per minute. Thermal conductivity of brass is 110W/m−K and specific latent heat of fusion of ice is 80cal/g.
View SolutionTwo chemically non-reactive gases are separately contained in two vessels of same capacity at pressure P1, and P2, respectively. If two vessels are connected, the total pressure of gaseous mixture will be
View SolutionTwo chemically non-reactive gases are separately contained in two vessels of same capacity at pressure P1 and P2 respectively. If two vessels are connected, the total pressure of gaseous mixture will
View Solution
