An ideal gas goes through the cycle abc. For the complete cycle 800J of heat flows out of the gas. Process ab is at constant pressure and process bc is at constant volume. In process c-a, p∝V. States a and b have temperature Ta=200K and Tb=300K. (a) Sketch the p-V diagram for the cycle. (b) What is the work done by the gas for the process ca?
The correct Answer is:B, D
(a)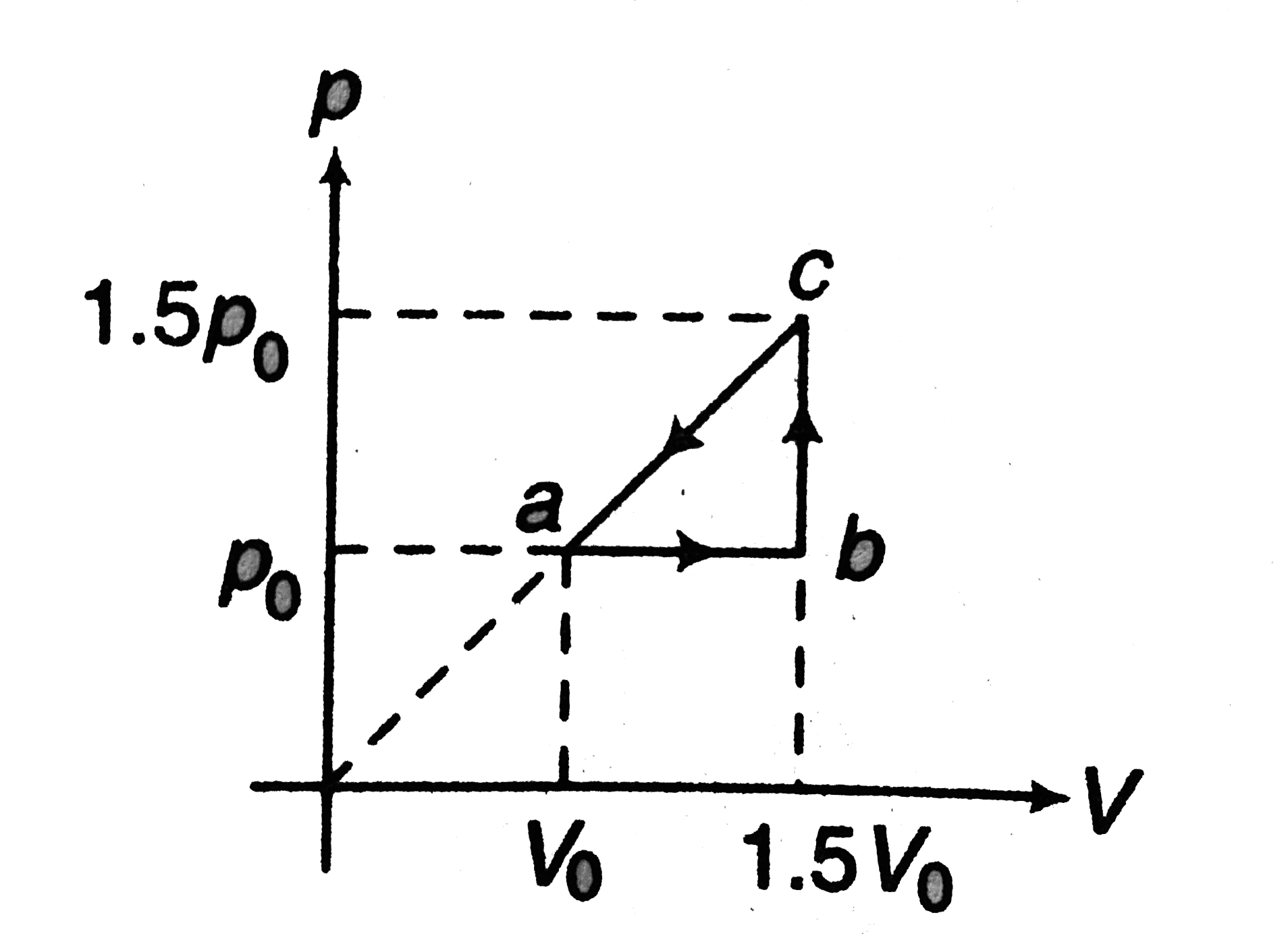 
Wnet=Qnet in a cycle.
Since, Qnet is negative (flows out of the gas). Hence, Wnet should aslo be negative. Or, cycle should be anti-clockwise as shown in figure.
From a to b
p=constant
∴ V∝T
T has become 1.5 times. Therefore, V will also become 1.5 times.
From c to a
p∝V
V has become 11.5 times. Therefore,
p will become 11.5 times.
In a cycle
|Qnet|=|Wnet|=Area of cycle
∴ 800=12(0.5p0)(0.5V0)
∴ p0V0=6400J
Wca=−Areaunderthegraph<br>=-1/2(2.5p_0)(0.5V_0)<br>=-0.625p_0V_0<br>=-0.625xx6400=-4000J
Topper's Solved these Questions
- LAWS OF THERMODYNAMICSBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 Passage II
3 Videos
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Medical entrances gallery
39 Videos
- MAGNETIC EFFECT OF CURRENT AND MAGNETISMBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC EFFECT OF CURRENT AND MAGNETISMEXERCISE - Integer type Questions
10 Videos
Similar Questions
An ideal gas change from state a to state b as shown in Fig. what is the work done by the gas in the process?
View SolutionIn figure, a sample of an ideal gas is taken through the cyclic process abca. 800J of work is done by the gas during process ab. If gas absorb no heat in process ab, rejects 100J of heat during bc and absord 500J of heat during process ca. Then (a) find the internal energy of the gas at b and c if its is 1000J at a (b). Also calculate the work by the gas during the part bc,
View Solution
Knowledge Check
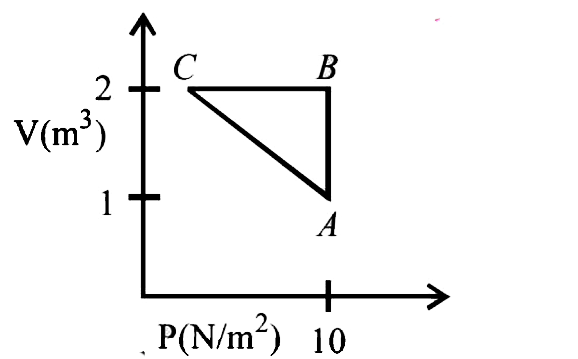
An ideal gas is taken through cycle A→B→C−A, as shown in Fig. IF the net heat supplied to the gas in the cycle is 5 J, the work done by the gas in the process C→A is
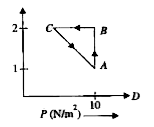A−5JB−10JC−15JD−20J
A sample of ideal gas is taken through the cyclic process shown in the figure. The temerauture of the gas in state A is TA =200 K. In states B and C the temperature of the gas is the same.
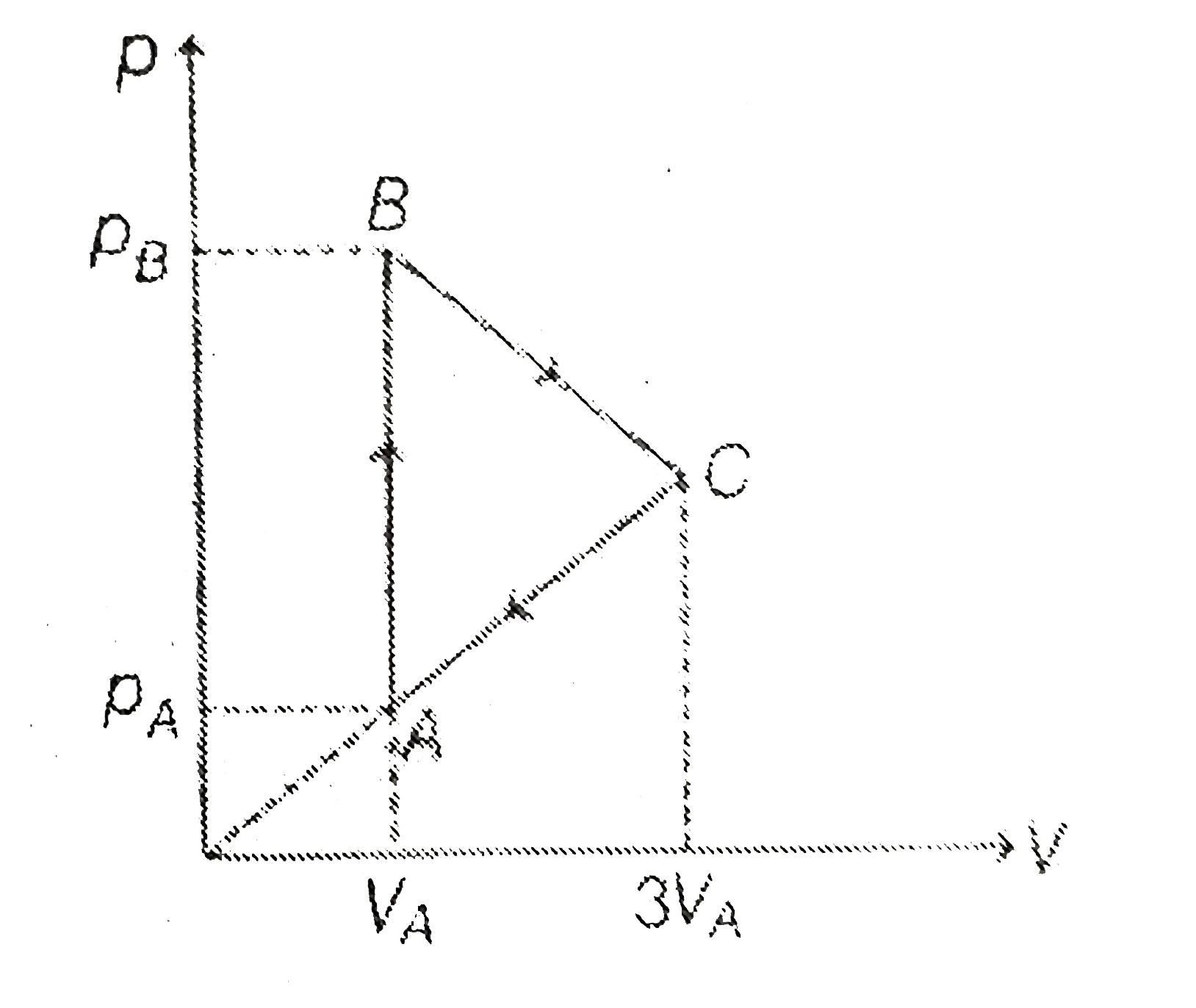
Net work done by the gas in the process is
View SolutionAn ideal gas is taken through the cycle A→B→C→A, as shown in the figure, If the net heat supplied to the gas in the cycle is 5J, the work done by the gas in the process CtoA is
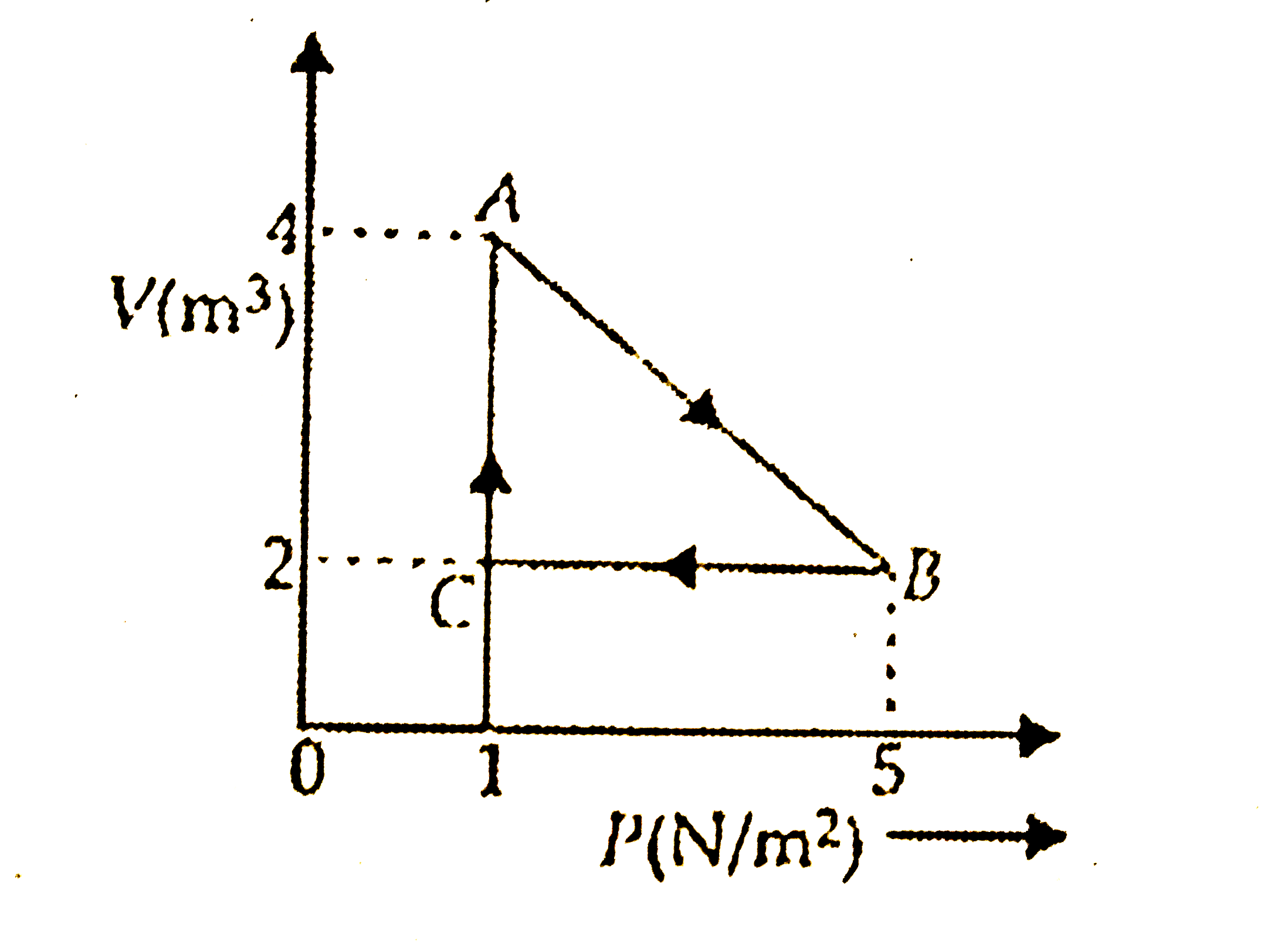
View SolutionThree moles of an ideal gas (Cp=72R) at pressure, PA and temperature TA is isothermally expanded to twice its initial volume. It is then compressed at constant pressure to its original volume. Finally gas is compressed at constant volume to its original pressure PA.
(a) Sketch P-V and P-T diagrams for the complete process.
(b) Calculate the net work done by the gas, and net heat supplied to the gas during the complete process.
View SolutionThree moles of an ideal gas at a pressure PA and temperature TA is isothermally expanded to twice its initial volume. It is then compressed at constant pressure to its original , volume. Finally the gas is compressed at constant volume to its original pressure PA
(a) Sketch the P-V and P-T diagrams for the complete process.
(b) Calculate the net work done by the gas, and net heat supplied to the gas during the complete process.
View SolutionAn ideal gas is taken through the cycle A→B→C→A, as shown in figure. If the net heat supplied to the gas in the cycle is 5 J the work done by the gas in the process A→B is
View SolutionThree processes compose a thermodynamic cycle shown in the accompanying P−V, diagram of an ideal gas.
Process 1→2 take place at constant temperature, during this process 60J of heat enters the system.
Process 2→3 takes place at constant volume. During this process 40J of heat leaves the system.
Process 3→1 is adiabatic.
What is the change in internal energy of the during process 3→1?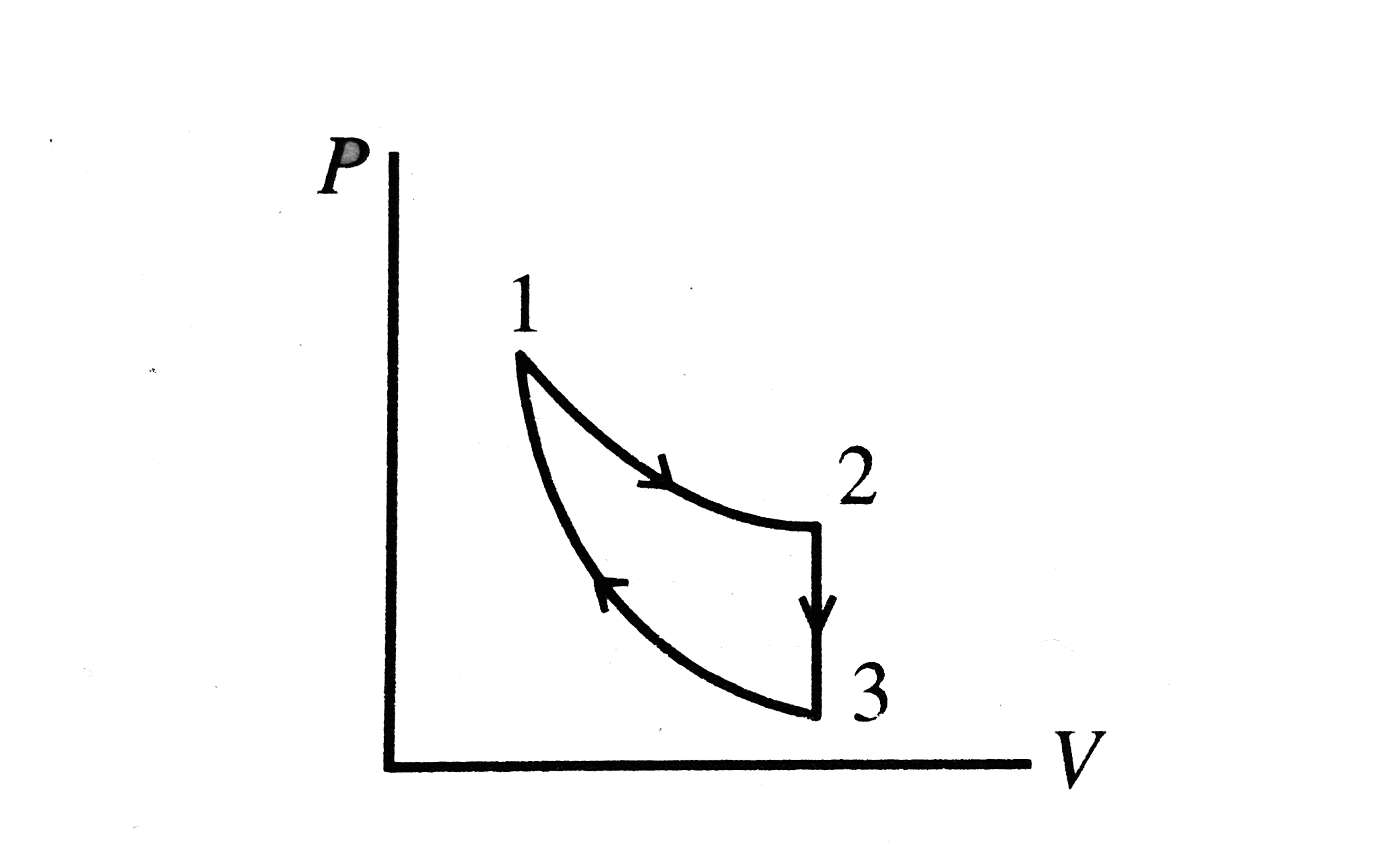
View SolutionTemperature of two moles of an ideal gas is increased by 300K in a process V=aT, where a is positive constant. Find work done by the gas in the given process.
View Solution