Two moles of a diatomic ideal gas is taken through pT= constant. Its temperature is increased from T to 2T. Find the work done by the system?
The correct Answer is:D
To solve the problem, we need to find the work done by the system when 2 moles of a diatomic ideal gas undergo a process at constant P⋅T while the temperature is increased from T to 2T.
1. Understanding the Process:
The process is defined as P⋅T=constant. We can denote this constant as K.
P⋅T=K
2. Using the Ideal Gas Law:
The ideal gas equation is given by:
PV=nRT
From the relation P=nRTV, we can substitute P in the equation P⋅T=K:
nRTV⋅T=K
This simplifies to:
nRT2V=K
3. Rearranging for Volume:
Rearranging gives us:
V=nRT2K
4. Differentiating Volume with Respect to Temperature:
To find the change in volume with respect to temperature, we differentiate:
V=nRKT2
Differentiating both sides with respect to T:
dV=nRK⋅2TdT
Thus,
dV=2nRKTdT
5. Finding Work Done:
The work done W by the gas is given by the integral of pressure with respect to volume:
W=∫PdV
Substituting P from the relation P=KT:
W=∫KTdV
Now substituting dV:
W=∫KT⋅2nRKTdT
Simplifying gives:
W=∫2nRdT
6. Evaluating the Integral:
The limits of integration are from T to 2T:
W=2nR∫2TTdT=2nR(T)2TT=2nR(2T−T)=2nRT
7. Substituting the Number of Moles:
Since we have 2 moles of gas:
W=2⋅2RT=4RT
Final Answer:
The work done by the system is:
W=4RT
The process is defined as P⋅T=constant. We can denote this constant as K.
P⋅T=K
The ideal gas equation is given by:
PV=nRT
From the relation P=nRTV, we can substitute P in the equation P⋅T=K:
nRTV⋅T=K
This simplifies to:
nRT2V=K
Rearranging gives us:
V=nRT2K
To find the change in volume with respect to temperature, we differentiate:
V=nRKT2
Differentiating both sides with respect to T:
dV=nRK⋅2TdT
Thus,
dV=2nRKTdT
The work done W by the gas is given by the integral of pressure with respect to volume:
W=∫PdV
Substituting P from the relation P=KT:
W=∫KTdV
Now substituting dV:
W=∫KT⋅2nRKTdT
Simplifying gives:
W=∫2nRdT
The limits of integration are from T to 2T:
W=2nR∫2TTdT=2nR(T)2TT=2nR(2T−T)=2nRT
Since we have 2 moles of gas:
W=2⋅2RT=4RT
The work done by the system is:
W=4RT
Topper's Solved these Questions
- LAWS OF THERMODYNAMICSBook:DC PANDEY ENGLISHChapter:LAWS OF THERMODYNAMICSExercise:Exercise 21.1
Explore 1 Video
- LAWS OF THERMODYNAMICSBook:DC PANDEY ENGLISHChapter:LAWS OF THERMODYNAMICSExercise:Exercise 21.2
Explore 7 Videos
- LAWS OF THERMODYNAMICSBook:DC PANDEY ENGLISHChapter:LAWS OF THERMODYNAMICSExercise:Example Type 3
Explore 1 Video
- LAWS OF MOTIONBook:DC PANDEY ENGLISHChapter:LAWS OF MOTIONExercise:Medical entrances gallery
Explore 39 Videos
- MAGNETIC EFFECT OF CURRENT AND MAGNETISMBook:DC PANDEY ENGLISHChapter:MAGNETIC EFFECT OF CURRENT AND MAGNETISMExercise:Integer type Questions
Explore 10 Videos
Similar Questions
In a thermodynamic process two moles of a monatomic ideal gas obeys PV–2=constant. If temperature of the gas increases from 300 K to 400 K, then find work done by the gas (Where R = universal gas constant)
View SolutionTwo moles of an ideal monatomic gas is heated at constant pressure so that its temperature increases from 127∘C to 227∘C , then work done by gas
View SolutionTwo moles of an ideal diatomic gas is taken through a process VT2= constant so that its temperature increases by ΔT=300K. The ratio (ΔUΔQ) of increase in internal energy and heat supplied to the gas during the process is
View SolutionOne mole of an ideal gas at temperature T expands slowly according to the law pV= constant.
Its final temperature is T2. The work done by the gas is
View SolutionOne mole of an ideal gas at temperature T expands slowly according to the law pV= constant.
Its final temperature is T2. The work done by the gas is
View SolutionOne mole of an ideal gas at temperature T expands slowly according to the law pV= constant.
Its final temperature is T2. The work done by the gas is
View SolutionOne mole of an ideal diatomic gas is taken through a process whose P−V diagram is shown in the figure. The work done by the gas is
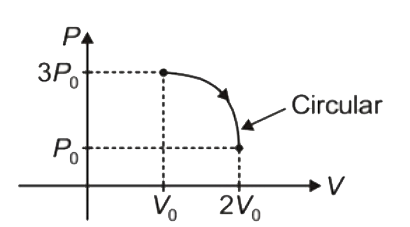
View SolutionOne mole of an ideal monoatomic gas is taken through a polytropic process P2T = constant. The heat required to increase the temperature of the gas by ΔT is
View Solutionn moles of an ideal gas undergo a process in which the temperature changes with volume as T=kv2. The work done by the gas as the temperature changes from T0 to 4T0 is
View SolutionTwo moles of an ideal monoatomic gas is taken through a cycle ABCA as shown in the p - T diagram. During the process AB, the pressure and temperature of the gas vary such that pT = constant. If T1=300K and the amount of heat released by the gas during the process AB is Q=−xR, then the value of x is
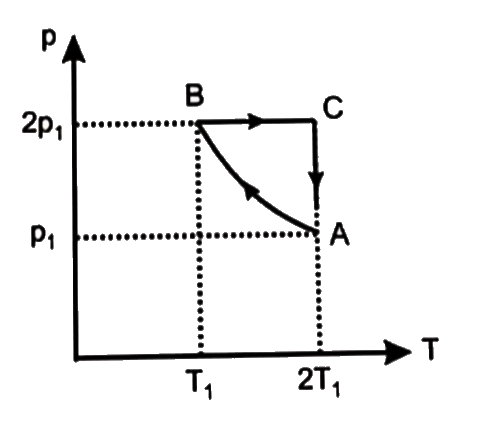
View Solution