A pump is designed as a horizontal cylinder with a piston of area A and and outlet orifice of area a arranged near the cylinder axis. Find the velocity of out flow of the liquid from the pump if the piston moves with a constant velocity under the action of a constant force F. The density of the liquid is ρ.
The correct Answer is:A, B
To find the velocity of outflow of the liquid from the pump, we can follow these steps:
Step 1: Define the pressures at the two sections
- Let P1 be the pressure just behind the piston (Section 1) and P2 be the pressure at the outlet orifice (Section 2).
- The pressure at Section 1 can be expressed as:
P1=Pa+FA
where Pa is the atmospheric pressure, F is the constant force applied, and A is the area of the piston.
- The pressure at Section 2 is simply the atmospheric pressure:
P2=Pa
Step 2: Apply the continuity equation
- According to the continuity equation, the mass flow rate at Section 1 must equal the mass flow rate at Section 2:
˙m1=˙m2
- This can be expressed as:
ρAv1=ρav2
where v1 is the velocity of the piston and v2 is the velocity of the outflow.
- We can simplify this to:
Av1=av2
- Rearranging gives:
v1=aAv2
Step 3: Apply Bernoulli's equation
- According to Bernoulli's theorem, we can write:
P1ρg+v212g=P2ρg+v222g
- Substituting for P1 and P2:
Pa+FAρg+v212g=Paρg+v222g
- This simplifies to:
FAρg+v212g=v222g
Step 4: Substitute v1 into Bernoulli's equation
- Substitute v1=aAv2 into the equation:
FAρg+12g(aAv2)2=v222g
- This leads to:
FAρg+a22A2gv22=v222g
Step 5: Rearranging the equation
- Rearranging gives:
FAρg=v222g−a22A2gv22
- Factoring out v22:
FAρg=v222g(1−a2A2)
Step 6: Solve for v22
- Multiply both sides by 2g(1−a2A2):
v22=2FρA⋅1(1−a2A2)
Step 7: Take the square root to find v2
- Taking the square root gives:
v2=
⎷2FρA⋅1(1−a2A2)
Step 8: Special case when a is negligible
- If a is very small compared to A, we can approximate:
v2≈√2FρA
Final Answer
Thus, the velocity of outflow of the liquid from the pump is:
v2=
⎷2FρA⋅1(1−a2A2)
or approximately:
v_2 \approx \sqrt{\frac{2F}{\rho A}} \quad \text{(if a is negligible)}
- Let P1 be the pressure just behind the piston (Section 1) and P2 be the pressure at the outlet orifice (Section 2).
- The pressure at Section 1 can be expressed as:
P1=Pa+FA
where Pa is the atmospheric pressure, F is the constant force applied, and A is the area of the piston.
P2=Pa
- According to the continuity equation, the mass flow rate at Section 1 must equal the mass flow rate at Section 2:
˙m1=˙m2
- This can be expressed as:
ρAv1=ρav2
where v1 is the velocity of the piston and v2 is the velocity of the outflow.
- We can simplify this to:
Av1=av2
- Rearranging gives:
v1=aAv2
- According to Bernoulli's theorem, we can write:
P1ρg+v212g=P2ρg+v222g
- Substituting for P1 and P2:
Pa+FAρg+v212g=Paρg+v222g
- This simplifies to:
FAρg+v212g=v222g
- Substitute v1=aAv2 into the equation:
FAρg+12g(aAv2)2=v222g
- This leads to:
FAρg+a22A2gv22=v222g
- Rearranging gives:
FAρg=v222g−a22A2gv22
- Factoring out v22:
FAρg=v222g(1−a2A2)
- Multiply both sides by 2g(1−a2A2):
v22=2FρA⋅1(1−a2A2)
- Taking the square root gives:
v2=
⎷2FρA⋅1(1−a2A2)
- If a is very small compared to A, we can approximate:
v2≈√2FρA
Thus, the velocity of outflow of the liquid from the pump is:
v2=
⎷2FρA⋅1(1−a2A2)
or approximately:
v_2 \approx \sqrt{\frac{2F}{\rho A}} \quad \text{(if a is negligible)}
Topper's Solved these Questions
- FLUID MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Subjective Question
2 Videos
- FLUID MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Level 2 Single Correct
36 Videos
- FLUID MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Level 1 Single Correct
49 Videos
- EXPERIMENTSBOOK - DC PANDEY ENGLISHCHAPTER - EXPERIMENTSEXERCISE - Subjective
15 Videos
- GENERAL PHYSICSBOOK - DC PANDEY ENGLISHCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE
2 Videos
Similar Questions
A metal plate of area 103 cm2 rests on a layer o oil 6 mm thick. A tangential force of 10−2 N is appled on it to move it with a constant velocity of 6 cm s−1 . The coefficient of viscosity of the liquid is :-
View SolutionA vertical cylinder of cross-section area A contains one mole of an ideal mono-atomic gas under a piston of mass M At a certain instant a heater which supplies heat at the rate q J/s is switched ON under the piston. The velocity with which the piston moves upward under the condition that pressure of gas remains constant is [Assume on heat transfer through walls of cylinder]
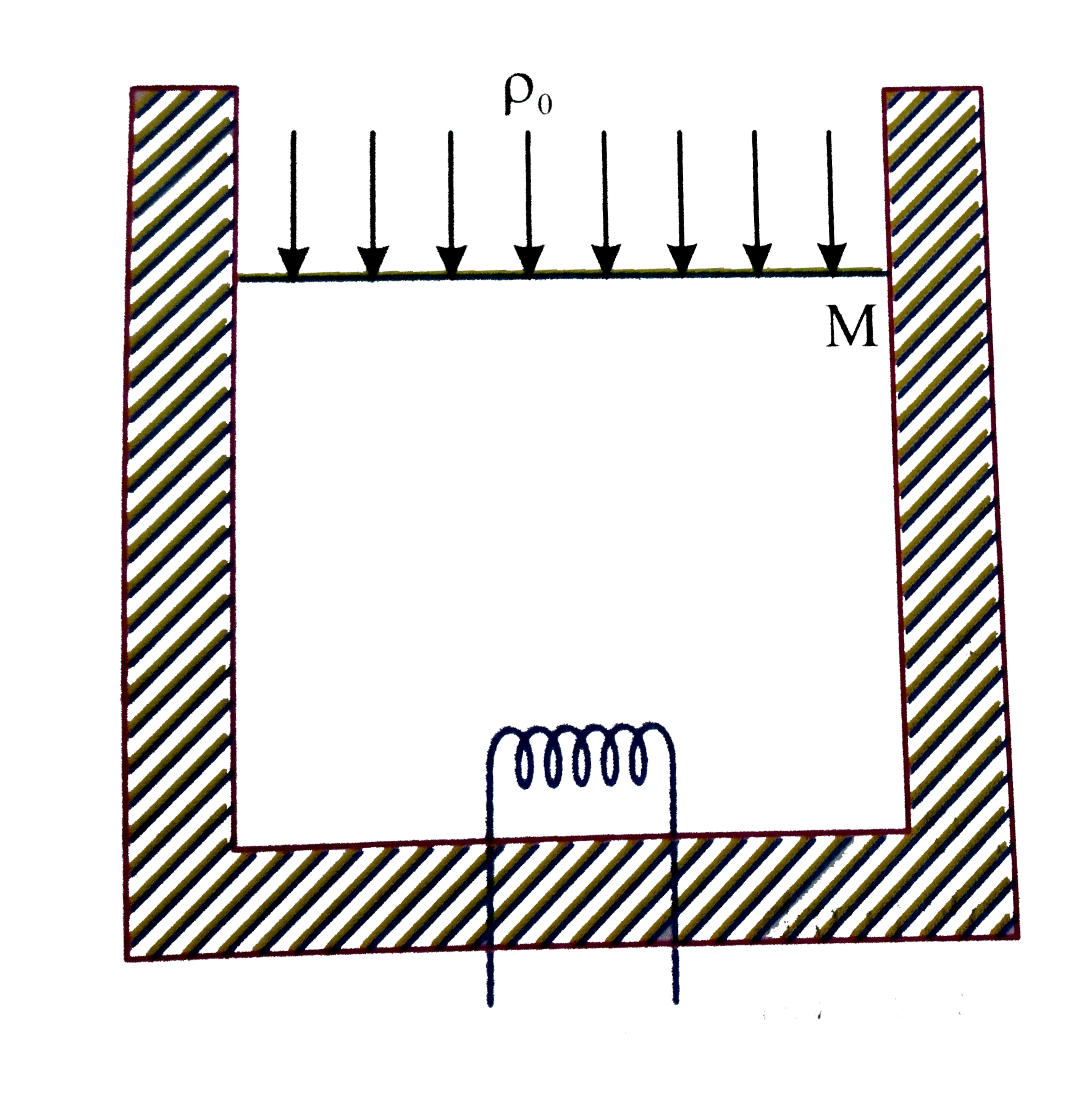
View Solution
Knowledge Check
A cylinider is filled with non viscous liquid of density d to a height h0 and a hole is made at a height h1 from the bottom of the cylinder. The velocity issuing out of the hole is
A√2gh0B√2g(h0−h1)C√dgh1D√dgh0
_E01_229_Q01.png)
A cylinderical tank having cross sectional area ∧=0.5m2 is filled liquids of densities ρ1=900kgm3&ρ2=600kgm3 to a height h=60cm as shown in the figure a small hole having area a=5cm2 is made in right vertical wall at a height y=20cm from the bottom calculate.
(i). velocity of efflux
(ii). horizontal force F to keep the cylinder in static equilibrium if it is placed on a smooth horizontal plane
(iii). minimum and maximum value of F to keep the cylinder at rest. The coefficient of friction between cylinder and the plane is μ=0.1
(iv). velocity of the top most layer of the liquid column and also the velocity of the boundary separating the two liquids.
View SolutionAflat plate of area 0.05m2 is separated from another large plate at rost by a liquid layer of uniform thickness 1μm. The tangential force needed to move the smaller plate with a constant velocity of 10cms−1 i s 20 N. Calculate the coefficent of viscosity of the liquid.
View SolutionFluids at rest exert a normal force to the walls of the container or to the sruface of the Body immersed in the fluid. The pressure exerted by this force at a point inside the liqid is the sum of atmospheric pressure and a factor which depends on the density of the liquid, the acceleration due to gravity and the height of the liquid, above that point. The upthrust acting on a body immersed in a stationary liquid is the net force acting on the body in the upward direction. A number of phenomenon of liquids in motion can be explain by Bernoulli's theorem which relates the pressure, flow speed and height for flow of an ideal incompressible fluid.
A container of large uniform corss sectional area. A resting on a horizontal surface holds two immiscible, non viscous and incompressile liquids of densities d and 2d, each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0.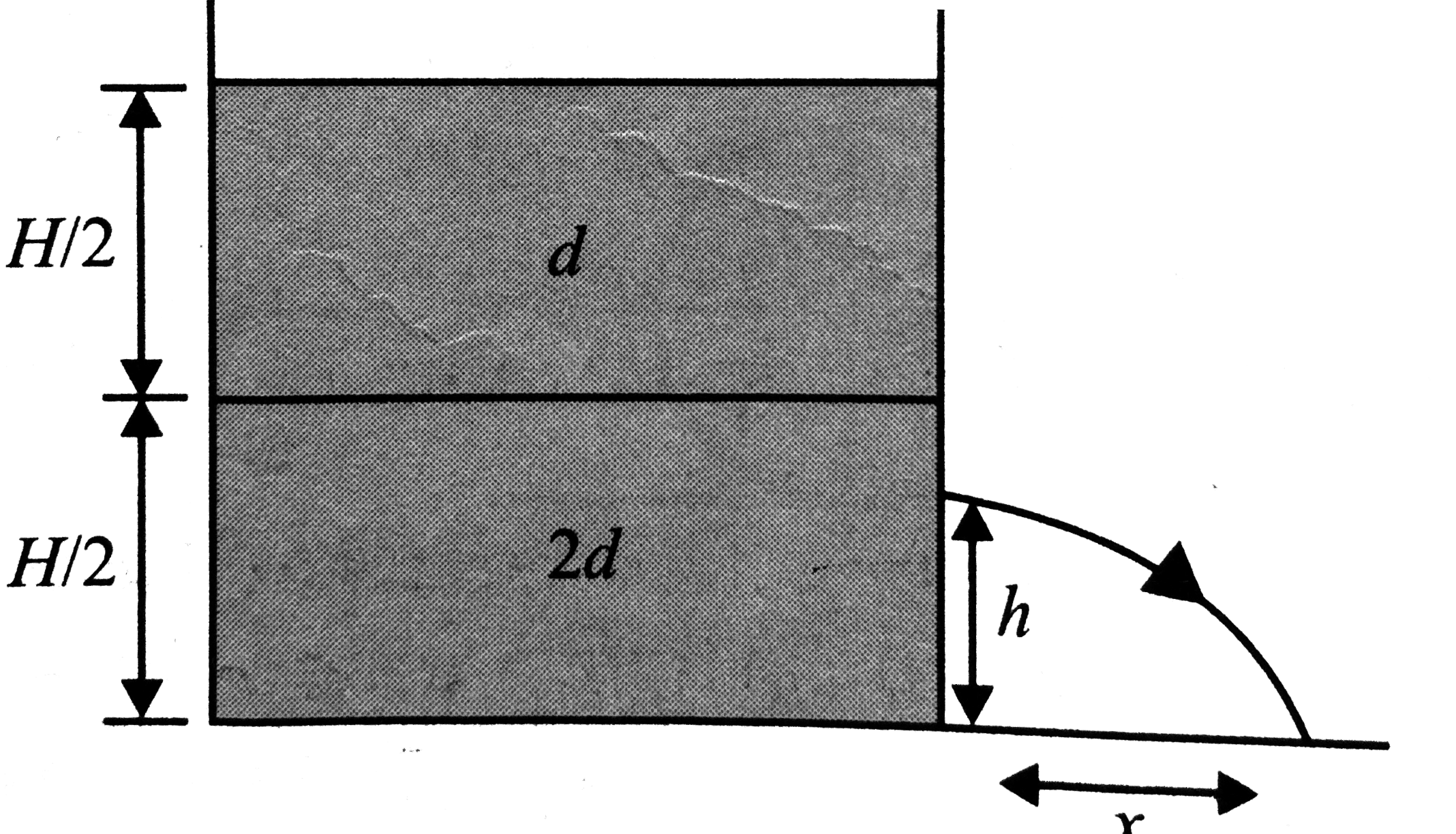
Situation I:
A homogeneous solid cylinder of length L(L<H/2). cross sectional area A/5 is immersed such that it floats with its axis vertical at liquid -liquid interface with lenght L/4 in the denser liquid.
The density of the solid is
View SolutionFluids at rest exert a normal force to the walls of the container or to the sruface of the body immersed in the fluid. The pressure exerted by this force at a point inside the liqid is the sum of atmospheric pressure and a factor which depends on the density of the liquid, the acceleration due to gravity and the height of the liquid, above that point. The upthrust acting on a body immersed in a stationary liquid is the net force acting on the body in the upward direction. A number of phenomenon of liquids in motion can be explain by Bernoulli's theorem which relates the pressure, flow speed and height for flow of an ideal incompressible fluid.
A container of large uniform corss sectional area. A resting on a horizontal surface holds two immiscible, non viscous and incompressile liquids of densities d and 2d, each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0.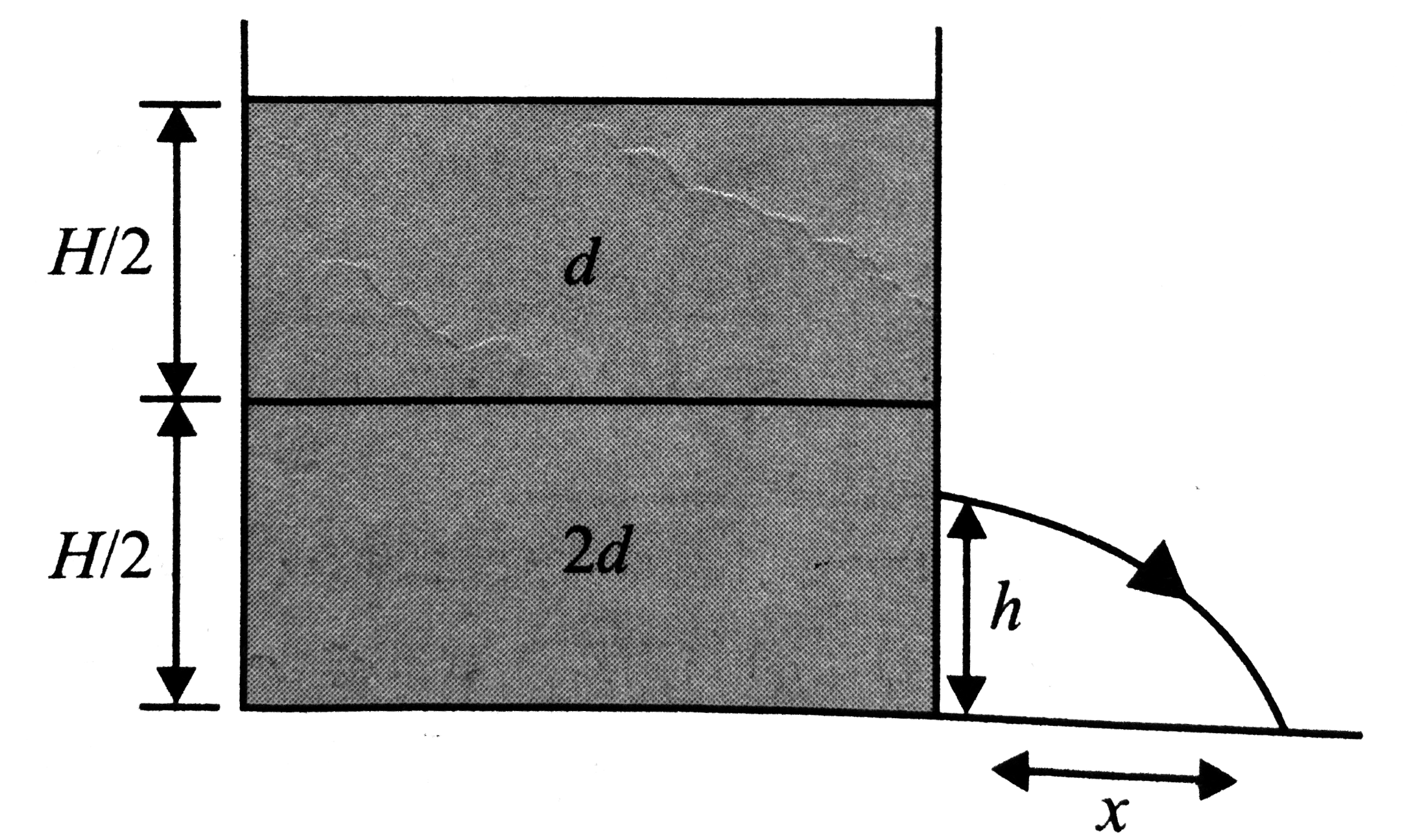
Situation I:
A homogeneous solid cylinder of length L(L<H/2). cross sectional area A/5 is immersed such that it floats with its axis vertical at liquid -liquid interface with lenght L/4 in the denser liquid.
The total pressure at the bottom of the container is
View SolutionA horizontal cylinder of length l contains water. On the left a constant force F is applied on the piston and on the right there is a small hole of area a. What is the velocity of the piston? Hence, calculate the work done in emptying the vessel in time t. brgt
 given area of cylinder is A
given area of cylinder is A
View SolutionA tank of height H and base area A is half filled with water and there is a small orifice at the bottom and there is a heavy solid cylinder having base area A3 and height of the cylinder is same as that of the tak. The water is flowing out of the orifice. Here cylinder is put into the tank to increase the speed of water flowing out.
After long time, when the height of water inside the tank again becomes equal to H2, the solid cylinder is taken out. then the velocity of liquid flowing out of the orifice (just after removing the cylinder) will be
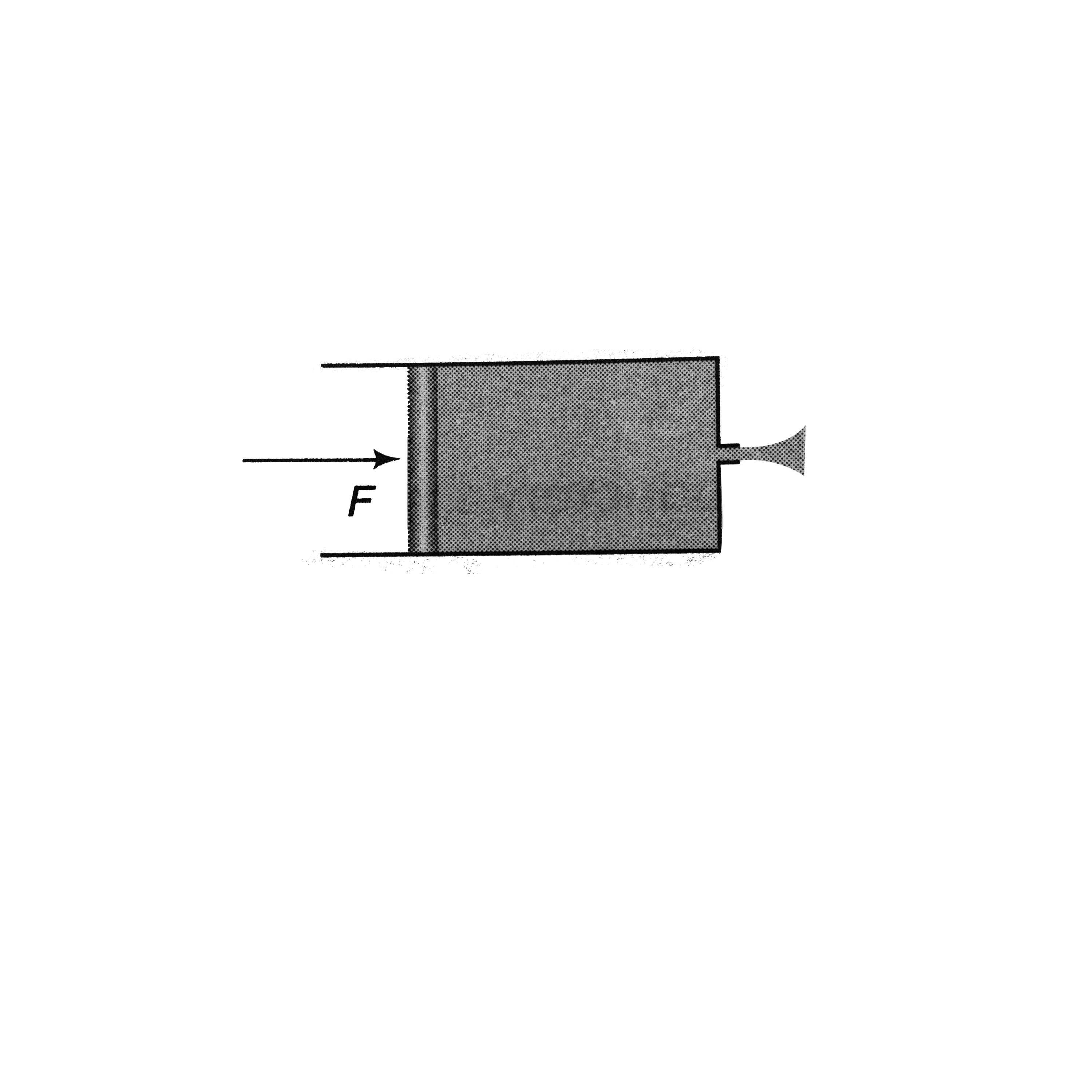
View SolutionWhat work should be done in order to squeeze all water from a horizontally located cylinder (figure) during the time t by means of a constant force acting on the piston? The volume of water in the cylinder is equal to V, the cross-sectional area of the orifice is s, with s being considerably less than the piston area. The friction and viscosity are negligibly small. Density of water is ρ
View Solution
