Question

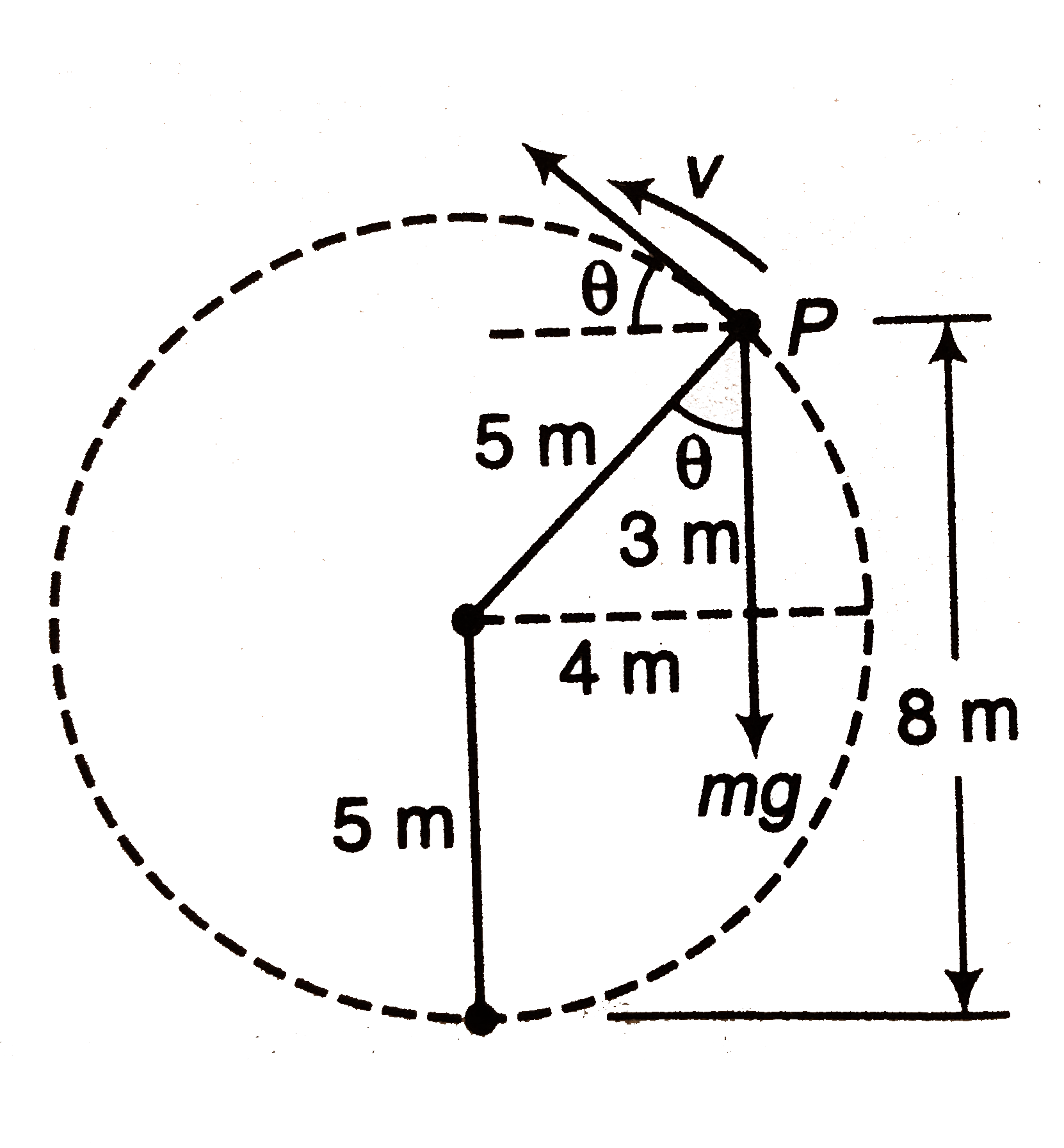
A particle is suspended from a fixed point by a string of lengh $5m$. It is projected from the equilibrium position with such a velocity that the string slakens after the particle has reached a height $8m$ above lowest point. Find the velocity of the particle, just before the string slackens.
Find also, to what to what height the particle can rise further?

Explanation

`T=0`
`mgcostheta=(mv^(2))/(R)`
or `gcostheta=(v^(2))/(R)`
or `(9.8)((3)/(5))`
`:. v=5.42m//s`
After point motion is projectile
`h=(v^(2)sin^(2)theta)/(2g)=((5.42)^(2)(4//5)^(2))/(2xx9.8)`
`=0.96m`