A partical of mass m and velocity v1 in positive y direction is projected on to a belt that is moving with uniform velocity v2 in x- direction as shown in figure. Coefficient of friction between partical and belt is μ . Assuming that the partical first touches the belt at the origin of fixed x−y coordinate system and remains on the belt , find co-ordinates (x−y) of the point where slidding stop.
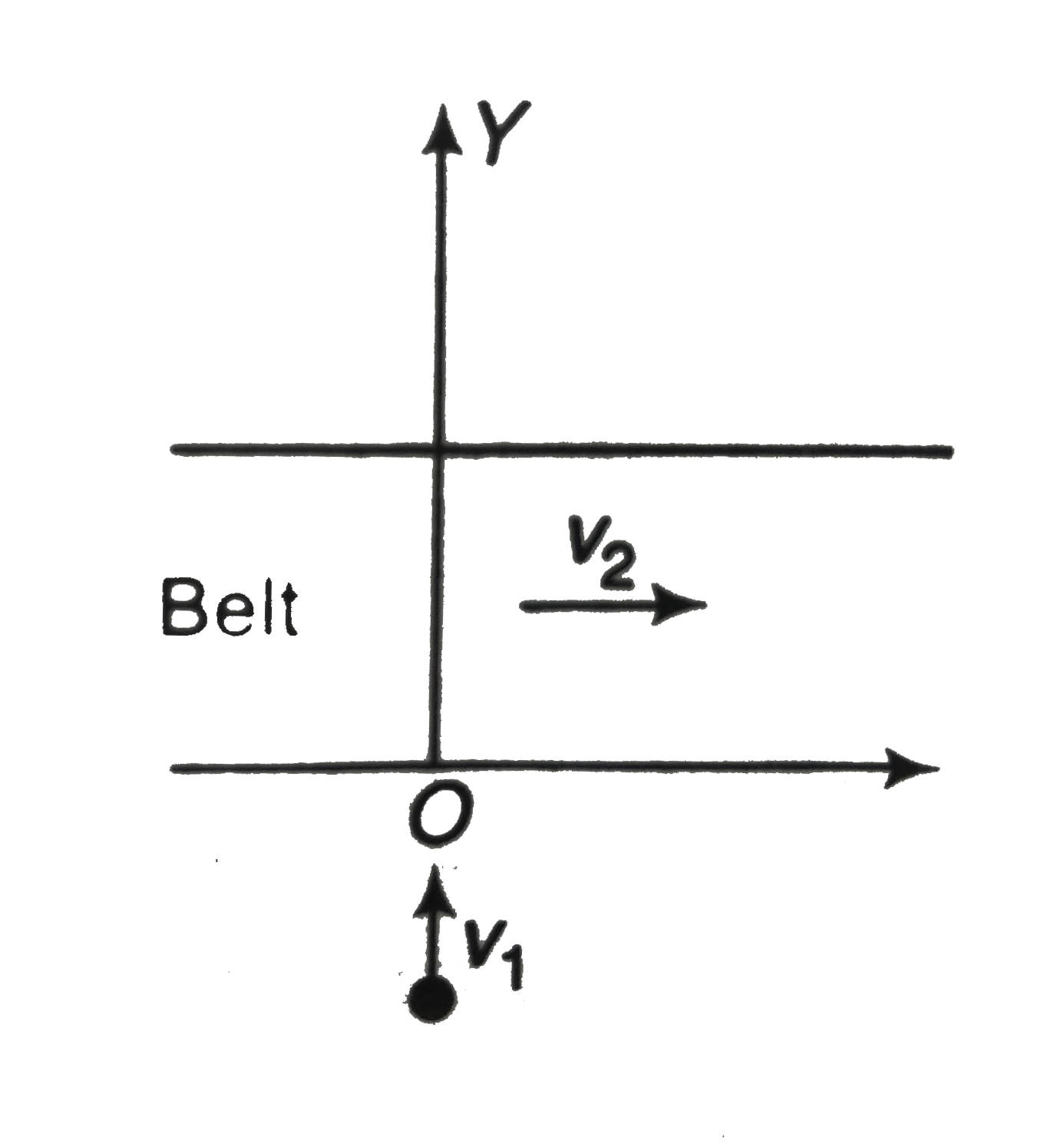
vr=√v21+v22
Reterdation a=μg
∴ Time when slipping stop is t=vra
or t=√v21+v22μg
sr=v2r2a=v21+v222μg
xr=−sr,cosθ=−(v21+v222μg)⎛⎜
⎜⎝v2√v21+v22⎞⎟
⎟⎠
−v2√v21+v222μg
yr=srsinθ=(v21+v222μg)⎛⎜
⎜⎝v1√v21+v22⎞⎟
⎟⎠<br>= (v_(1)sqrt (v_(1)^(2) + v_(2)^(2)))/(2 mu g)<br><imga≤ocr_imageload∈g=lazysrc=https://d10lpgp6xz60nq.cloudfront.net/physics_images/DCP_V01_C08_E01_198_S01.pngwh=80%mx-autosty≤=background:white;><br>Intimetbe<willmoveadistances = v_(2) t<br>orv_(2)sqrt (v_(1)^(2)) + v_(2)^(2))/( mu g)∈x−direction<br>Hence,c∞rd∈ateofpartic≤,<br>x = x_(r) + s = v_(2)sqrt (v_(1)^(2) + v_(2)^(2))/( 2 mu g)<br>andy = y_(r) = v_(1)sqrt (v_(1)^(2)) + v_(2)^(2))/( 2 mu g)
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Check point 5.120videos
LAWS OF MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Check point 5.215videos
LAWS OF MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Comprehension9videos
LAWS OF MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE15videos
KINEMATICS 1
- BOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 Subjective18videos
LAWS OF THERMODYNAMICS
Similar Questions
A particle of mass m moving with speed v in position x - direction collides perfectly inelastically with another identical particle moving with same speed in positive y - direction . Find final velocity of the combination.
View SolutionA particle is projected with a speed v0=√gR. The coefficient of friction the particle and the hemi- spherical plane is μ=0.5 Then , the acceleration of the partical is
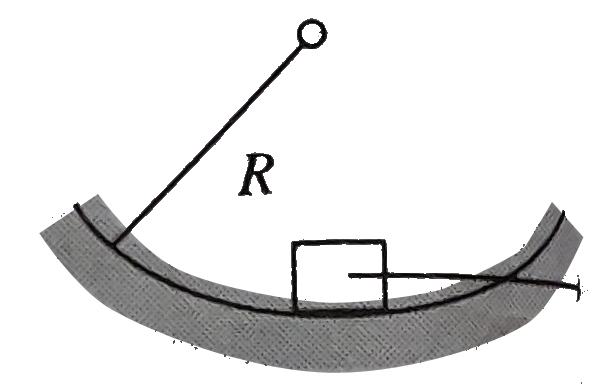 View Solution
View SolutionA particle of mass m1 moving with velocity v in a positive direction collides elastically with a mass m2 moving in opposite direction also at velocity v. If m2>>m1, then
View SolutionA lift moving with a uniform velocity v in which a block of mass m is lying .The friction force offered by the when coefficient of friction is μ=0.5 will be
View SolutionA charged particle (charge q, mass m) has velocity v0 at origin in +x direction. In space there is a uniform magnetic field B in -z direction. Find the y coordinate of particle when is crosses y axis.
View SolutionA skater of mass m standing on ice throws a stone of mass M with a velocity of v in a horizontal direction. The distance over which the skater will move back (the coefficient of friction between the skater and the ice is μ)
View SolutionWith what minimum velocity should be projected from left end A toward end B such that it reaches the other end B of conveyer belt moving with constant velocity v The friction coefficient between block and belt is μ
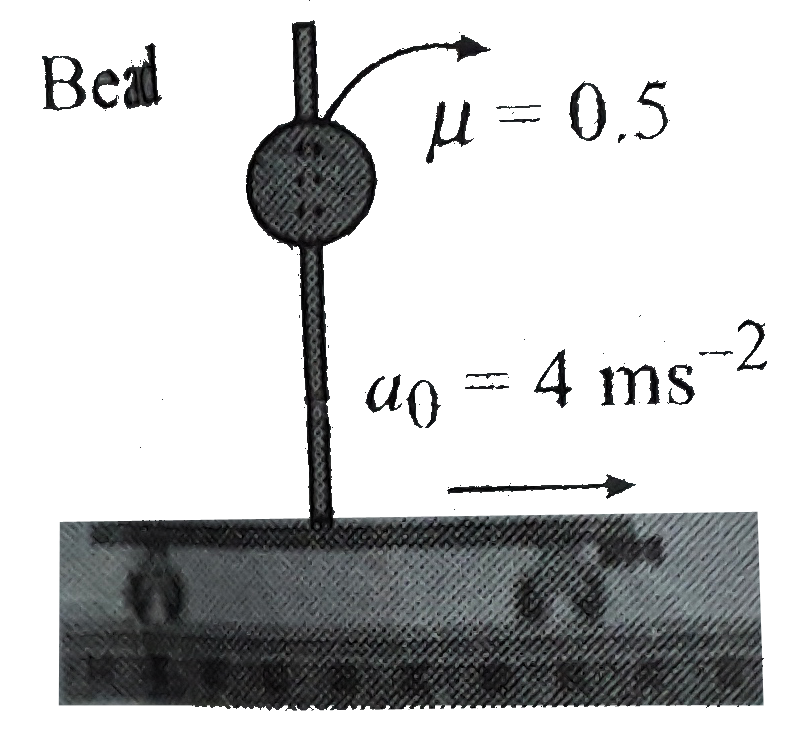 View Solution
View Solution
A solid sphere of mass m and radius R is gently placed on a conveyer belt moving with constant velocity v0. If coefficient of friction between belt and sphere is 2/7 the distance traveled by the centre of the sphere before it starts pure rolling isView SolutionFigure shows a man standing stationary with respect to a horizontal conveyor belt that is accelerating with l1ms−2 . The net force on the man, if the coefficient of static friction between the man's shoes and the belt is 0.2, up to what acceleration of the belt can the man continue to be stationary relative to the belt
 (mass of the man-65kg)View Solution
(mass of the man-65kg)View SolutionA particle is projected directly along a rough plane of inclination θ with velocity u. If after coming to the rest the particle returns to the starting point with velocity v, the coefficient of friction between the partice and the plane is
View Solution
