Inside a parallel plate capacitor of width 'd' a di-electric plate with dielectric constant k & width 3d/4 is inserted the new capacitance is C'. Before insertion of dielectric the capacitance was C0. Find relation between C0&C'
The correct Answer is:C, D
To find the relationship between the initial capacitance C0 and the new capacitance C′ after inserting a dielectric plate in a parallel plate capacitor, we can follow these steps:
1. Understand the Initial Capacitance:
The initial capacitance C0 of a parallel plate capacitor with air (or vacuum) between the plates is given by the formula:
C0=Aϵ0d
where:
- A is the area of the plates,
- ϵ0 is the permittivity of free space,
- d is the separation between the plates.
2. Insert the Dielectric Plate:
A dielectric plate with dielectric constant k and thickness t=3d4 is inserted between the plates. The remaining air gap will have a thickness of:
d−t=d−3d4=d4
3. Calculate the Capacitance with Dielectric:
The capacitor now consists of two sections in series:
- The section with the dielectric: thickness t=3d4 and capacitance C1:
C1=Aϵ0kt=Aϵ0k3d4=4Aϵ0k3d
- The section with air: thickness d4 and capacitance C2:
C2=Aϵ0d4=4Aϵ0d
4. Combine the Capacitances in Series:
The total capacitance C′ of the capacitor with the dielectric is given by the formula for capacitors in series:
1C′=1C1+1C2
Substituting the values of C1 and C2:
1C′=3d4Aϵ0k+d4Aϵ0
5. Find a Common Denominator:
The common denominator for the right-hand side is 4Aϵ0k:
1C′=3d4Aϵ0k+d⋅k4Aϵ0k=3d+dk4Aϵ0k
Therefore:
1C′=d(3+k)4Aϵ0k
6. Invert to Find C′:
Taking the reciprocal gives:
C′=4Aϵ0kd(3+k)
7. Relate C′ to C0:
Now, substituting C0=Aϵ0d into the equation for C′:
C′=4kC03+k
Final Relation:
Thus, the relationship between the initial capacitance C0 and the new capacitance C′ after inserting the dielectric is:
C′=4kC03+k
The initial capacitance C0 of a parallel plate capacitor with air (or vacuum) between the plates is given by the formula:
C0=Aϵ0d
where:
- A is the area of the plates,
- ϵ0 is the permittivity of free space,
- d is the separation between the plates.
A dielectric plate with dielectric constant k and thickness t=3d4 is inserted between the plates. The remaining air gap will have a thickness of:
d−t=d−3d4=d4
The capacitor now consists of two sections in series:
- The section with the dielectric: thickness t=3d4 and capacitance C1:
C1=Aϵ0kt=Aϵ0k3d4=4Aϵ0k3d
- The section with air: thickness d4 and capacitance C2:
C2=Aϵ0d4=4Aϵ0d
The total capacitance C′ of the capacitor with the dielectric is given by the formula for capacitors in series:
1C′=1C1+1C2
Substituting the values of C1 and C2:
1C′=3d4Aϵ0k+d4Aϵ0
The common denominator for the right-hand side is 4Aϵ0k:
1C′=3d4Aϵ0k+d⋅k4Aϵ0k=3d+dk4Aϵ0k
Therefore:
1C′=d(3+k)4Aϵ0k
Taking the reciprocal gives:
C′=4Aϵ0kd(3+k)
Now, substituting C0=Aϵ0d into the equation for C′:
C′=4kC03+k
Thus, the relationship between the initial capacitance C0 and the new capacitance C′ after inserting the dielectric is:
C′=4kC03+k
Topper's Solved these Questions
- JEE MAIN 2021Book:JEE MAINS PREVIOUS YEARChapter:JEE MAIN 2021Exercise:SECTION-A
Explore 80 Videos
- JEE MAIN 2021Book:JEE MAINS PREVIOUS YEARChapter:JEE MAIN 2021Exercise:SECTION-B
Explore 40 Videos
- JEE MAINBook:JEE MAINS PREVIOUS YEARChapter:JEE MAINExercise:All Questions
Explore 473 Videos
- JEE MAIN 2022Book:JEE MAINS PREVIOUS YEARChapter:JEE MAIN 2022Exercise:Question
Explore 492 Videos
Similar Questions
Find an expression for the capacitance of a parallel plate capacitor when a dielectric slab of dielectric constant K and thickness t=d2 but the same area on the plates is inserted between the the capacitors plate. (d=separation between the plates)
View SolutionThe capacitance of a parallel plate capacitor isCa (Fig. a). A dielectric of dielectric constant K is inserted as shown in fig. (b) and (c). If Cb and Cc denote the capacitances in fig. (b) and (c), then
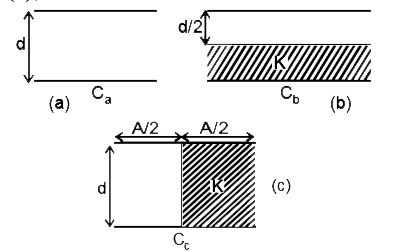
View Solution
Knowledge Check
Capacitor of capacitance C with oil between the plates of dielectric constant 2. If oil is removed, the capacitance is .
A2 CB√2CCC√2DC2A parallel plate capacitor with air between plates (dielectric constant K =2) has a capacitance C. If the air is removed, then capacitance of the capacitor is
AC√2BC2C√2CD2CA parallel plate capacitor with oil as a dielectric between the plates has a capacitance C. if the oil, with dielectric constannt (K=3), is removed, then the capacitance of the capacitor will be
A3CBC√3CC3D√3C
A parallel plate capacitor with a sheet of paper (dielectric constant K) between the plates has a capacitance C. If the sheet is removed then capacitance of the capacitor becomes
View SolutionA paralle plate air capacitor has capacitance C. Now half of the space is fillel with a dielectric material with dielectric constant K as shown in figure . The new capacitance is C . Then

View SolutionA parallel plate capacitor has a capacity c. If a medium of dielectric constant K is introduced between plates, the capacity of capacitor becomes
View SolutionA parallel plate condenser with oil between the plates (dielectric constant of oil K = 2 ) has a capacitance C. if the oil is removed , then capacitance of the capacitor becomes
View SolutionA parallel plate condenser with oil (dielectric constant 2) between the plates has capacitance C. If oil is removed, the capacitance of capacitor becomes
View Solution