If the Point (a3/(a−1),(a2−3)/(a−1)),(b2/(b−1),(b2−3)/(b−1)),and(c3/c−1,c2−3c−1), where a,b,c are different from 1, lie on the line lx+my+n=0 thenSolution in Telugu
a+b+c=−ml
ab+bc+ca=nl
abc=(m+n)l
abc−(bc+ca+ab)+3(a+b+c)=0
The correct Answer is:A, C
Step by step video, text & image solution for If the Point (a^3//(a-1),(a^2-3)//(a-1)),(b^2//(b-1),(b^2-3)//(b-1)), and (c^3//c-1,(c^2-3)/(c-1)), where a,b,c are different from 1, lie on the line lx+my+n=0 then by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- STRAIGHT LINESBOOK - AAKASH SERIESCHAPTER - STRAIGHT LINESEXERCISE - LECTURE SHEET(EXERCISE-III Problems on Point of intersection of line and concurrency of lines)(Linked comprhension Type Questions Type Question)
3 Videos
- STRAIGHT LINESBOOK - AAKASH SERIESCHAPTER - STRAIGHT LINESEXERCISE - LECTURE SHEET(EXERCISE-III Problems on Point of intersection of line and concurrency of lines)(Matrix Matching Type Questions Type Question)
2 Videos
- STRAIGHT LINESBOOK - AAKASH SERIESCHAPTER - STRAIGHT LINESEXERCISE - LECTURE SHEET(EXERCISE-III Problems on Point of intersection of line and concurrency of lines)(Straight Objective Type Questions Type Question)
22 Videos
- REVISION EXERCISEBOOK - AAKASH SERIESCHAPTER - REVISION EXERCISEEXERCISE - PROPERTIES OF TRIANGLES
57 Videos
- TANGENT AND NORMAL BOOK - AAKASH SERIESCHAPTER - TANGENT AND NORMAL EXERCISE - ADVANCED SUBJECTIVE TYPE QUESTIONS
48 Videos
Similar Questions
Let L be the line belonging to the family of straight lines (a+2b)x+(a−3b)y+a−8b=0a,b∈R, which is the farthest from the point (2,2)
The equation of line L is
View SolutionMatch the following
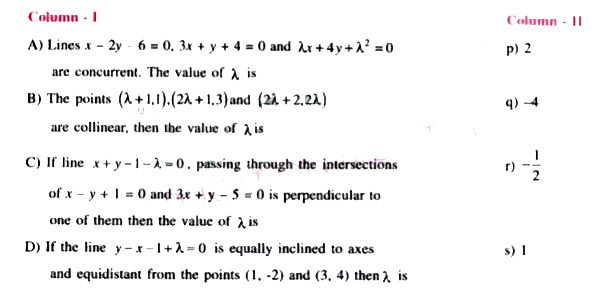
View SolutionThe value of k such that the lines 2x−3y+k=0,3x−4y−13=0 and 8x−11y−33=0 are concurrent, is
View SolutionIn a triangle ABC, if A,B, C are in A.P and b:c =√3:√2, then
View SolutionFind the equations of tangent and normal to the curve
(xa)4+(yb)4=2 at (a,b)
View SolutionIf the points ((a^(3))/(a-1),(a^(2)-3)/(a-1))((b^(3))/(b-1),(b^(2)-3)/(b-1)),((c^(3))/(c-1),(c^(2)-3)/(c-1)) are a!=1,b!=1,c!=1, then find the value of abc-(ab+bc+ca)+3(a+b+c)
View SolutionIf the points (a3(a−1)),((a2−3)(a−1)),(b3b−1),(b2−3(b−1)),(c3c−1) and ((c2−3)(c−1)), where a,b,c are different from 1, lie on the lx+my+n=0 , then (a) a+b+c=−ml (b)ab+bc+ca=nl (c)abc=(m+n)l (d)abc−(bc+ca+ab)+3(a+b+c)=0
View SolutionIf the points (a3a−1,a2−3a−1),(b3b−1,b3−3b−1)and(c3c−1,c3−3c−1) where a,b,c are different from 1 lie on the line lx+my+n=0 a+b+c=−ml ab+bc+ca+nl=0 abc=(3m+n)l abc−(bc+ca+ab)+3(a+b+c)=0
View SolutionIf a+b+c=1,a^(2)+b^(2)+c^(2)=9 and a^(3)+b^(3)+c^(3)=1, then (1)/(a)+(1)/(b)+(1)/(c) is (i)0 (ii) -1(iii)1(iv)3
View SolutionIf a!=b!=c such that |[a^3-1,b^3-1,c^3-1] , [a,b,c] , [a^2,b^2,c^2]|=0 then
View Solutionसिद्ध करे कि
tan−1a3−b31+a3b3+tan−1b3−c31+b3c3+tan−1c3−a31+c3a3=tan−1a2−b21+a2b2+tan−1b2−c21+b2c2+tan−1c2−a21+c2a2
View SolutionIf a,b,c are different and ∣∣ ∣ ∣∣aa2a3−1bb2b3−1cc2c3−1∣∣ ∣ ∣∣=0 then
View SolutionIf a,b,c are all different and ∣∣ ∣ ∣∣1+a21+b21+c2abca3b3c3∣∣ ∣ ∣∣=0 then a+b+c=
View SolutionIf ∣∣ ∣ ∣∣aa21+a3bb21+b3cc21+c3∣∣ ∣ ∣∣=0 and vectors (1,a,a2),(1,b,b2) and (1,c,c2) are non coplanar then the product abc=
View SolutionIf a≠be≠c such that
∣∣ ∣ ∣∣a3−1b3−1c3−1abca2b2c2∣∣ ∣ ∣∣=0 then,
View Solution
