In ΔABC, AD and BE are altitudes. If BC=8, AC=12 and AD=6, find ar (ABC) and BE. Solution in Gujrati
The correct Answer is:ar (ABC)=24 ,BE=4
Step by step video, text & image solution for In DeltaABC, AD and BE are altitudes. If BC=8, AC=12 and AD=6, find ar (ABC) and BE. by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
- QUESTION PAPER 2: FOR THE SECOND TESTBOOK - KUMAR PRAKASHANCHAPTER - QUESTION PAPER 2: FOR THE SECOND TESTEXERCISE - SECTION C (Solve the following :)
4 Videos
- QUESTION PAPER 2: FOR THE SECOND TESTBOOK - KUMAR PRAKASHANCHAPTER - QUESTION PAPER 2: FOR THE SECOND TESTEXERCISE - SECTION D (Solve the following :)
5 Videos
- QUESTION PAPER 2: FOR THE SECOND TESTBOOK - KUMAR PRAKASHANCHAPTER - QUESTION PAPER 2: FOR THE SECOND TESTEXERCISE - SECTION D (Solve the following :)
5 Videos
- QUADRILATERALSBOOK - KUMAR PRAKASHANCHAPTER - QUADRILATERALSEXERCISE - MULTIPLE CHOICE QUESTION(MCQs)
15 Videos
- QUESTION PAPER 3 : FOR THE ANNUAL EXAMINATION BOOK - KUMAR PRAKASHANCHAPTER - QUESTION PAPER 3 : FOR THE ANNUAL EXAMINATION EXERCISE - SECTION -D
5 Videos
Similar Questions
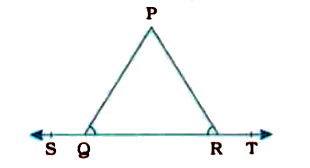
In the given figure, ∠PQR=∠PRQ, then prove that ∠PQS=∠PRT.
View SolutionLocate √3 on number line
View SolutionLocate √3 on number line
View SolutionThe ratio of four angles in order of a quadrilateral is 2:4:5: 4. Then, the measure of the smallest angle of the quadrilateral is ………..
View SolutionProve that the angles opposite to equal sides of an isosceles triangle are equal.
View SolutionIn Delta ABC,AD perp BC,AD=6,BC=8 then find distance between mid-points of BD and AC
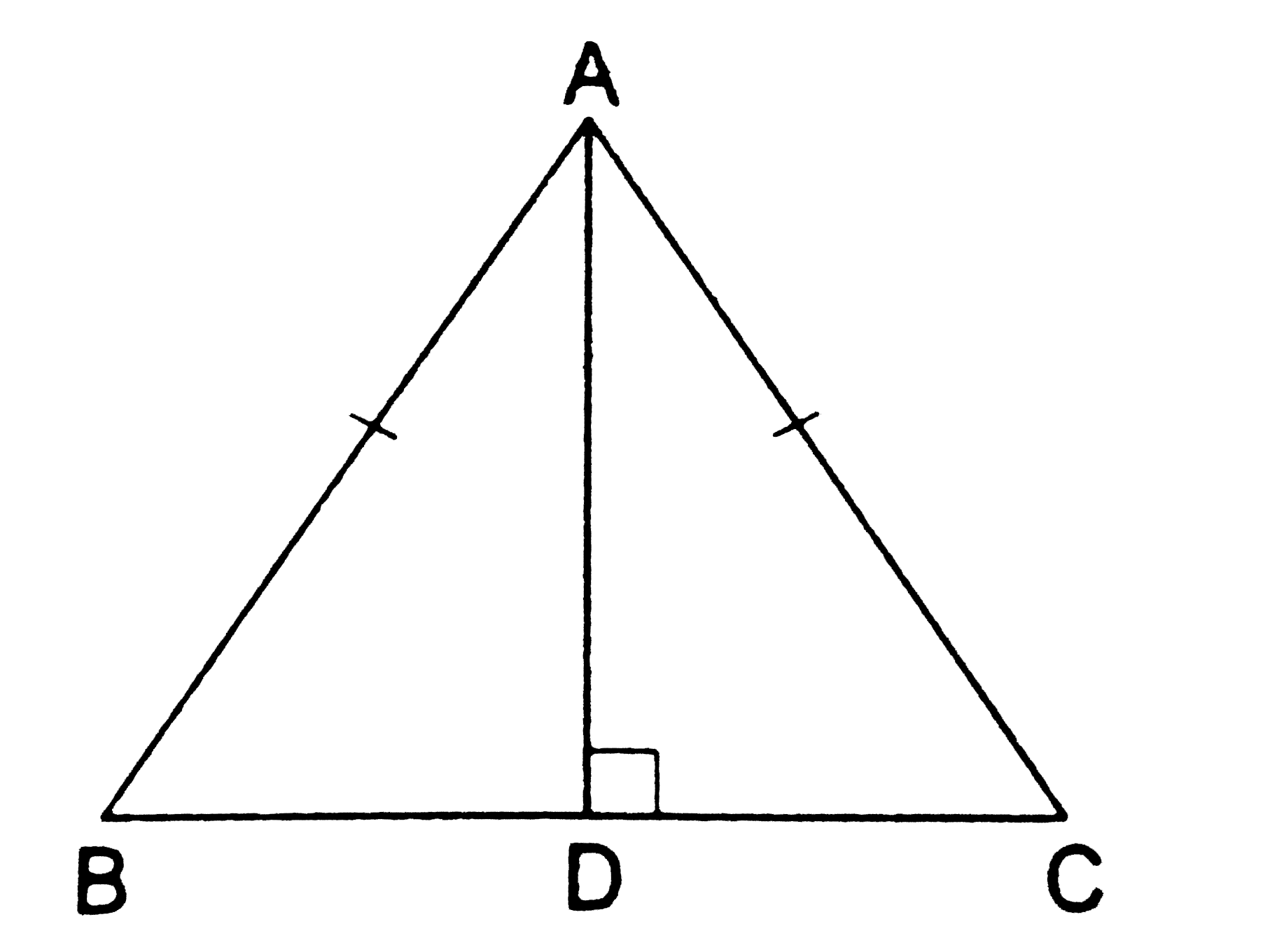
View SolutionAD is an altitude of an isosceles ΔABC in which AB = AC.
Show that (i) AD bisects BC,
(ii) AD bisects ∠A.
View Solution(a) ΔABC में , AB=5 सेमी , BC = 13 सेमी तथा AC = 12 सेमी है । यदि BC का मध्य बिंदु D है , तो माध्यिका AD कि माप ज्ञात कीजिए ।
(b) निम्न चित्र में ,ΔABC की माध्यिक AD=6 सेमी है । यदि आधार BC = 8 सेमी हो तो का AB2+AC2 मान ज्ञात कीजिए ।
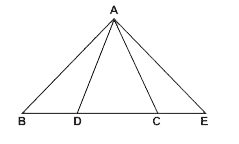
View SolutionIn the given figure ar (ΔABC)= ar (ΔADE). AC is the median of ΔADE. If ar (ACE) =14cm2 then ar (ΔABC) = ?
View Solution△ABC is a right angled at A and AD is the altitude to BC. If AB= 7 cm and AC = 24 cm. Find the ratio of AD is to AM if M is the mid-point of BC.
View SolutionAD is an altitude of an isosceles triangle ABC in which AB = AC. Show that, (i) AD bisects BC (ii) AD bisects ∠A.
View SolutionAD is an altitude of an isosceles triangle ABC in which AB = AC. Show that, (i) AD bisects BC (ii) AD bisects ∠A.
View SolutionAD is an altitude of an isosceles triangle ABC in which AB = AC. Show that, (i) AD bisects BC (ii) AD bisects ∠A.
View SolutionAD is an altitude of an isosceles triangle ABC in which AB = AC. Show that, (i) AD bisects BC (ii) AD bisects ∠A.
View SolutionAD is an altitude of an isosceles ΔABC in which AB = AC.
Show that (i) AD bisects BC,
(ii) AD bisects ∠A.

View Solution
