In a parallelogram ABCD, P is the mid point of the side AD. The line BP cuts the diagonal AC in the point Q. Then the ratio AQ:QC= Solution in Telugu
1:3
3:1
2:1
1:2
The correct Answer is:D
Step by step video, text & image solution for In a parallelogram ABCD, P is the mid point of the side AD. The line BP cuts the diagonal AC in the point Q. Then the ratio AQ:QC= by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- APPENDICES (REVISION EXERCISE)BOOK - AAKASH SERIESCHAPTER - APPENDICES (REVISION EXERCISE)EXERCISE - DOT PRODUCT OF TWO VECTORS
34 Videos
- APPENDICES (REVISION EXERCISE)BOOK - AAKASH SERIESCHAPTER - APPENDICES (REVISION EXERCISE)EXERCISE - CROSS PRODUCT OF TWO VECTORS
17 Videos
- APPENDICES (REVISION EXERCISE)BOOK - AAKASH SERIESCHAPTER - APPENDICES (REVISION EXERCISE)EXERCISE - LINEAR EQUATIONS
13 Videos
- 3D-COORDINATE SYSTEMBOOK - AAKASH SERIESCHAPTER - 3D-COORDINATE SYSTEMEXERCISE - PRACTICE EXERCISE
40 Videos
- CHANGE OF AXESBOOK - AAKASH SERIESCHAPTER - CHANGE OF AXESEXERCISE - Practice Exercise
29 Videos
Similar Questions
If AD, BE and CF are the medians of ΔABC, then the value of ¯¯¯¯¯¯BC.¯¯¯¯¯¯AD+¯¯¯¯¯¯CA.¯¯¯¯¯¯BE+¯¯¯¯¯¯AB.¯¯¯¯¯¯CF=
View SolutionLet ¯aand¯b be two non-collinear unit vectors. If ¯u=¯a−(¯a.¯b)¯band¯v=¯aׯb, then |¯v|=
View SolutionIf a+b+c≠0, the system of equations
(b+c)(y+z)−ax=b−c,
(c+a)(z+x)−by=c−a,
(a+b)(x+y)−cz=a−b has
View SolutionThe point (-1,4,-2) lie in the octant
View SolutionThe coordinates of the point (3,-5) in the new system when the origin is shifted to (-2,3) are
View SolutionP is the mid-point of the side AD of the parallelo- gram ABCD.The line BP meets the diagonal AC in Q and the line CD in R .Then RQ:QB is equal to
View SolutionABCD is a parallelogram and the segment bar(AD) dividing it internally in the ratio 3:1 the line BP meet the diagonal AC in Q. Then the ratio AQ:QC is
View SolutionABCD is a parallelogram P is a point on AD suchAP.that (AP)/(AD)=1/2013. Q is the point of intersection of AC and BP. Calculate (AQ)/(AC)=
View SolutionIf P and Q are the mid points of the sides AB and CD of a parallelogram ABCD, prove that DP and BQ cut the diagonal AC in its points of trisection which are also the points of trisection of DP and BQ respectively.
View SolutionIn a parallelogram ABCD, E and F are the mid-points of sides BC and AD respectively. Show that the line segment BF and ED trisect the diagonal AC.
View SolutionA transversal cuts side AB and AD and diagonal AC of parallelogram ABCD in points P,Q and R respectively such that AP: PB =1 :2 and AQ: QD=2:3 . Find the ratio AR : RC
View SolutionIn parallelogram ABCD, if P is the mid-point of side AB, then
View SolutionABCD is a quadrilateral in which P, Q, R and S are mid- points of the sides AB, BC, CD and DA. AC is a diagonal. Show that :
PQRS is a parallelogram.
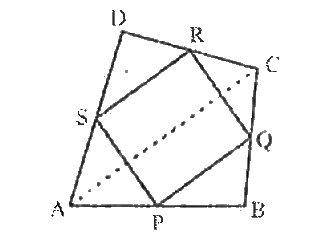
View SolutionABCD is a parallelogram and P is themid point of the side AD. The line BP meets the diagonal AC in Q. Then the ratio AQ : QC =
View SolutionABCD is a parallelogram and P is the midpoint of the side AD. The line BP meets the diagonal AC in Q. Then, the ratio of AQ:QC is equal to
View Solution
