A particle starts performing SHM on a smooth horizontal plane and it is released from x=A2 and it is moving in -ve x-direction then ϕ=?
π6
5π6
2π3
π3
The correct Answer is:B
To solve the problem, we need to determine the phase angle ϕ of a particle performing simple harmonic motion (SHM) given that it is released from x=A2 and is moving in the negative x-direction.
1. Understanding the SHM Equation:
The displacement x of a particle in SHM can be expressed as:
x=Asin(ωt+ϕ)
where:
- A is the amplitude,
- ω is the angular frequency,
- t is the time,
- ϕ is the phase angle.
2. Initial Conditions:
At the moment of release, we set t=0 and x=A2. Substituting these values into the SHM equation gives:
A2=Asin(ϕ)
3. Simplifying the Equation:
Dividing both sides by A (assuming A≠0):
12=sin(ϕ)
4. Finding Possible Values of ϕ:
The values of ϕ that satisfy sin(ϕ)=12 are:
ϕ=π6andϕ=5π6
5. Determining the Direction of Motion:
We know that the particle is moving in the negative x-direction. To find out which ϕ corresponds to this direction, we need to analyze the velocity.
6. Velocity in SHM:
The velocity v of the particle is given by the derivative of the displacement:
v=dxdt=Aωcos(ωt+ϕ)
At t=0:
v=Aωcos(ϕ)
Since the particle is moving in the negative x-direction, we require:
v<0⇒cos(ϕ)<0
7. Analyzing the Cosine Values:
- For ϕ=π6, cos(π6)>0 (positive).
- For ϕ=5π6, cos(5π6)<0 (negative).
8. Conclusion:
Since we need cos(ϕ)<0 for the velocity to be negative, we conclude that:
ϕ=5π6
Final Answer:
ϕ=5π6
The displacement x of a particle in SHM can be expressed as:
x=Asin(ωt+ϕ)
where:
- A is the amplitude,
- ω is the angular frequency,
- t is the time,
- ϕ is the phase angle.
At the moment of release, we set t=0 and x=A2. Substituting these values into the SHM equation gives:
A2=Asin(ϕ)
Dividing both sides by A (assuming A≠0):
12=sin(ϕ)
The values of ϕ that satisfy sin(ϕ)=12 are:
ϕ=π6andϕ=5π6
We know that the particle is moving in the negative x-direction. To find out which ϕ corresponds to this direction, we need to analyze the velocity.
The velocity v of the particle is given by the derivative of the displacement:
v=dxdt=Aωcos(ωt+ϕ)
At t=0:
v=Aωcos(ϕ)
Since the particle is moving in the negative x-direction, we require:
v<0⇒cos(ϕ)<0
- For ϕ=π6, cos(π6)>0 (positive).
- For ϕ=5π6, cos(5π6)<0 (negative).
Since we need cos(ϕ)<0 for the velocity to be negative, we conclude that:
ϕ=5π6
ϕ=5π6
Topper's Solved these Questions
- JEE MAIN 2021Book:JEE MAINS PREVIOUS YEARChapter:JEE MAIN 2021Exercise:SECTION-A
Explore 80 Videos
- JEE MAIN 2021Book:JEE MAINS PREVIOUS YEARChapter:JEE MAIN 2021Exercise:SECTION-B
Explore 40 Videos
- JEE MAINBook:JEE MAINS PREVIOUS YEARChapter:JEE MAINExercise:All Questions
Explore 473 Videos
- JEE MAIN 2022Book:JEE MAINS PREVIOUS YEARChapter:JEE MAIN 2022Exercise:Question
Explore 492 Videos
Similar Questions
A particle is performing SHM along x−axis with amplitude 4.0cm and time period 1.2s . What is the minimum time is deci− second taken by the particle to move from x=+2cmto x=+4cm and back again.
View SolutionA particle strikes a smooth horizontal surface at an angle θ>0 and it rebounds at an angle ϕ as shown
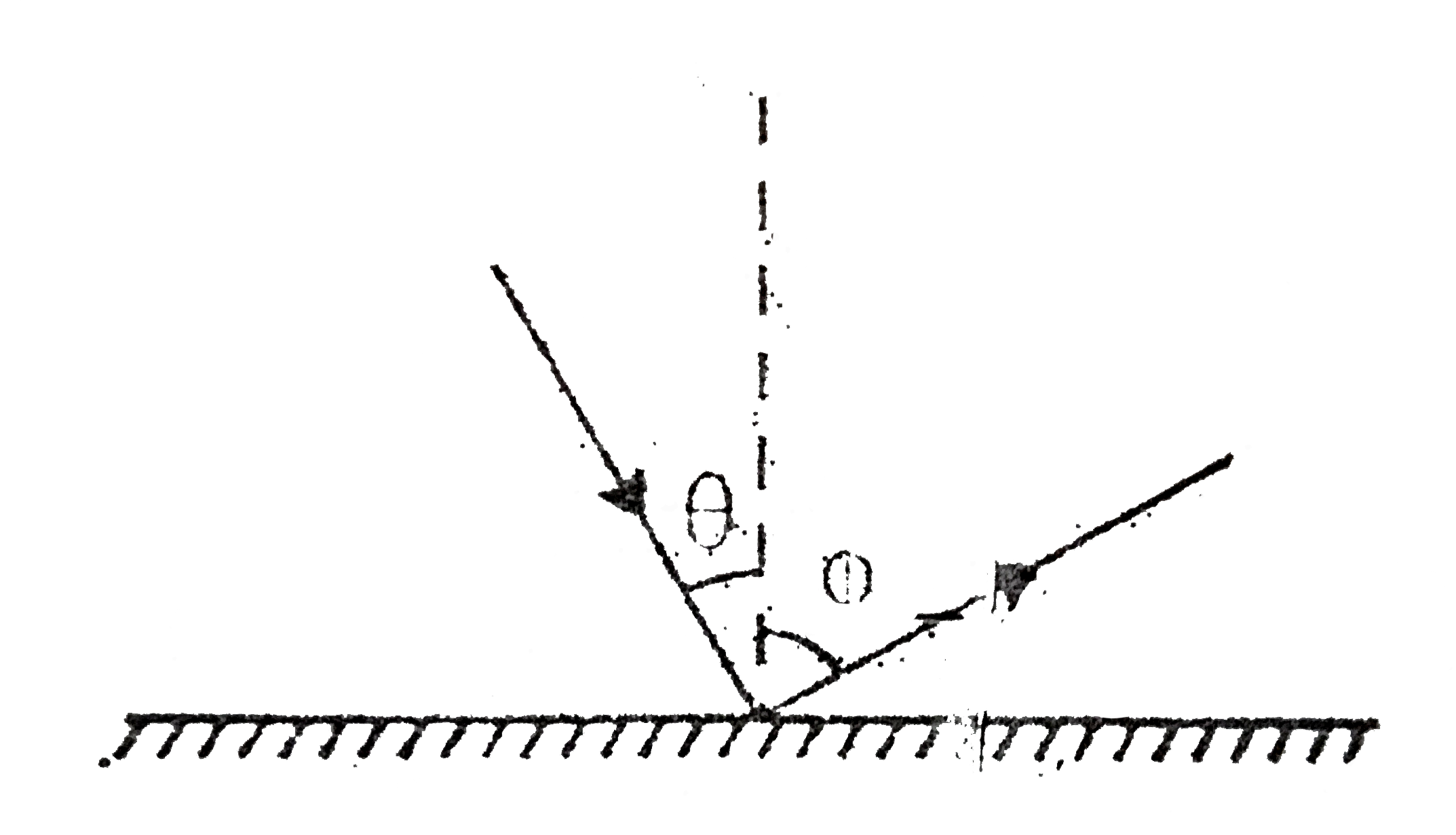
View Solution
Knowledge Check
The displacement-time equation of a particle executing SHM is A−Asin(ωt+ϕ). At tme t=0 position of the particle is x=A2 and it is moving along negative x-direction. Then the angle ϕ can be
Aπ6Bπ3C2π3D5π6Two particles starting from a point on a circle of radius 4m in horizontal plane move along the respectively in opposite directions. The particle will collide with each other after a time of
A3.0sB2.5sC2.0sD1.5sThe displecemen-time equation of a particle execitting SHM is x=Asin(ωt+ϕ) At time t=0 position of are position is x=A/2 and it is moving along negative x−direction .Then the angle ϕ can be
A[1k1+1k2]1B(4t−π6)C12π(k1+k2M)1/2D12π(k1−k2M)1/2
A particle performs SHM in a straight line. In the first second, starting from rest, it travels a distance a and in the next second it travels a distance b in the same direction. The amplitude of the SHM is
View SolutionA particle performing SHM starts from mean position. The phase of that particle is π/s when it has
View SolutionA particle P lying on a smooth horizontal x−y plane starts from (3ˆi+4ˆj)m with velocity (2ˆi)m/s. Another particle Q is projected (horizontally from origin with velocity (xˆi+yˆj) so that is strikes P after 2s. Then
View SolutionThe equation of a progressive wave moving in +ve X-direction is given by -
View SolutionA particle is performing SHM along x-axis such that its acceleration along x-axis is : a=2−x where a is in m/s2 and x is in meter. If speed of the particle at x = 1 is zero in INCORRECT statement is :
View Solution

