If electric field is $3 ˆ i + 4 ˆ j$ then find out ratio of flux when a rectangular plane is put on $x - z$ plane and when same plane is put on $y - z$ plane.

A

$ϕ_{1} : ϕ_{2} = 4 : 3$

B

$ϕ_{1} : ϕ_{2} = 3 : 4$

C

$ϕ_{1} : ϕ_{2} = 2 : 3$

D

$ϕ_{1} : ϕ_{2} = 3 : 2$

Video Solution

Text Solution

Generated By DoubtnutGPT

The correct Answer is:A

To solve the problem of finding the ratio of electric flux through two rectangular planes placed on the x-z and y-z planes, we will follow these steps:
Step 1: Understand the Electric Field and Area Vectors
The electric field is given as E = 3i + 4j. We need to determine the area vectors for the two surfaces:
- For the rectangular plane on the x-z plane, the area vector A1 will be in the y-direction (perpendicular to the x-z plane).
- For the rectangular plane on the y-z plane, the area vector A2 will be in the x-direction (perpendicular to the y-z plane).
Step 2: Define the Area Vectors
- **A1 = A * j (where A is the area of the rectangle)
- A2 = A * i
Step 3: Calculate the Electric Flux for Each Surface
The electric flux (Φ) through a surface is given by the formula:
$Φ = E \cdot A = | E | | A | cos (θ)$
where θ is the angle between the electric field vector and the area vector.
For Surface 1 (x-z plane):
- The area vector A1 is in the y-direction** (A1 = A * j).
- The electric field E has components in both the x and y directions.
- The angle θ between E and A1 is 0° for the j component of E (4j) and 90° for the i component (3i).
Calculating the flux:
$Φ_{1} = E \cdot A 1 = (3 i + 4 j) \cdot (A * j)$
$Φ_{1} = 3 A \cdot 0 + 4 A \cdot 1 = 4 A$
For Surface 2 (y-z plane):
- The area vector A2 is in the x-direction (A2 = A * i).
- The angle θ between E and A2 is 0° for the i component of E (3i) and 90° for the j component (4j).
Calculating the flux:
$Φ_{2} = E \cdot A 2 = (3 i + 4 j) \cdot (A * i)$
$Φ_{2} = 3 A \cdot 1 + 4 A \cdot 0 = 3 A$
Step 4: Find the Ratio of the Fluxes
Now we can find the ratio of the fluxes:
$\frac{Φ_{1}}{Φ_{2}} = \frac{4 A}{3 A} = \frac{4}{3}$
Final Answer
The ratio of the electric flux when the rectangular plane is placed on the x-z plane to when it is placed on the y-z plane is:
$\frac{Φ_{1}}{Φ_{2}} = \frac{4}{3}$

|

Updated on:18/9/2024

Class 12 PHYSICS QUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
If $\to E = \frac{3}{5} ˆ i + \frac{4}{5} ˆ j$ then find electric flux through an area of 0.4 m^2 parallel to y-z plane
A $0.12 N \frac{m^{2}}{C}$
B $0.24 N \frac{m^{2}}{C}$
C $0.36 N \frac{m^{2}}{C}$
D $0.48 N \frac{m^{2}}{C}$
Question 2 - Select One
If the uniform electric field is $3 \times 10^{3} ˆ i N C^{- 1}$ , then the flux of this field through a square of 10 cm on a side whose plane is parallel to the y-z- plane gt?
A $30 N m^{2} C^{- 1}$
B $60 N m^{2} C^{- 1}$
C $15 N m^{2} C^{- 1}$
D $25 N m^{2} C^{- 1}$
Question 3 - Select One
In a region of uniform electric field $\to E = E_{0} ˆ i$ a semi cylinder is placed with it's rectangular shaped plane surface in y-z plane so that x-axis is normal to the plane surface. Given $l$ is length and $r$ is radius of cylinder the flux with curved surface alone is

A $E r l$
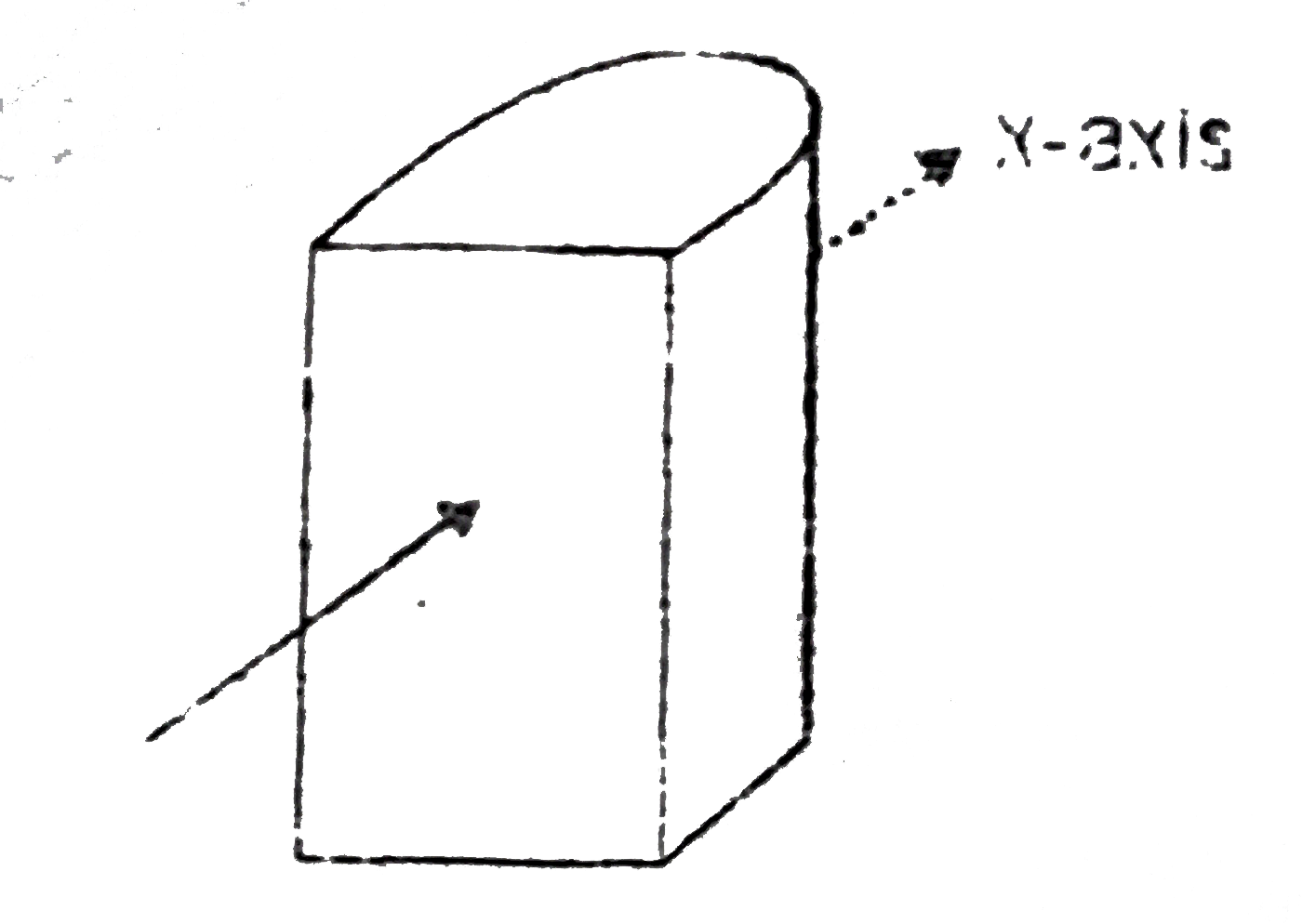B $\frac{E r l}{2}$
C $2 E r l$
DZero

JEE MAINS PREVIOUS YEAR-JEE MAIN 2021-PHYSICS SECTION B

Home
Ask Doubt
Profile