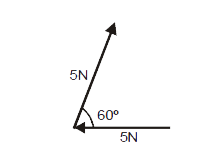
Two forces, each numerically equal to 5 N, are acting as shown in the figure. Then the resultant is
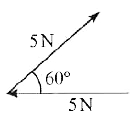
2.5 N
5 N
5√3N
10 N
The correct Answer is:B
The angle between the given forces is 120∘ and not 60∘
Resultant force is
∣∣→R∣∣=√∣∣→A∣∣2+∣∣→B∣∣2+2×∣∣→A∣∣∣∣→B∣∣cosθ
=√(5)2+(5)2+2×(5)×(5)×(−12)=5N
Topper's Solved these Questions
- KINEMATICS BOOK - MTG-WBJEECHAPTER - KINEMATICS EXERCISE - WB JEE WORKOUT ( CATEGORY 2 : Single Option Correct Type (2 Marks))
15 Videos
- KINEMATICS BOOK - MTG-WBJEECHAPTER - KINEMATICS EXERCISE - WB JEE WORKOUT ( CATEGORY 3 : One or More than One Option Correct Type (2 Marks))
10 Videos
- HEAT AND THERMAL PHYSICSBOOK - MTG-WBJEECHAPTER - HEAT AND THERMAL PHYSICSEXERCISE - WB JEE PREVIOUS YEARS QUESTIONS
15 Videos
- KINETIC THEORY OF GASES BOOK - MTG-WBJEECHAPTER - KINETIC THEORY OF GASES EXERCISE - WB JEE PREVIOUS YEARS QUESTIONS (MCQ)
7 Videos
Similar Questions
Knowledge Check
Two forces , each numerically equal to 10 dyne are acting as shown in the following figure Their resultant is :
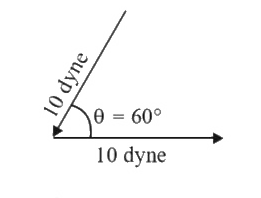 A10 dyneB20 dyneC10√3 dyneD5 dyne
A10 dyneB20 dyneC10√3 dyneD5 dyneTwo forces each numerically equal to 10 dynes are acting as shown in the following figure, then their resultant is -
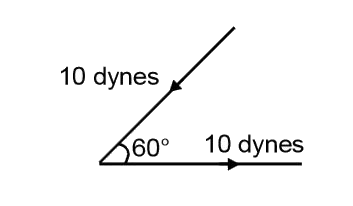 A10 dynesB20 dynesC10√3 dynesD5 dynes
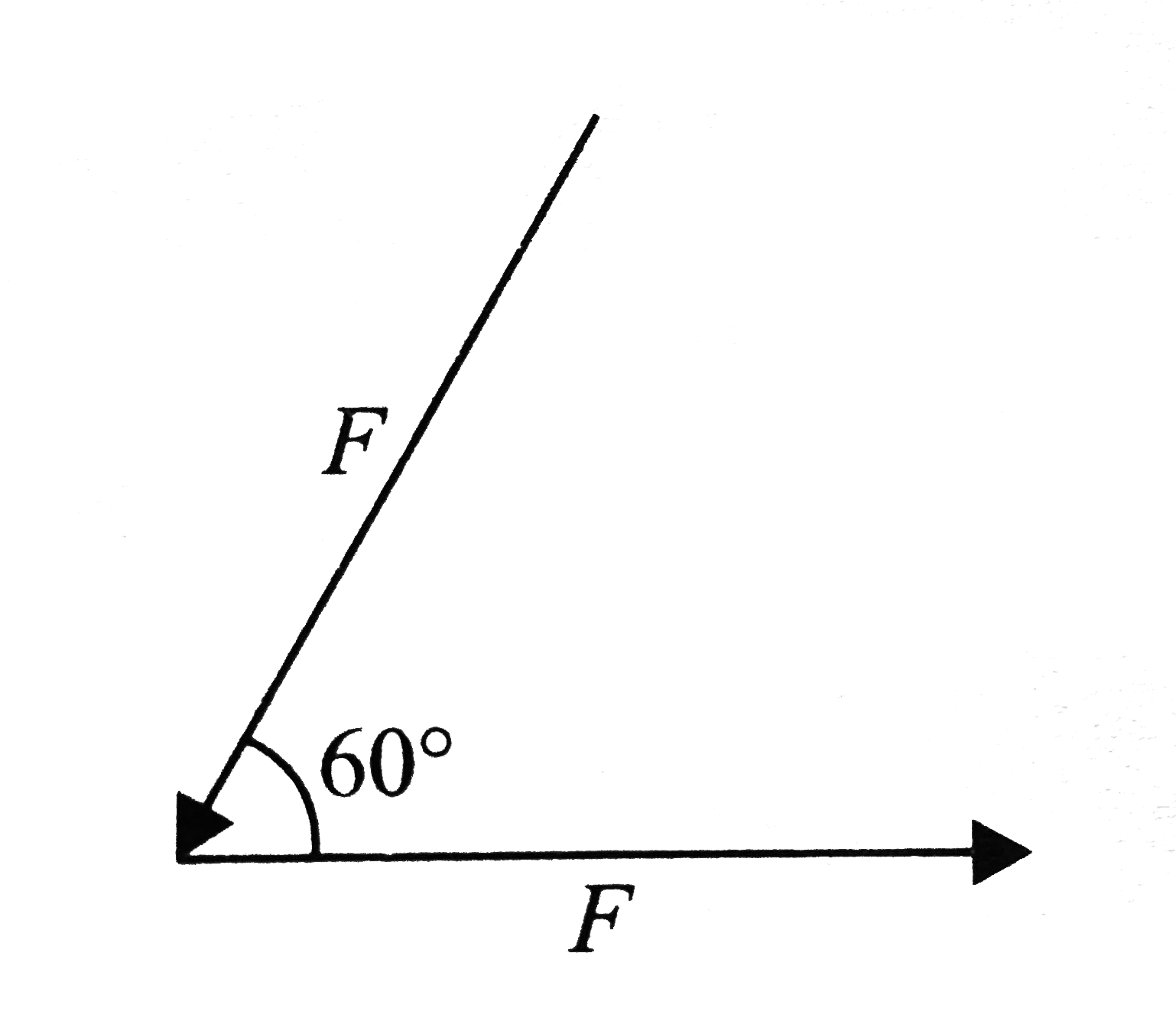
A10 dynesB20 dynesC10√3 dynesD5 dynesTwo forces, each equal to F, act as shown in figure. Their resultant is
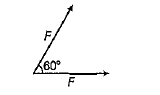 AF/2BFC√3D√5F
AF/2BFC√3D√5F
The forces, each numerically equal to 5 N, are acting as shown in the Figure. Find the angle between forces?
View SolutionFour coplanar forces are applied at a point O. Each of them is equal to k and the angle between consecutive forces equals 45∘ as shown in the figure. Then the resultant has the magnitude equal to:
View SolutionTwo forces, each equal to F, act as shown in (figure) Their resultant is
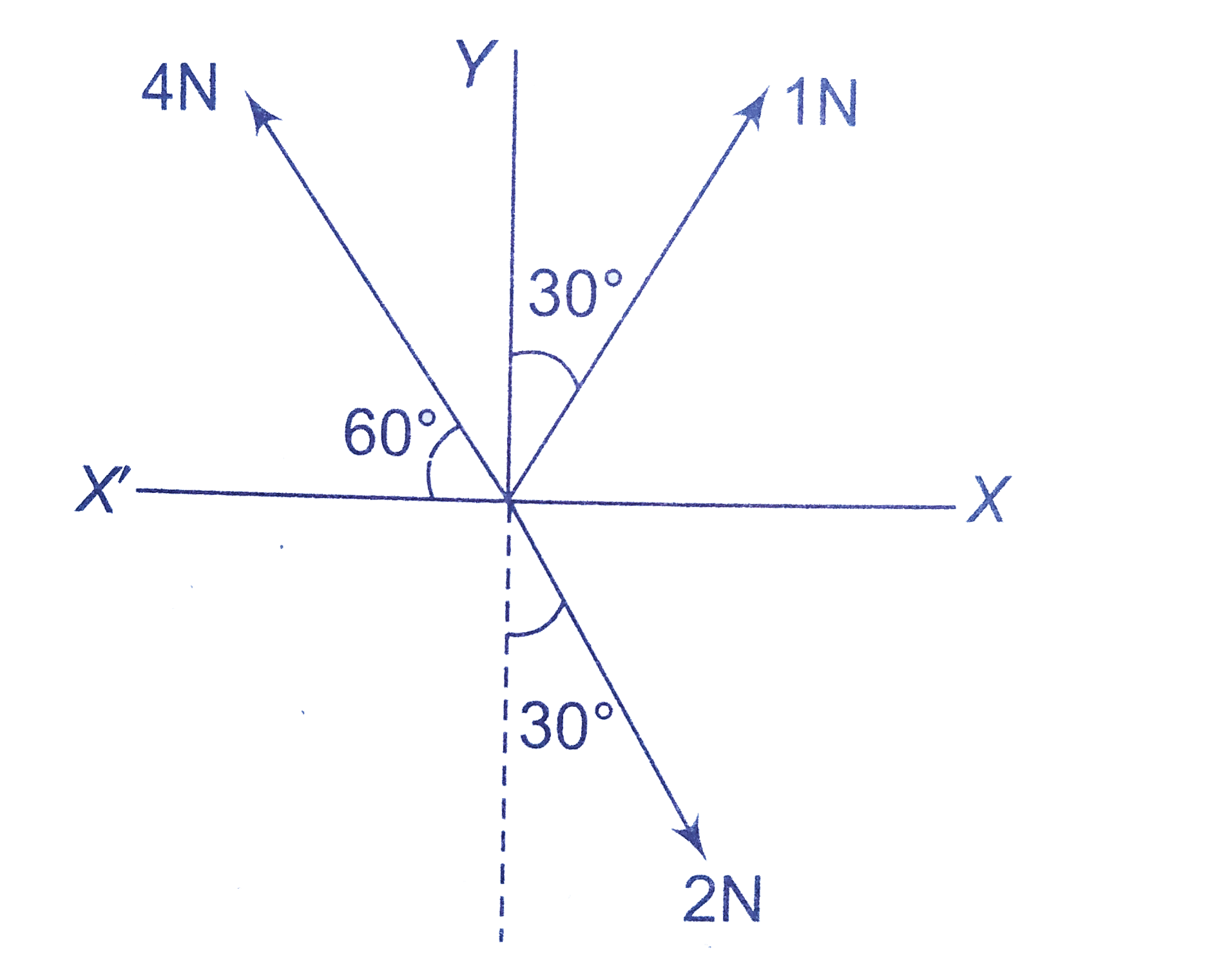
View SolutionThree forces are acting on a particle as shown in the figure. To have the resultant force only along the Y-direction, the magnitude of the maximum additional force needed is
View SolutionIn the given figure O is the centre of regular pentagon ABCDE. Five forces each of magnitude F0 are acted as shown in figure. The resultant force is

View Solution
