Column-IColumn-IlA) Thermal stress,P)3αΔt(100)B) Loss in time of a pendulum clock per sec.Q)d(α2−α1)ΔtC) Percentage in volume of a solidR)YαΔtD) Radius of circular arc of a bimetal stripS)(1/2)αΔtSolution in Telugu
The correct Answer is:A-R; B-S; C-P; D-Q
Step by step video, text & image solution for {:("Column-I","Column-Il"),("A) Thermal stress,","P)" 3 alpha Delta t (100) ),("B) Loss in time of a pendulum clock per sec.","Q)" (d)/( ( alpha_(2) - alpha_(1) ) Delta t) ),("C) Percentage in volume of a solid","R)" Y alpha Delta t ),("D) Radius of circular arc of a bimetal strip","S)" (1//2) alpha Delta t):} by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - EXPANSION OF SOLIDS (LECTURE SHEET) Integer Type Questions5videos
THERMAL PROPERTIES OF MATTER
- BOOK - AAKASH SERIESCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - EXPANSION OF SOLIDS (PRACTICE SHEET) LEVEL-I (Straight Objective Type Questions) Geometry Applications10videos
THERMAL PROPERTIES OF MATTER
- BOOK - AAKASH SERIESCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - EXPANSION OF SOLIDS (LECTURE SHEET) Linked Comprehension Type Questions5videos
THERMAL PROPERTIES OF MATTER
- BOOK - AAKASH SERIESCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - PRACTICE EXERCISE99videos
SYSTEM OF PARTICLES AND ROTATIONAL MOTION
- BOOK - AAKASH SERIESCHAPTER - THERMODYNAMICSEXERCISE - EXERCISE - 333videos
THERMODYNAMICS
Similar Questions
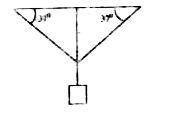
A block is hanged by means of two identical wires having cross-section area A (1mm2) as shown in the figure. If temperature is lowered by ΔT(10∘C), find the mass (in kg) to be added to hanging mass such that junction remains at its initial positions. Given that coefficient of linear expansion α=2×10−5I0C and Young's modulus Y=5×1011N/m2 for the wire.
 04:10View Solution
04:10View SolutionTwo large holes are cut in a metal sheet. If this is heated, distance AB and BC, (as shown)
 10:14View Solution
10:14View SolutionPassage - I:
A rod of length 2m is at a temperature of 20∘C. Find the free expansion of the rod, if the temperature is increased to 50°C, then find stress produced when the rod is
Fully prevented to expand06:49View SolutionThe centre of mass of two particles with masses 2kg and 6 kg located at (1,1,1), (2,2,1) respectively has the coordinates
04:04View SolutionA bullet of mass 10×10−3 moving with a speed of 20ms−1 hits an ice block (0∘C) of 990g kept at rest on a frictionless floor and gets embedded in it. If ice takes 50% of K.E, the amount of ice melted (in grams) approximately is (J=4.2J/cal) (latent heat of ice=80cal/g)
02:46View SolutionLet alpha,beta be the roots of x^(2)-4x+A=0 and gamma,delta be the roots of x^(2)-36x+B=0 .If alpha,beta,gamma,delta form an increasing G.P.and A^(t)=B then value of 't' equals:
04:38View SolutionIf alpha,beta,gamma,delta are four complex numbers such that (gamma)/(delta) is real and alpha delta-beta gamma!=0 then z=(alpha+beta t)/(gamma+delta t) represents a
03:54View Solution(a) The displacement s of a particale in time t related as s = alpha + beta t + gamma t^(2) + delta t^(2) (b) The veloctiy v of particle varies with time as v = alpha t + beta t^(2) + (gamma )/(t + s) Findk the dimension fo alpha, beta, gamma and delta .
07:48View SolutionWhen the two rods having expansion cofficient alpha_(1), alpha_(2) (alpha_(2) gt alpha_(1)) and width d are heated then the radius of the rod after expansion. R=(d)/((alpha_(2)-alpha_(1))Delta T)
14:25View Solution[" 17.The temperature of a thin uniform rod increases by "Delta" .If moment of inertia I "],[" about an axis perpendicular to its length,then its moment of increases by "],[[" 1) "0," 2) "alpha" I "Delta t," 3) "2 alpha l Delta t," 4) "alpha^(2)I Delta t]]
06:44View SolutionShow that the coefficient of area expansion , (Delta A//A)//Delta T , of a rectangular sheet of the solid is twice its linear expansivity , alpha_(1) .
04:28View SolutionShow that the coefficient of area expansion , (ΔA/A)/ΔT , of a rectangular sheet of the solid is twice its linear expansivity , α1.
View SolutionShow that the coefficient of area expansion , (Delta A//A)//Delta T , of a rectangular sheet of the solid is twice its linear expansivity , alpha_(1) .
07:54View SolutionIf α,β,γ,δ are four complex numbers such that γδ is real and αδ−βγ≠0 then z = α+βtγ+δt where t is a rational number, then it represents:
04:54View SolutionIf α,β are the roots of x2+px+q=0 and γ,δ are the roots of x2+px+r=0, then (α−γ)(α−δ)(β−γ)(β−δ)=
(a) 1 (b) q (c) r (d) q+r05:10View Solution
