Show that the coefficient of area expansion , (ΔA/A)/ΔT , of a rectangular sheet of the solid is twice its linear expansivity , α1.Solution in Telugu
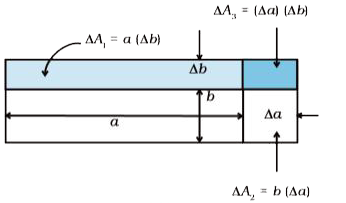
Consider a rectangular sheet of the solid material of length a and breadth b (Fig. 11.8). When the temperature increases by ΔT,a Increases by Δa=α1aΔT and b increases by Δb=α1,bDetlaT. From Fig. 11.8, the increase in area
ΔA=ΔA1+ΔA2+ΔA3
ΔA=aΔb+bΔa+(Δa)(Δb)
=aα1bΔT+bα1aΔT+(α1)2ab(ΔT)2
=α1abΔT(2+α1ΔT)=α1AΔT(2+α1ΔT)
Since α1≈10−5K−1, from Table 11.1 , the product α1ΔT for fractional temperature is small in comparision with 2 and may be neglected.
Hence,
(ΔAA)1ΔT≈2αl
Topper's Solved these Questions
- THERMAL PROPERTIME OF MATTERBOOK - NCERT TELUGUCHAPTER - THERMAL PROPERTIME OF MATTEREXERCISE - EXERCISES
30 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - NCERT TELUGUCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - EXERCISES (TRUE OR FALSE)
5 Videos
- THERMODYNAMICS BOOK - NCERT TELUGUCHAPTER - THERMODYNAMICS EXERCISE - EXERCISES
10 Videos
Similar Questions
The triple points of neon and carbon dioxide are 24.57K and 216.55K respectively. Express these temperatures on the Celsius and Fahrenheit scales.
View SolutionDuring rolling, the force of friction acts in the same direction as the direction of motion of the CM of the body.
View SolutionA geyser heats water flowing at the rate of 3.0 litres per minute from 27°C to 77°C. If the geyser operates on a gas burner, what is the rate of consumption of the fuel if its heat of combustion is 4.0×104J/g ?
View SolutionThe coefficient of linear expansion of crystal in one direction is alpha_(1) and that in every direction perpendicular to it is alpha_(2) . The coefficient of cubical expansion is
View SolutionShow that the coefficeint of area expansions (Delta A//A)//Delta T of a rantangular sheet of the solid is twice its linear expansitivity alpha .
View Solutionयह दर्शाइए कि किसी ठोस की आयताकार शीट का क्षेत्र प्रसार गुणांक, ΔA/AΔT, इसके रैखिक प्रसार गुणांक αl का दो गुना होता है |
View Solution" The relation between coefficient of linear expansion "(alpha_(1))" and coefficient of volume expansion ( "alpha_(5)" ) is "
View SolutionA solid rectangular sheet has two different coefficients of linear expansion alpha_(1) " and " alpha_(2) along its length and breadth respectively. The coefficient of surface expansion is ("for " alpha_(1)t "<<"1, alpha_(2)t"<<"1)
View SolutionShow that the coefficient of superficial expansion of a rectangular sheet of the solid is twice its coefficient of linear expansion.
View SolutionShow that the coefficient of area expansion , (ΔA/A)/ΔT , of a rectangular sheet of the solid is twice its linear expansivity , α1.
View SolutionShow that the coefficient of area expansion , (Delta A//A)//Delta T , of a rectangular sheet of the solid is twice its linear expansivity , alpha_(1) .
View SolutionA solid rectangular sheet has two different coefficients of linear expansion alpha_1 and alpha_2 along its length and breadth respectively. The coefficient of surface expansion is (for alpha_1 t ltlt 1, alpha_2 lt lt 1 )
View SolutionShow that the coefficient of area expansions (ΔA/A)/ΔT of a rectangular sheet of the solid is twice its linear expansivity α.
View SolutionShow that the coefficient of area expansions, (DeltaA//A)//DeltaT , of a rectangular sheet of the solid is twice its linear expansively, alpha_(l).(alpha_(l)=10^(-5)K^(-1))
View SolutionThe coefficient of linear expansion of crystal in one direction is α1 and that in every direction perpendicular to it α2. The coefficient of cubical expansion is :
View Solution
