A vertical lamp-post of height h stands at a point on the boundary of a circular field. A man of height a is running round the boundary. Prove that the end of the shadow of the man will also travel on a circle. Find the ratio of the radii of the two circles.
To solve the problem step by step, we will prove that the end of the shadow of the man travels in a circle and find the ratio of the radii of the two circles.
Step 1: Understanding the Setup
Let:
- H = height of the lamp-post
- A = height of the man
- R = radius of the circular field
- S = length of the shadow of the man
The man is running around the boundary of the circular field, and we need to analyze the geometry of the situation.
Step 2: Establishing Relationships
When the man is at a point on the boundary of the circular field, the light from the lamp-post will cast a shadow. The angle formed by the line from the top of the lamp-post to the end of the shadow and the horizontal line can be denoted as θ.
Using the tangent function, we can express:
tan(θ)=HS
This equation relates the height of the lamp-post to the length of the shadow.
Step 3: Analyzing the Man's Position
When the man is at a distance r from the foot of the lamp-post, the angle θ can also be expressed in terms of the man's height:
tan(θ)=AR+S
Here, R+S is the horizontal distance from the lamp-post to the end of the shadow.
Step 4: Setting Up the Equation
Since both expressions for tan(θ) are equal, we can set them equal to each other:
HS=AR+S
Step 5: Cross-Multiplying
Cross-multiplying gives us:
H(R+S)=AS
Step 6: Rearranging the Equation
Rearranging the equation, we have:
HR+HS=AS
HR=AS−HS
HR=S(A−H)
Thus, we can express S:
S=HRA−H
Step 7: Finding the Ratio of the Radii
The radius of the circle traced by the man is R, and the radius of the circle traced by the end of the shadow is R+S. Therefore, the ratio of the radii is:
Ratio=RR+S
Substituting S from the previous step:
Ratio=RR+HRA−H=RR(1+HA−H)=11+HA−H
This simplifies to:
Ratio=A−HA
Conclusion
Thus, we have proved that the end of the shadow of the man travels in a circle and found the ratio of the radii of the two circles to be:
A−HA
Let:
- H = height of the lamp-post
- A = height of the man
- R = radius of the circular field
- S = length of the shadow of the man
When the man is at a point on the boundary of the circular field, the light from the lamp-post will cast a shadow. The angle formed by the line from the top of the lamp-post to the end of the shadow and the horizontal line can be denoted as θ.
tan(θ)=HS
This equation relates the height of the lamp-post to the length of the shadow.
When the man is at a distance r from the foot of the lamp-post, the angle θ can also be expressed in terms of the man's height:
tan(θ)=AR+S
Here, R+S is the horizontal distance from the lamp-post to the end of the shadow.
Since both expressions for tan(θ) are equal, we can set them equal to each other:
HS=AR+S
Cross-multiplying gives us:
H(R+S)=AS
Rearranging the equation, we have:
HR+HS=AS
HR=AS−HS
HR=S(A−H)
Thus, we can express S:
S=HRA−H
The radius of the circle traced by the man is R, and the radius of the circle traced by the end of the shadow is R+S. Therefore, the ratio of the radii is:
Ratio=RR+S
Substituting S from the previous step:
Ratio=RR+HRA−H=RR(1+HA−H)=11+HA−H
This simplifies to:
Ratio=A−HA
Thus, we have proved that the end of the shadow of the man travels in a circle and found the ratio of the radii of the two circles to be:
A−HA
Topper's Solved these Questions
Similar Questions
A flagstaff stands vertically on a pillar, the height of the flagstaff being double the height of the pillar. A man on the ground at a distance finds that both the pillar and the flagstaff subtend equal angles at his eyes. The ratio of the height of the pillar and the distance of the man from the pillar is
View SolutionA man of height 1.7 m walks at a uniform speed of 6.6 m/min from a lamp post which is 5m high.Find the rate at which the length of his shadow increases.
View Solution
Knowledge Check
A man of height h walks in a straight path towards a lamp post of height H with uniform velocity u. Then , the velocity of the edge of the shadow on the ground will be
AhuH−hBHuH+hCH−hHuDH+hHuA man of height h walks in a straight path towards a lamp post of height H with uniform velocity u. Then , the velocity of the edge of the shadow on the ground will be
AhuH−hBHuH+hCH−hHuDH+hHuman of height h walks in a straight path towards a lamp post of height H with uniform velocity u. Then the velocity of the edge of the shadow on the ground will be :-
AHu(H−h)BHu(H+h)C(H−h)HuD(H+h)Hu
A vertical pole stands at a point A on the boundary of a circular park of radius a and subtends an angle α at another point β on the boundary. If the chord AB subtends an angle α at the centre of the park, the height of the pole is
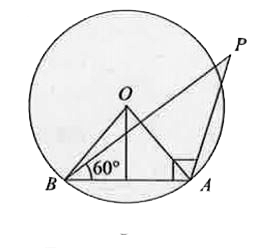
View SolutionA vertical pole stands at a point A on the boundary of a circular park of radius 2 km and subtends an angle 60∘ at another point B on the boundary. If the chord AB subtends the same angle 60∘ at the centre of the park, the height of the pole is
View SolutionA pole of height h stands in the centre of a circular platform in the centre of a circular field. Another pole of equal height is at a point on the boundary of the field. The angles of elevation of the top of the first pole from the bottom and top of the second pole are respectively αandβ. Height of the platform from the ground is
View SolutionA tower stands at the centre of a circular park. A and B are two points on the boundary of the park such that AB(=alpha) subtends an angle of elevation of the top of the first tower from A or B is 30∘. The height of the tower is
View SolutionA tower stands at the centre of a circular park . A and B are two points on the boundary of the park such that AB(=a) subtends an angle of 60∘ at the foot of the tower , and the angle of elevation of the top of the tower from A or B is 30∘. The height of the tower is
View Solution
