Two circular, similar, coaxial loops carry equal currents in the same direction. If the loops are brought nearer, what will happen?
P increases while in Q decreases
Q increases while in P decreases
both P and Q increases
both P and Q decreases
The correct Answer is:D
To solve the question regarding the behavior of two circular, similar, coaxial loops carrying equal currents in the same direction when brought closer together, we can follow these steps:
1. Understanding the Setup:
- We have two circular loops (let's call them Loop A and Loop B) that are coaxial, meaning they share the same center axis. Both loops are carrying equal currents in the same direction.
2. Magnetic Field Due to Each Loop:
- Each loop generates a magnetic field around it due to the current flowing through it. The direction of the magnetic field can be determined using the right-hand rule.
3. Bringing the Loops Closer:
- When Loop B is brought closer to Loop A, the magnetic field produced by Loop B will influence the magnetic flux through Loop A and vice versa.
4. Change in Magnetic Flux:
- As Loop B approaches Loop A, the magnetic flux linked with Loop A increases because the magnetic field from Loop B is stronger at closer distances.
5. Induction of Current:
- According to Faraday's law of electromagnetic induction, a change in magnetic flux through a loop induces an electromotive force (EMF) in that loop. Therefore, the increasing magnetic flux through Loop A will induce a current in Loop A.
6. Direction of Induced Current:
- The direction of the induced current will be such that it opposes the change in magnetic flux, in accordance with Lenz's Law. Since the magnetic flux is increasing, the induced current will flow in a direction that creates a magnetic field opposing the increase.
7. Effect on Current in Each Loop:
- The induced current in Loop A will oppose the original current flowing in Loop A, leading to a decrease in the net current in Loop A. Similarly, Loop B will experience an induced current that opposes its original current, leading to a decrease in the net current in Loop B as well.
8. Conclusion:
- When the two loops are brought closer together, the current in each loop decreases due to the induced currents opposing the original currents, as per Lenz's Law.
Final Answer:
When two circular, similar, coaxial loops carrying equal currents in the same direction are brought nearer, the current in each loop will decrease.
- We have two circular loops (let's call them Loop A and Loop B) that are coaxial, meaning they share the same center axis. Both loops are carrying equal currents in the same direction.
- Each loop generates a magnetic field around it due to the current flowing through it. The direction of the magnetic field can be determined using the right-hand rule.
- When Loop B is brought closer to Loop A, the magnetic field produced by Loop B will influence the magnetic flux through Loop A and vice versa.
- As Loop B approaches Loop A, the magnetic flux linked with Loop A increases because the magnetic field from Loop B is stronger at closer distances.
- According to Faraday's law of electromagnetic induction, a change in magnetic flux through a loop induces an electromotive force (EMF) in that loop. Therefore, the increasing magnetic flux through Loop A will induce a current in Loop A.
- The direction of the induced current will be such that it opposes the change in magnetic flux, in accordance with Lenz's Law. Since the magnetic flux is increasing, the induced current will flow in a direction that creates a magnetic field opposing the increase.
- The induced current in Loop A will oppose the original current flowing in Loop A, leading to a decrease in the net current in Loop A. Similarly, Loop B will experience an induced current that opposes its original current, leading to a decrease in the net current in Loop B as well.
- When the two loops are brought closer together, the current in each loop decreases due to the induced currents opposing the original currents, as per Lenz's Law.
When two circular, similar, coaxial loops carrying equal currents in the same direction are brought nearer, the current in each loop will decrease.
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXEMPLAR PROBLEMS
3 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - ASSERTION AND REASON
15 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Assertion And Reason
15 Videos
- ELECTROMAGNETIC WAVESBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Assertion And Reason
15 Videos
Similar Questions
Two identical coaxial circular loops carry a current i each circulating int the same direction. If the loops approch each other the current in
View SolutionTwo identical coils are placed coaxially. They carry equal currents in same direction
NOTE in parts (a), (b) and (c) exclude the points at infinity.
View Solution
Knowledge Check
Two identical current carrying coaxial loops, carry current I in an opposite sense. A simple amperian loop passes through both of them once, calling the loop as C, which of the option is correct ?
A∮c→B.→dl=±2μ0JBthe value of ∮→B.→dl is independent of sense of C.Cthere may be a point on C where B and dl are parallel.Dnone of these
(a) Two straight long parallel conductors carry currents I1 and I2 in the same direction. Deduce the expression for the firce per unit length between them.
Depict the pattern of magnetic field lines around them.
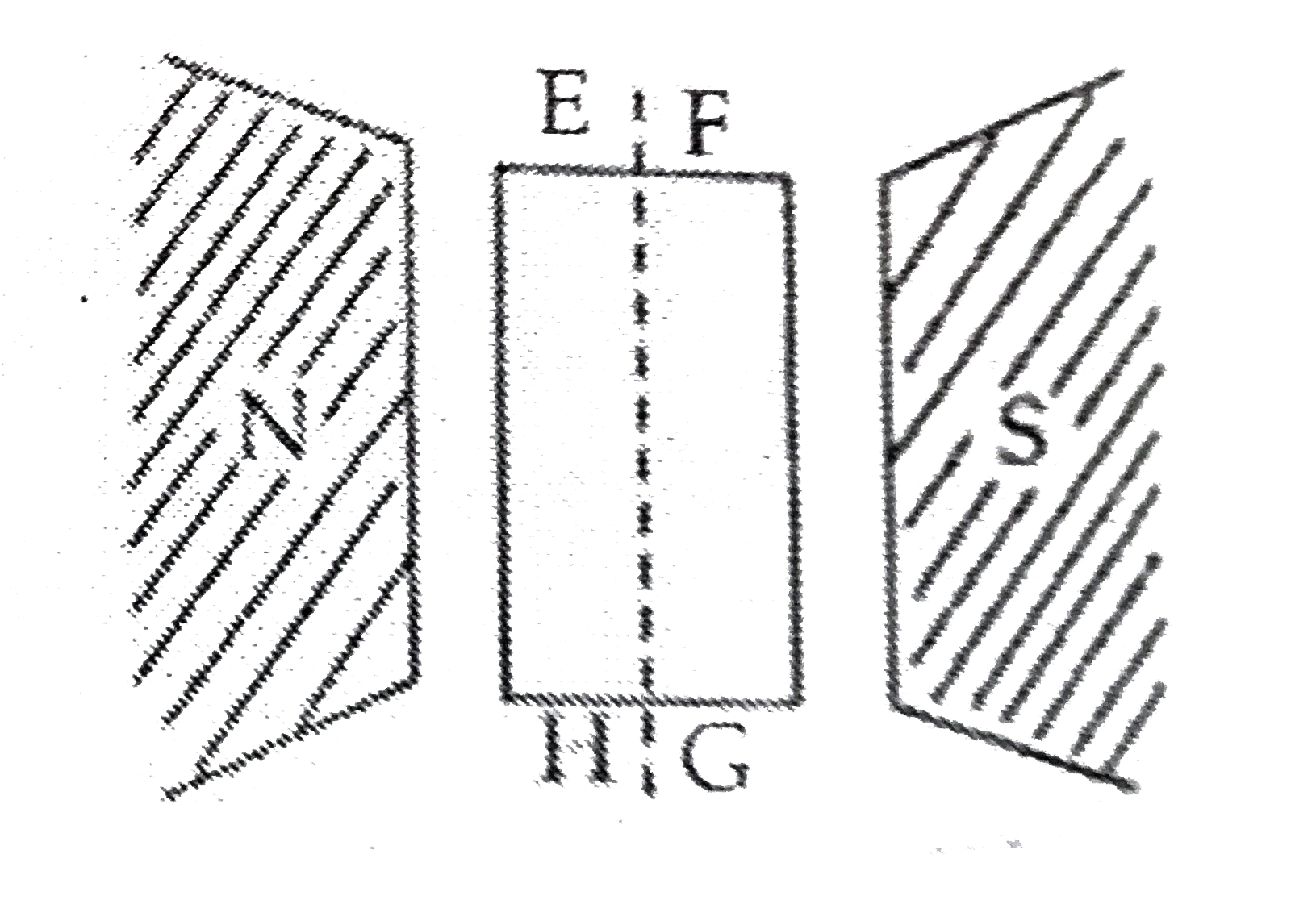
(b) A rectangular current carrying loop EFGH is kept in a uniform magnetic field as shown in the figure.
(i) What is the direction of the magnetic moment of the current loop ?
(ii) What is the torque acting on the loop (A) maximum, (B) zero ?
View SolutionA wire of length 'I' is bent into a circular loop of radius R and carries a current I. The magnetic field at the centre of the loop is 'B '. The same wire is now bent into a double loop. If both loops carry the same current I and it is in the same direction, the magnetic field at the centre of the double loop will be
View SolutionTwo identical current carrying coaxial loops, carry current I in an opposite sense. A simple amperian loop passes through both of them once. Calling the loop as C,
View SolutionA current carrying circular coil is bent so as to convert it into a double loop, both the loops being concentric and are carrying current in the same direction. If B is the initial magnetic field at the centre, the final magnetic field at the centre will be
View SolutionA circular loop carrying a current is replaced by an equivalent magnetic dipole. A point on the axis of the loop is in
View SolutionA square loop of side a carris a current I. The magnetic field at the centre of the loop is
View SolutionTwo circular loops lie side by side in the same plane. One is connected to a source that supplies an increasing current, the other is a simple closed ring. Is the induced current in the ring is in the same direction as that in the loop connected to the source or opposite? What if the current in the first loop is decreasing?
View Solution
