There is a solid sphere of radius R having uniformly distributed charge throughout it. What is the relation between electric field E and distance r from the centre (r is less than R) ?
E∝r−2
E∝r−1
E∝r
E∝r2
The correct Answer is:C
To find the relation between the electric field E and the distance r from the center of a uniformly charged solid sphere (where r<R), we can follow these steps:
1. Understand the Setup:
We have a solid sphere of radius R with a uniform charge distribution. We want to find the electric field E at a distance r from the center of the sphere, where r<R.
2. Use Gauss's Law:
According to Gauss's Law, the electric flux through a closed surface is equal to the charge enclosed by that surface divided by the permittivity of free space ϵ0:
ΦE=Qencϵ0
where ΦE=∮→E⋅d→A.
3. Choose a Gaussian Surface:
We choose a Gaussian surface that is a sphere of radius r (where r<R). The electric field E is uniform over this surface and directed radially outward.
4. Calculate the Electric Flux:
The electric flux through the Gaussian surface is:
ΦE=E⋅4πr2
where 4πr2 is the surface area of the sphere.
5. Determine the Charge Enclosed:
The charge enclosed Qenc within the Gaussian surface can be calculated using the charge density ρ:
Qenc=ρ⋅Venc=ρ⋅43πr3
where Venc is the volume of the sphere of radius r.
6. Relate Charge Density to Total Charge:
The charge density ρ can be expressed in terms of the total charge Q of the sphere:
ρ=Q43πR3
7. Substitute Charge Enclosed into Gauss's Law:
Substitute Qenc into Gauss's Law:
E⋅4πr2=ρ⋅43πr3ϵ0
8. Simplify the Equation:
Substitute ρ from step 6:
E⋅4πr2=(Q43πR3)⋅43πr3ϵ0
This simplifies to:
E⋅4πr2=Q⋅r33ϵ0R3
9. Solve for Electric Field E:
Rearranging gives:
E=Q⋅r4πϵ0R3
10. Conclusion:
The electric field E inside a uniformly charged solid sphere (for r<R) is directly proportional to the distance r from the center:
E∝r
We have a solid sphere of radius R with a uniform charge distribution. We want to find the electric field E at a distance r from the center of the sphere, where r<R.
According to Gauss's Law, the electric flux through a closed surface is equal to the charge enclosed by that surface divided by the permittivity of free space ϵ0:
ΦE=Qencϵ0
where ΦE=∮→E⋅d→A.
We choose a Gaussian surface that is a sphere of radius r (where r<R). The electric field E is uniform over this surface and directed radially outward.
The electric flux through the Gaussian surface is:
ΦE=E⋅4πr2
where 4πr2 is the surface area of the sphere.
The charge enclosed Qenc within the Gaussian surface can be calculated using the charge density ρ:
Qenc=ρ⋅Venc=ρ⋅43πr3
where Venc is the volume of the sphere of radius r.
The charge density ρ can be expressed in terms of the total charge Q of the sphere:
ρ=Q43πR3
Substitute Qenc into Gauss's Law:
E⋅4πr2=ρ⋅43πr3ϵ0
Substitute ρ from step 6:
E⋅4πr2=(Q43πR3)⋅43πr3ϵ0
This simplifies to:
E⋅4πr2=Q⋅r33ϵ0R3
Rearranging gives:
E=Q⋅r4πϵ0R3
The electric field E inside a uniformly charged solid sphere (for r<R) is directly proportional to the distance r from the center:
E∝r
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - ASSERTION & REASON
15 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Electric Charges
5 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - NCERT FINGERTPS ENGLISHCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - Assertion And Reason
15 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - NCERT Exemplar
6 Videos
Similar Questions
A spherical shell of radius R has a uniformly distributed charge ,then electric field varies as
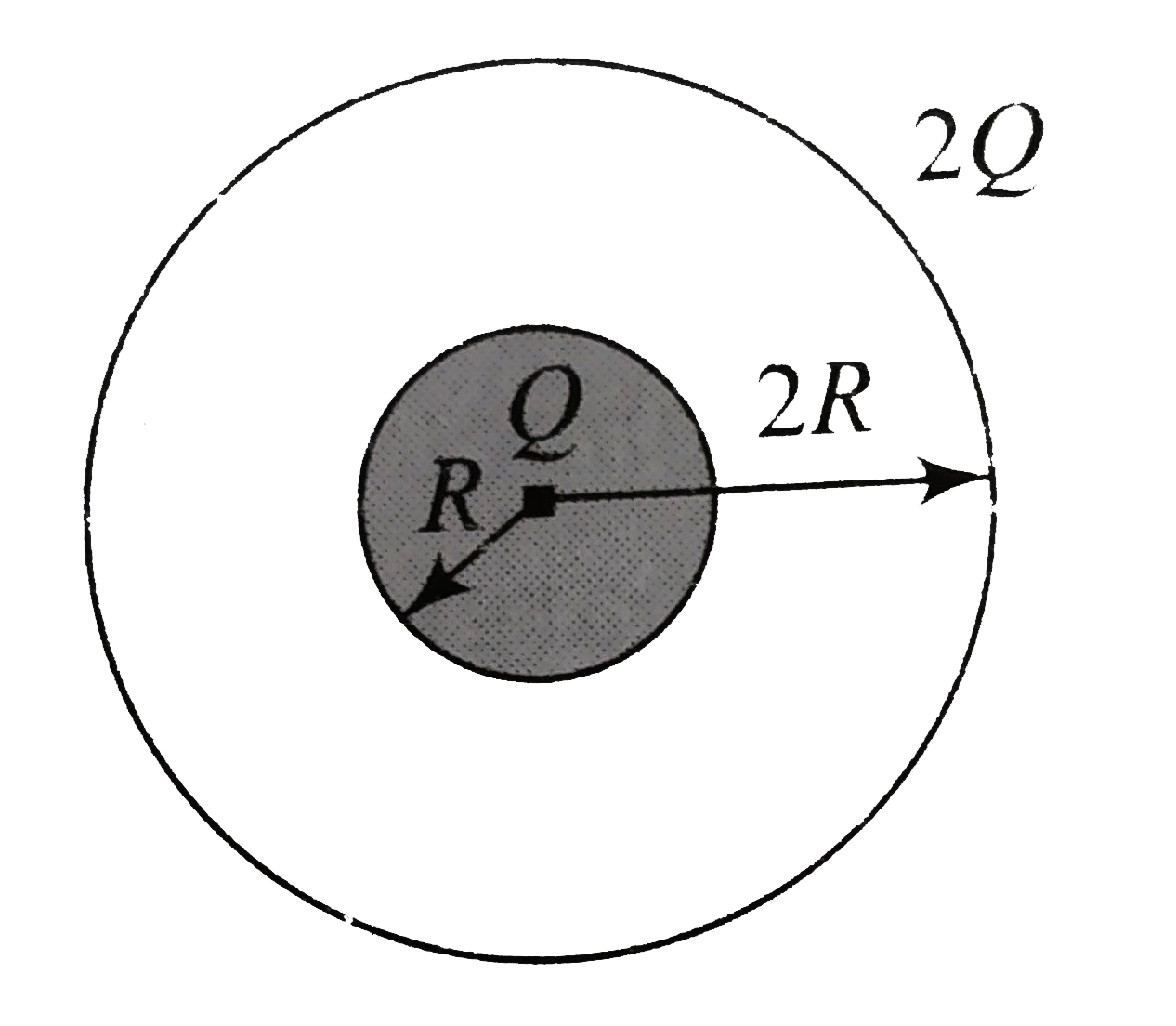
View SolutionA charge Q is uniformly distributed in a dielectric sphere of radius R (having dielectric constant unity). This dielectric sphere is enclosed by a concentric spherical shell of radius 2R and having uniformly distributed charge 2Q. Which of the following graph correctly represents variation of electric field with distance r from the common centre?
View SolutionAn isolated solid metal sphere of radius R is given an electric charge. The variation of the intensity of the electric field with the distance r from the centre of the sphere is best shown by
View SolutionA non-conducting solid sphere of radius R is uniformly charged. The magnitude of the electric filed due to the sphere at a distance r from its centre
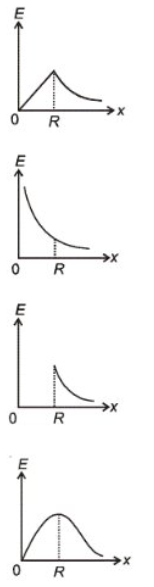
View SolutionA non-conducting solid sphere of radius R, has a uniform charge density. The graph representing variation of magnitude of electric field (E) as a function of distance (x) from the centre of the sphere is
View SolutionA conducting shell of radius R carries charge −Q. A point charge +Q is placed at the centre. The electric field E varies with distance r (from the centre of the shell) as
View SolutionFigure shows two concentric shells of radii a and b uniformly distributed charges q and -q. Which of the following graphs gives correct variation of electric field intensity E with the distance r from the centre?

View SolutionIf the potential at the centre of a uniformly charged hollow sphere of radius R is V, then electric field at a distance r from the centre of sphere will be (r>R).

View SolutionA sphere of radius R carries charge such that its volume charge density is proportional to the square of the distance from the centre. What is the ratio of the magnitude of the electric field at a distance 2 R from the centre to the magnitude of the electric field at a distance of R/2 from the centre?
View SolutionConsider a sphere of radius R having charge q uniformly distributed insider it. At what minimum distance from surface the electric potential is half of the electric potential at its centre?
View Solution
