Two parallel infinite line charges +λand−λ are placed with a separation distance R in free space. The net electric field exactly mid-way between the two line charges is
zero
2λπε0R
λπε0R
λ2πε0R
The correct Answer is:B
To solve the problem of finding the net electric field exactly midway between two parallel infinite line charges with charge densities +λ and −λ separated by a distance R, we can follow these steps:
Step 1: Understand the Configuration
We have two infinite line charges:
- The first line charge has a linear charge density of +λ.
- The second line charge has a linear charge density of −λ.
- The distance between the two line charges is R.
Step 2: Identify the Midpoint
The midpoint between the two line charges is located at a distance of R2 from each line charge.
Step 3: Calculate the Electric Field Due to Each Line Charge
The electric field E due to an infinite line charge with linear charge density λ at a distance r from the line charge is given by the formula:
E=λ2πϵ0r
Electric Field Due to the Positive Line Charge
At the midpoint (distance R2):
E+λ=λ2πϵ0(R2)=λ2πϵ0⋅2R=2λ2πϵ0R
Electric Field Due to the Negative Line Charge
Similarly, for the negative line charge:
E−λ=−λ2πϵ0(R2)=−λ2πϵ0⋅2R=−2λ2πϵ0R
Step 4: Determine the Direction of the Electric Fields
- The electric field due to the positive line charge E+λ points away from the line charge (to the right).
- The electric field due to the negative line charge E−λ points towards the line charge (also to the right).
Step 5: Calculate the Net Electric Field
Since both electric fields point in the same direction (to the right), we can add their magnitudes:
Enet=E+λ+|E−λ|
Enet=2λ2πϵ0R+2λ2πϵ0R=4λ2πϵ0R
Enet=2λπϵ0R
Final Answer
The net electric field exactly midway between the two line charges is:
Enet=2λπϵ0R
We have two infinite line charges:
- The first line charge has a linear charge density of +λ.
- The second line charge has a linear charge density of −λ.
- The distance between the two line charges is R.
The midpoint between the two line charges is located at a distance of R2 from each line charge.
The electric field E due to an infinite line charge with linear charge density λ at a distance r from the line charge is given by the formula:
E=λ2πϵ0r
At the midpoint (distance R2):
E+λ=λ2πϵ0(R2)=λ2πϵ0⋅2R=2λ2πϵ0R
Similarly, for the negative line charge:
E−λ=−λ2πϵ0(R2)=−λ2πϵ0⋅2R=−2λ2πϵ0R
- The electric field due to the positive line charge E+λ points away from the line charge (to the right).
- The electric field due to the negative line charge E−λ points towards the line charge (also to the right).
Since both electric fields point in the same direction (to the right), we can add their magnitudes:
Enet=E+λ+|E−λ|
Enet=2λ2πϵ0R+2λ2πϵ0R=4λ2πϵ0R
Enet=2λπϵ0R
The net electric field exactly midway between the two line charges is:
Enet=2λπϵ0R
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - ASSERTION & REASON
15 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Electric Charges
5 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - NCERT FINGERTPS ENGLISHCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - Assertion And Reason
15 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - NCERT Exemplar
6 Videos
Similar Questions
Two parallel infinite line charges +λand−λ are placed with a separation distance R in free space. The net electric field exactly mid-way between the two line charges is
View SolutionTwo parallel infinite line charges with linear charge densities +λC/m and −λ C/m are placed at a distance of 2R in free space. What is the electric field mid-way between the two line charges?
View SolutionTwo charges +5μC and +10μC are placed 20cm apart. The net electric field at the mid-point between the two charges is
View SolutionTwo infinitely long thin straight wires having uniform linear charge densities λ and 2λ are arranged parallel to each other at a distance r apart.The intensity of the electric field at a point midway between them is
View SolutionTwo charges e and 3e are placed at a distance r. The distance of the point where the electric field intensity will be zero is
View SolutionTwo point charges +4q and +q are placed 30cm apart. At what point on the line joining them is the electric field zero
View SolutionTwo point electric charges of unknown magnitudes and signs are placed a certain distance apart. The electric field intensity is zero at a point not between the charges but on the line joining them. Write two essential conditions for this to happen.
View SolutionTwo point charges +Q and -Q are separated by a certain distance. The resultant electric field is parallel to the line joining the charges at the points
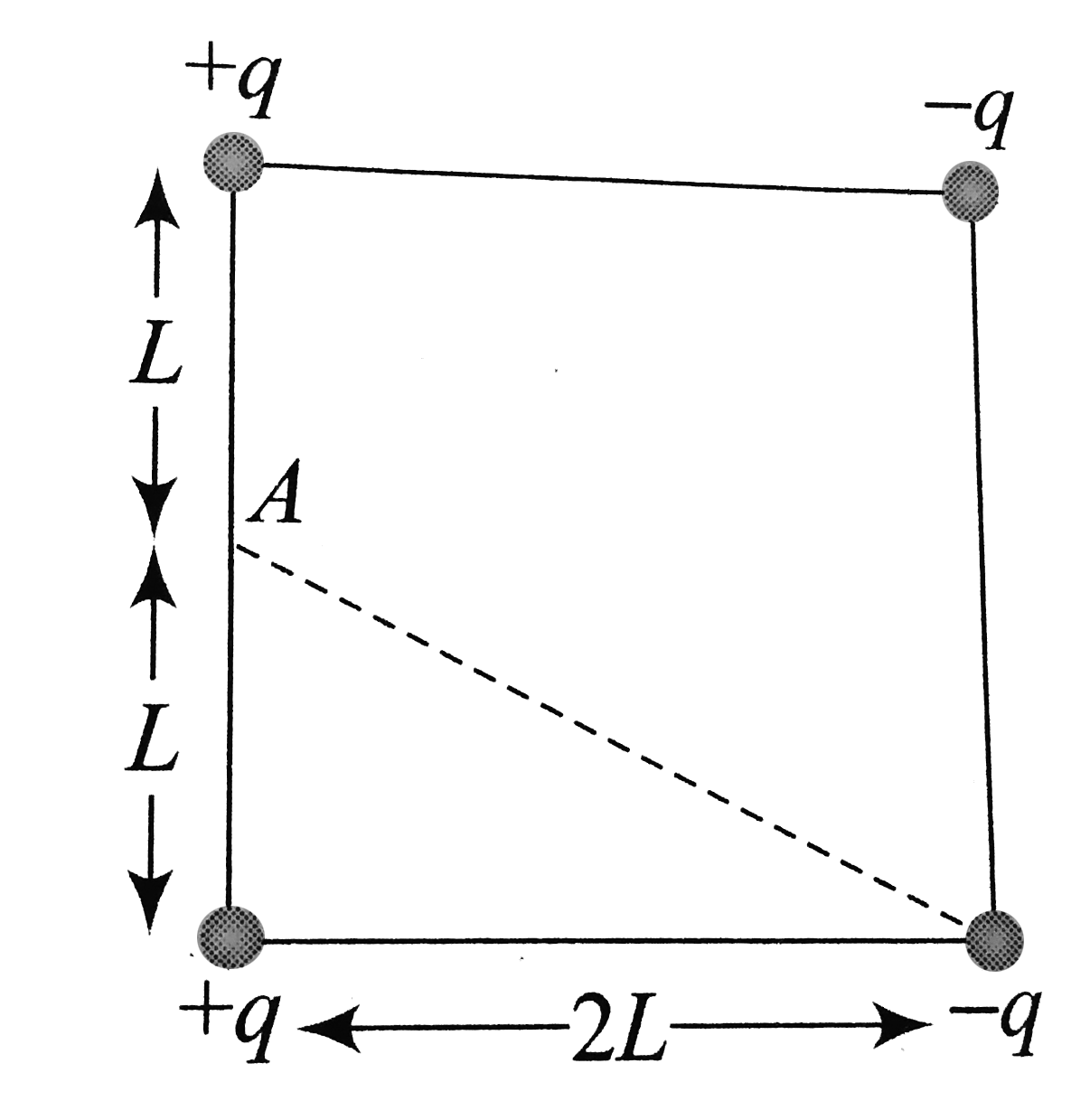
View SolutionFour electric charges +q,+q,−q and −q are placed at the corners of a square of side 2L (see figure). The electric potential at point A, mid-way between the two charges +q and +q, is
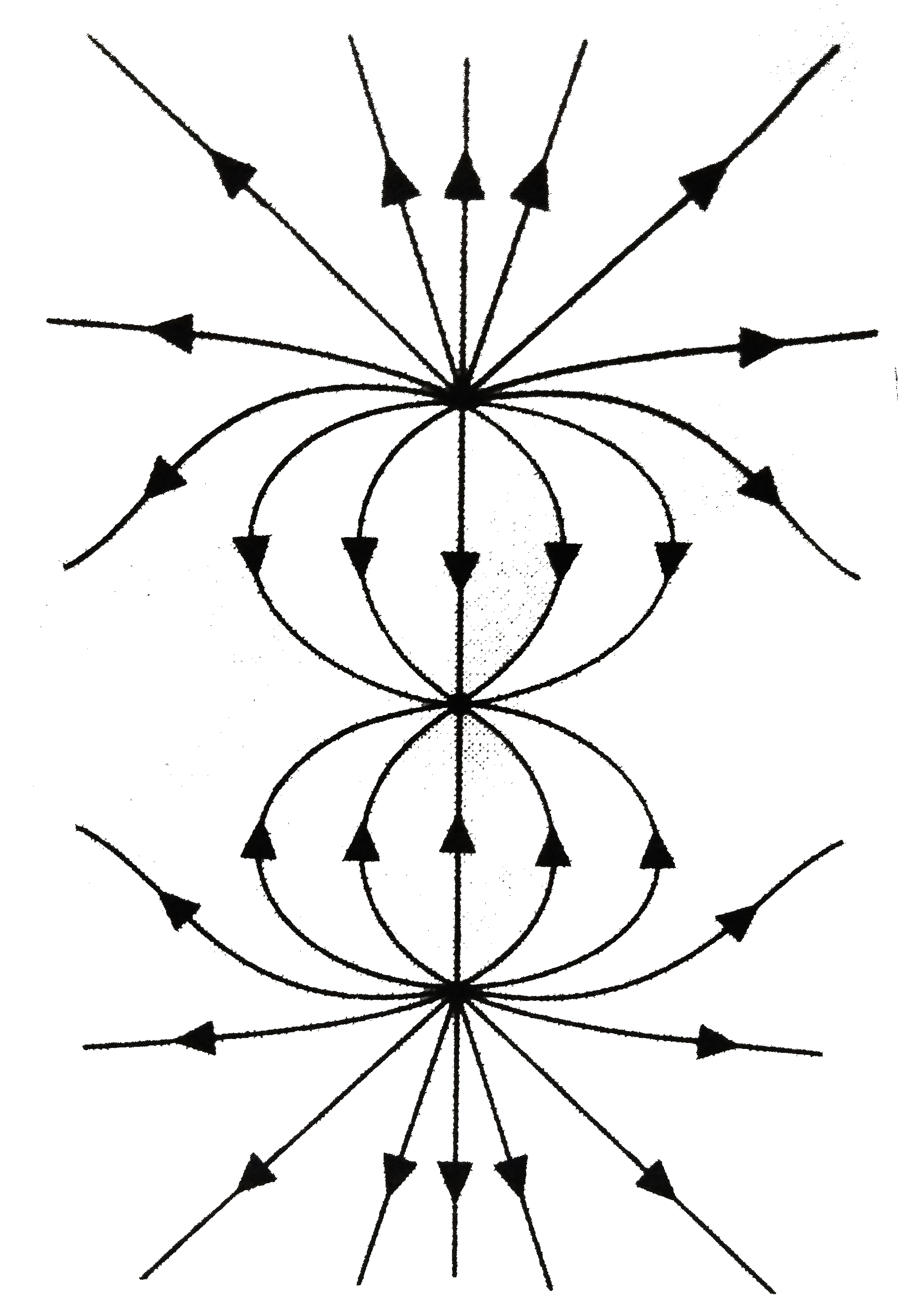
View SolutionFig. shows some of the electric field lines due to three point charges arranged along the vertical axis. All three charges have the same magnitude.
(a) What are the signs of each of the three charges? Explain your reasoning.
(b) At what points(s) is the magnitude of the electric field the smallest? Explain your reasoning. Explain how the fields produced by each individual point charge combine to give a small net field at this point or points.
(c ) Two point charges q and -q are placed at a distance d apart. What are the points at which the resultant electric field is parallel to the line joining the two charge?
 .
.
View Solution
