An ideal gas undergoes isothermal process from some initial state i to final state f. Choose the correct alternatives.
1.dU=dQ
2. dU=-dW
3. dU=0
4. dU=dW
The correct Answer is:C
To solve the question regarding an ideal gas undergoing an isothermal process from an initial state i to a final state f, we will analyze the situation using the first law of thermodynamics and the properties of isothermal processes.
1. Understanding Isothermal Process:
- An isothermal process is defined as a thermodynamic process that occurs at a constant temperature. For an ideal gas, this means that the internal energy of the gas does not change during the process.
2. Applying the First Law of Thermodynamics:
- The first law of thermodynamics is given by the equation:
dQ=dU+dW
where dQ is the heat added to the system, dU is the change in internal energy, and dW is the work done by the system.
3. Change in Internal Energy:
- The change in internal energy dU for an ideal gas can be expressed as:
dU=mCvΔT
where m is the mass of the gas, Cv is the specific heat at constant volume, and ΔT is the change in temperature.
4. Temperature Change in Isothermal Process:
- Since the process is isothermal, the temperature remains constant. Therefore, the change in temperature ΔT is zero:
ΔT=Tf−Ti=0
This implies:
dU=mCv⋅0=0
5. Conclusion on Internal Energy:
- Since dU=0, we can substitute this back into the first law of thermodynamics:
dQ=0+dW⇒dQ=dW
This means that the heat added to the system is equal to the work done by the system.
6. Evaluating the Options:
- Now, we can evaluate the given options:
- Option 1: dU=dQ → Incorrect, since dU=0.
- Option 2: dU=−dW → Incorrect, since dU=0.
- Option 3: dU=0 → Correct, as derived above.
Final Answer:
The correct alternative is that dU=0.
- An isothermal process is defined as a thermodynamic process that occurs at a constant temperature. For an ideal gas, this means that the internal energy of the gas does not change during the process.
- The first law of thermodynamics is given by the equation:
dQ=dU+dW
where dQ is the heat added to the system, dU is the change in internal energy, and dW is the work done by the system.
- The change in internal energy dU for an ideal gas can be expressed as:
dU=mCvΔT
where m is the mass of the gas, Cv is the specific heat at constant volume, and ΔT is the change in temperature.
- Since the process is isothermal, the temperature remains constant. Therefore, the change in temperature ΔT is zero:
ΔT=Tf−Ti=0
This implies:
dU=mCv⋅0=0
- Since dU=0, we can substitute this back into the first law of thermodynamics:
dQ=0+dW⇒dQ=dW
This means that the heat added to the system is equal to the work done by the system.
- Now, we can evaluate the given options:
- Option 1: dU=dQ → Incorrect, since dU=0.
- Option 2: dU=−dW → Incorrect, since dU=0.
- Option 3: dU=0 → Correct, as derived above.
The correct alternative is that dU=0.
Topper's Solved these Questions
- THERMODYNAMICSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - HOTs
8 Videos
- THERMODYNAMICSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - Assertion & Reason
15 Videos
- THERMAL PROPERTIES OF MATTERBOOK - NCERT FINGERTPS ENGLISHCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Assertion And Reason
10 Videos
- UNITS AND MEASUREMENTSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - UNITS AND MEASUREMENTSEXERCISE - Assertion And Reason
15 Videos
Similar Questions
Knowledge Check
An ideal gas undergoes for different processes from the same initial state (figure). Four processes are adiabatic, isothermal, isobaric and isochoric. Out of 1, 2, 3 and 4 which one is adiabatic ?
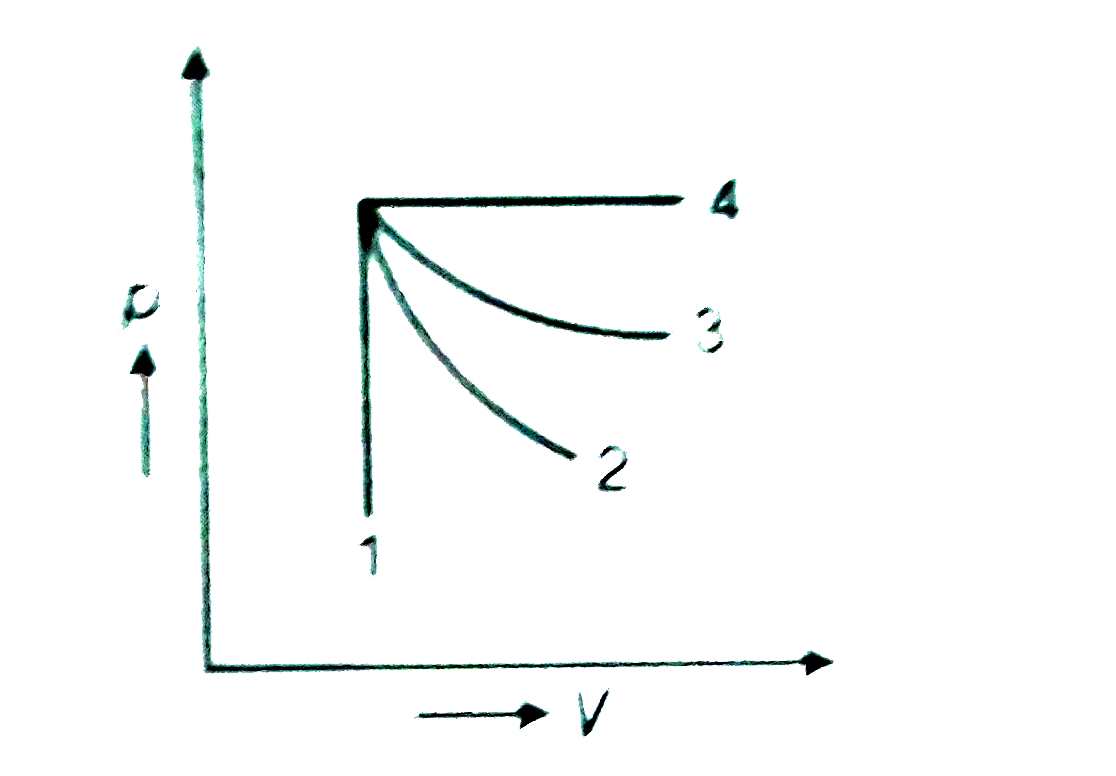 A4B3C2D1
A4B3C2D1An ideal gas system undergoes an isothermal process, then the work done during the process is
AnRTln(V2V1)BnRTln(V1V2)C2nRTln(V2V1)D2nRTln(V1V2)
For an ideal gas undergoing isothermal reversible expansion
View SolutionAn ideal gas undergoes for different processes from the same initial state (figure). Four processes are adiabatic, isothermal, isobaric and isochoric. Out of 1, 2, 3 and 4 which one is adiabatic ?

View SolutionAn ideal gas undergoes isothermal expansion at constant pressure . During the process.
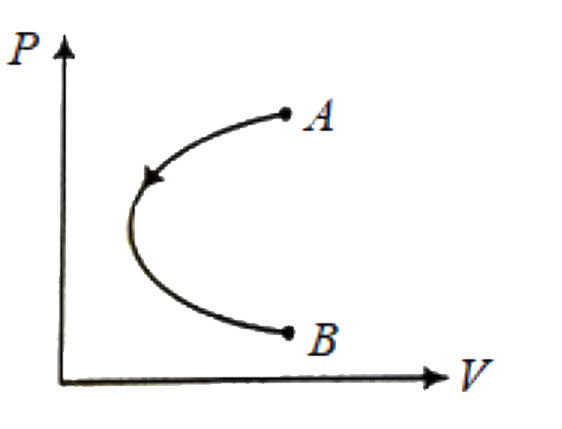
View SolutionP - V diagram of an ideal gas is shown. The gas undergoes from initial state A to final state B such that initial and final volumes are same. Select the correct alternative for given process AB.
View SolutionAn ideal gas is taken from an initial state i to a final state f in such a way that the ratio of the pressure to the absolute temperature remains constant. What will be the work done by the gas ?
View SolutionFor an ideal gas, consider only P-V work in going from an initial state X to the final state Z. The final state Z can be reached by either of the two paths shown in the figure. Which of the following choice(s) is(are) correct? [Take ΔS as change in entropy and w as work done]

View Solution
