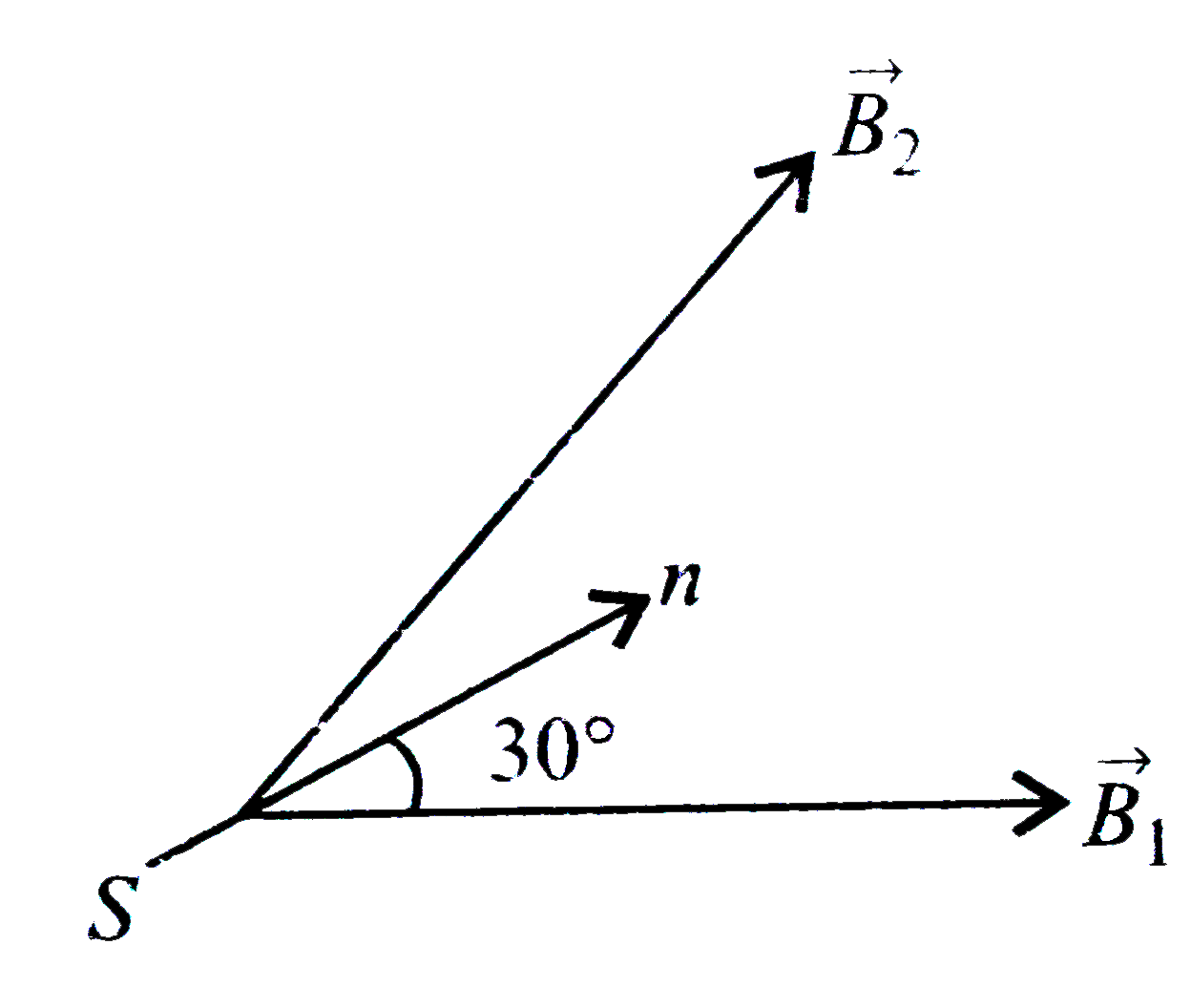
A magnetic dipole is under the influence of two magnetic fields. The angle between the field direction is 60∘ and one of the fields has magnitude of 1.2×10−2T. If the dipole comes to stable equilibrium at an angle of 30∘ with this Held, then the magnitude of the field is
1.2×10−4T
2.4×10−4T
1.2×10−2T
2.4×10−2T
The correct Answer is:C
Here,θ=60∘,B1=1.2×10−2T
θ1=30∘andθ2=60∘−30∘=30∘
in stable equilibrium, torques due to two fields myst be balanced, i.e. τ1=τ2
⇒MB1sinθ1=MB2sinθ2
orB2−B1sinθ1sinθ2
orB2sin30∘sin30∘=B1
=1.2×10−2T
Related Playlists
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - MAGNETISM AND GAUSS S LAW2videos
MAGNETISM AND MATTER
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - THE EARTH S MAGNETISM19videos
MAGNETISM AND MATTER
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - Assertion And Reason15videos
ELECTROSTATIC POTENTIAL AND CAPACITANCE
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MOVING CHARGES AND MAGNETISM EXERCISE - Assertion And Reason15videos
MOVING CHARGES AND MAGNETISM
Similar Questions
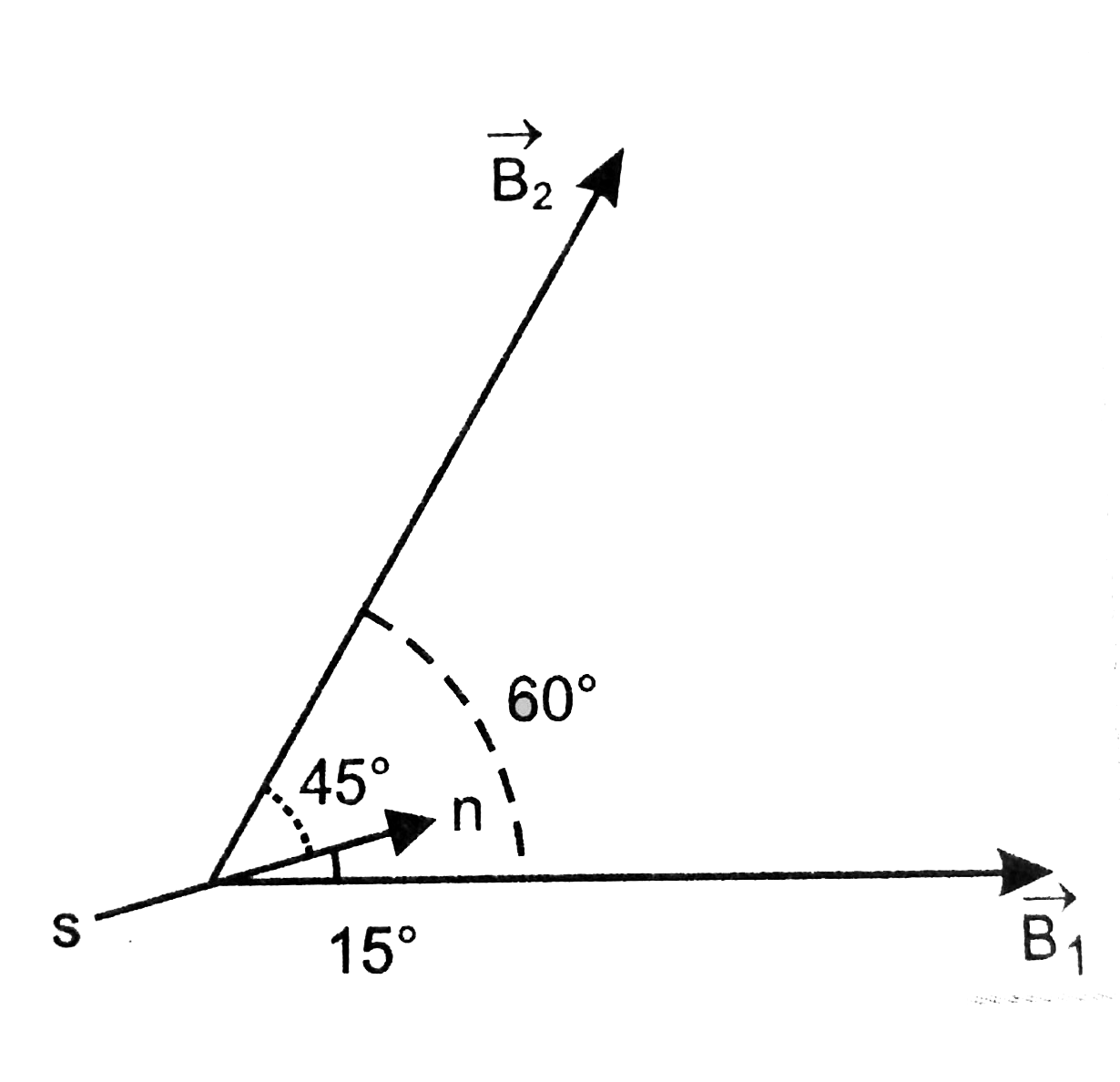
A magnetic dipole is under the influence of two magnetic fields. The angle between the field directions is 60∘ and one of the fields has a magnitude of 1⋅2×10−2 tesla. If the dipole comes to stable equilibrium at an angle of 15∘ with this field, figure, what is the magnitude of the other field?
 View Solution
View SolutionA magnetic dipole is acted upon by two magnetic fields with inclined to each other at an angle of 75∘. One of the fields has a magnitude of 15 mT. The dipole attains stable equilibrium at an angle of 30∘ with this field. The magnitude of the other field (in mT) is close to
View SolutionA magentic dipole in a constant magnetic field has
View SolutionA magentic dipole in a constant magnetic field has
View SolutionThe force exerted on a current-carrying wire placed in magnetic field is zero when the angle between the wire and the direction of magnetic field is :
View SolutionAn electric dipole is plced at an angle of 30∘ to a nonuniform electric field. The dipole will experience
View SolutionAn electric dipole is placed at an angle of 30∘ to a non-uniform electric field. The dipole will experience
View SolutionA magnetic dipole is placed in a uniform magnetic field of intensity B, oriented along the direction of the field. If the magnetic dipole moment is M, then the maximum work an external agent can perform in rotating the dipole will be
View SolutionAn electric dipole when placed in uniform electric field has zero potential energy. The angle between dipole moment and electric field is ....
View SolutionAn electric dipole when placed in uniform electric field has zero potential energy. The angle between dipole moment and electric field is ....
View Solution