When a resistance of 100Ω is connected in series with a galvanometer of resistance R, then its range is V. To double its range, a resistance of 1000Ω is connected in series. Find the value of R.
1100
1000
900
800
The correct Answer is:C
To solve the problem, we need to analyze the situation step by step.
Step 1: Understand the Initial Setup
When a resistance of 100 Ω is connected in series with a galvanometer of resistance R, the total resistance in the circuit is:
Rtotal1=100+R
Step 2: Write the Expression for Current
Using Ohm's Law, the current (I) flowing through the circuit when the voltage (V) is applied can be expressed as:
I=VRtotal1=V100+R
Let’s call this Equation (1).
Step 3: Analyze the Second Setup
To double the range, a resistance of 1000 Ω is connected in series with the galvanometer. The total resistance in this case becomes:
Rtotal2=1000+100+R=1100+R
Step 4: Write the Expression for Current in the Second Setup
In this case, the new voltage range is 2V, so the current can be expressed as:
I=2VRtotal2=2V1100+R
Let’s call this Equation (2).
Step 5: Set the Two Current Equations Equal
Since the current remains the same in both cases (because they are in series), we can set the two equations for current equal to each other:
V100+R=2V1100+R
Step 6: Cancel V from Both Sides
Assuming V is not zero, we can cancel it from both sides:
1100+R=21100+R
Step 7: Cross-Multiply to Solve for R
Cross-multiplying gives us:
1100+R=2(100+R)
Expanding the right side:
1100+R=200+2R
Step 8: Rearranging the Equation
Now, we rearrange the equation to isolate R:
1100−200=2R−R
This simplifies to:
900=R
Step 9: Conclusion
Thus, the value of R is:
R=900Ω
When a resistance of 100 Ω is connected in series with a galvanometer of resistance R, the total resistance in the circuit is:
Rtotal1=100+R
Using Ohm's Law, the current (I) flowing through the circuit when the voltage (V) is applied can be expressed as:
I=VRtotal1=V100+R
Let’s call this Equation (1).
To double the range, a resistance of 1000 Ω is connected in series with the galvanometer. The total resistance in this case becomes:
Rtotal2=1000+100+R=1100+R
In this case, the new voltage range is 2V, so the current can be expressed as:
I=2VRtotal2=2V1100+R
Let’s call this Equation (2).
Since the current remains the same in both cases (because they are in series), we can set the two equations for current equal to each other:
V100+R=2V1100+R
Assuming V is not zero, we can cancel it from both sides:
1100+R=21100+R
Cross-multiplying gives us:
1100+R=2(100+R)
Expanding the right side:
1100+R=200+2R
Now, we rearrange the equation to isolate R:
1100−200=2R−R
This simplifies to:
900=R
Thus, the value of R is:
R=900Ω
Topper's Solved these Questions
- PRACTICE PAPPERBook:NCERT FINGERTPS ENGLISHChapter:PRACTICE PAPPERExercise:Practice Papper 3
Explore 50 Videos
- PRACTICE PAPPERBook:NCERT FINGERTPS ENGLISHChapter:PRACTICE PAPPERExercise:Practice Paper 1
Explore 50 Videos
- PRACTICE PAPPERBook:NCERT FINGERTPS ENGLISHChapter:PRACTICE PAPPERExercise:Practice Paper 3
Explore 50 Videos
- NUCLEIBook:NCERT FINGERTPS ENGLISHChapter:NUCLEIExercise:Assertion And Reason
Explore 15 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBook:NCERT FINGERTPS ENGLISHChapter:RAY OPTICS AND OPTICAL INSTRUMENTSExercise:NCERT Exemplar
Explore 11 Videos
Similar Questions
A voltmeter has resistance 2000Ω and range 5 V. For increasing its range upto 20V, a resistance of
View SolutionA battery of emf 12 V and internal resistance 2Ω is connected in series with a tangent galvanometer of resistance 4Ω . The deflection is 60∘ when the plane of the coil is along the magnetic meridian . To get a deflection of 30∘, the resistance to be connected in series with the tangent galvanometer is
View Solution
Knowledge Check
A potentiometer wire of length 100cm has a resistance of 10Ω. It is connected in series with a resistance and a cell of emf 2V and of negligible interal resistance. A source of emf 10mV is balanced against a length of 40cm of the potentiometer wire. What is the value of external resistance?
A790ΩB890ΩC990ΩD1090Ω
The resistance 4R,16R,64R....∞ are connected in series. Find their equivalent resistance.
View SolutionA potentiometer wire of length 100cm having a resistance of 10Ω is connected in series with a resistance R and a cell of emf 2V of negligible internal resistance. A source of emf

of 10mV is balanced against a length of 40cm of the potentiometer wire. What is the value of resistance R ?
View SolutionA potentiometer wire of length 100cm having a resistance of 10Ω is connected in series with a resistance R and a cell of emf 2V of negligible internal resistance. A source of emf

of 10mV is balanced against a length of 40cm of the potentiometer wire. What is the value of resistance R ?
View SolutionVoltage range of galvanometer of resistance R is 0 to 1V. When its range increases upto 2V for this the additional resistance required in series will be
View Solution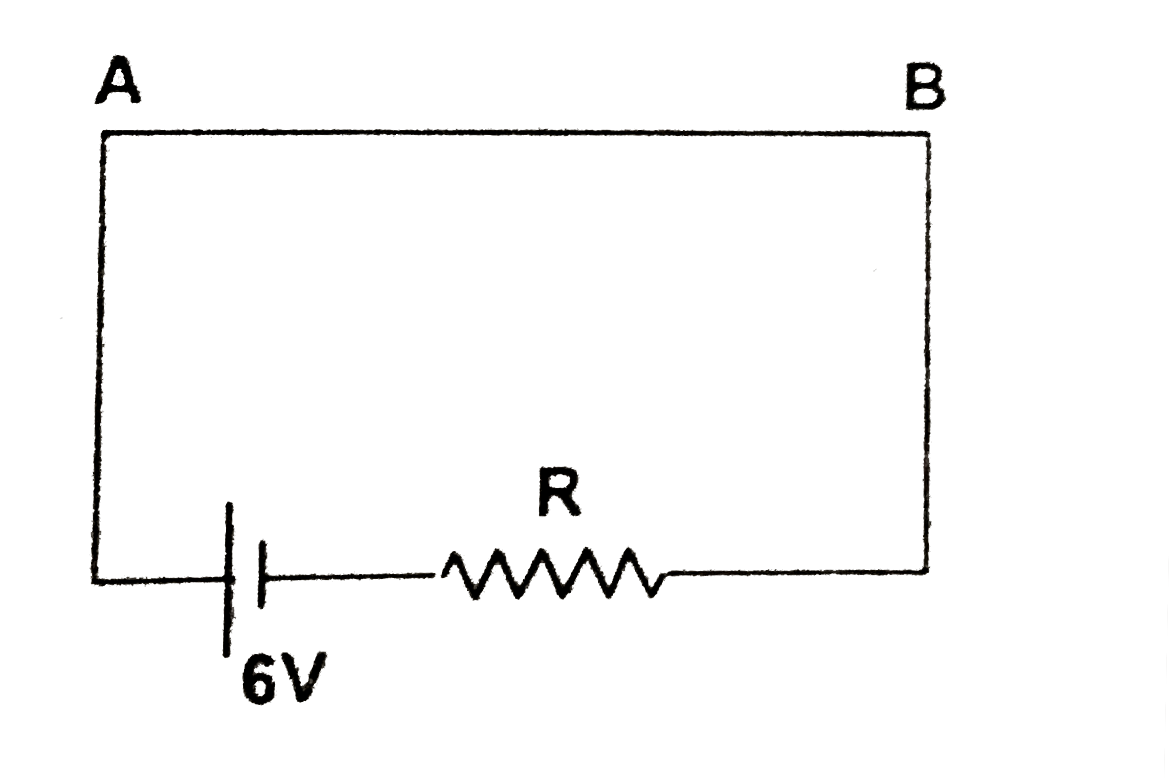
A uniform potentiometer wire AB of length 100 cm has a resistance of 5Ω and it is connected in series with an external resistance R and a cell of emf 6 V and negligible internal resistance. If a source of potential drop 2 V is balanced against a length of 60 cm of the potentiometer wire, the value of resistance R is
View SolutionThe temperature coefficient of resistance of tungsten is 4.5×10−3∘C−1 and that of germanium is −5×10−2∘C−1 . A tungsten wire of resistance 100Ω is connected in series with a germanium wire of resistance R. The value of R for which the resistance of combination does not change with temperature is .
View Solution(a) Briefly explain how a galvanometer is converted into a voltmeter.
(b) A voltmeter of a certain range is constructed by connecting a resistance of 980Ω in series with a galvanometer. When the resistance of 470Ω is connected in series, the range gets halved. Find the resistance of the galvanometer.
View Solution
