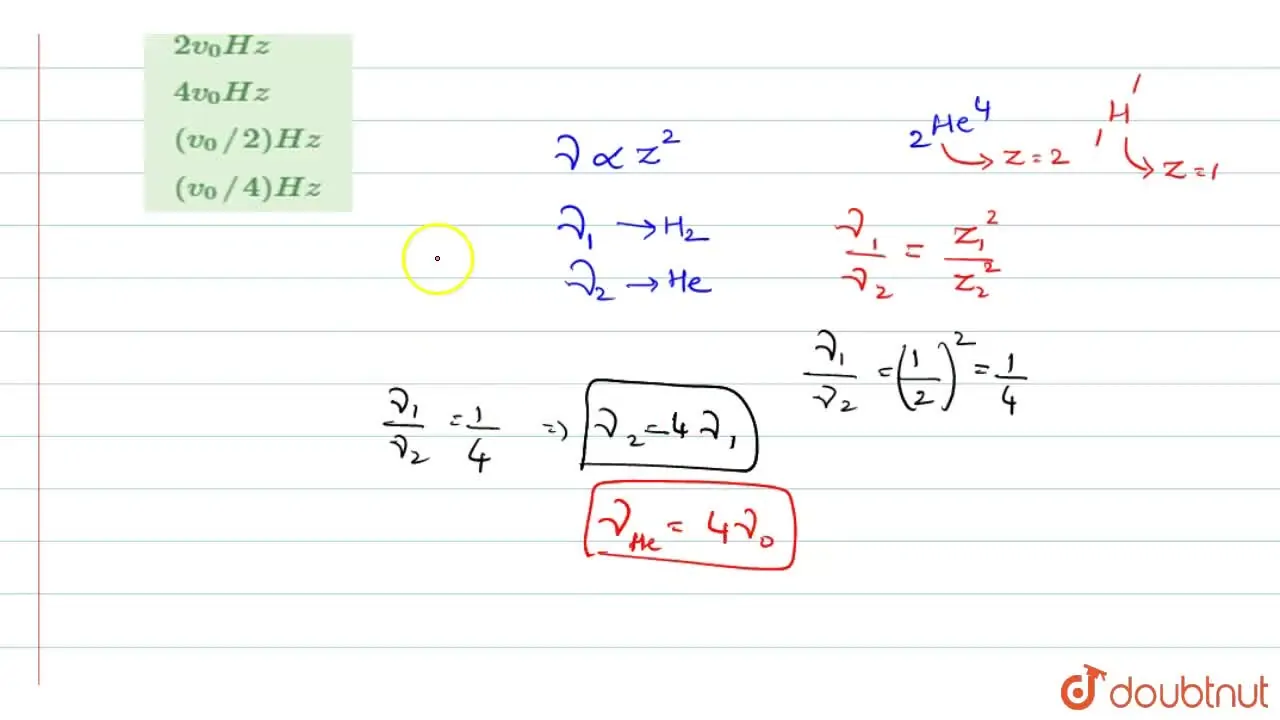
The frequency of 1st line Balmer series in $H_{2}$ atom is $v_{0}$ . The frequency of line emitted by single ionised He atom is

A

$2 v_{0} H z$

B

$4 v_{0} H z$

C

$(v_{0} / 2) H z$

D

$(v_{0} / 4) H z$

Video Solution

Text Solution

Generated By DoubtnutGPT

The correct Answer is:B

To solve the problem, we need to find the frequency of the line emitted by a singly ionized helium atom (He $^{+}$ ) in relation to the frequency of the first line of the Balmer series in a hydrogen atom (H). The frequency of the first line of the Balmer series in hydrogen is given as $v_{0}$ .
1. Understand the Balmer Series:
The Balmer series corresponds to the transitions of electrons in hydrogen from higher energy levels to the second energy level (n=2). The first line of this series is the transition from n=3 to n=2.
2. Identify the Atomic Numbers:
- For hydrogen (H), the atomic number $Z_{1} = 1$ .
- For singly ionized helium (He $^{+}$ ), the atomic number $Z_{2} = 2$ .
3. Frequency Relation:
The frequency of emitted light is proportional to the square of the atomic number. Therefore, we can express the relationship between the frequencies of hydrogen and helium as:
$\frac{v_{1}}{v_{2}} = \frac{Z_{1}^{2}}{Z_{2}^{2}}$
where $v_{1}$ is the frequency for hydrogen and $v_{2}$ is the frequency for helium.
4. Substituting Values:
Substitute the atomic numbers into the equation:
$\frac{v_{0}}{v_{2}} = \frac{1^{2}}{2^{2}} = \frac{1}{4}$
5. Rearranging the Equation:
Rearranging gives:
$v_{2} = 4 v_{0}$
6. Conclusion:
Thus, the frequency of the line emitted by the singly ionized helium atom is:
$v_{2} = 4 v_{0}$
Final Answer:
The frequency of the line emitted by the singly ionized helium atom is $4 v_{0}$ .
---

Updated on:18/9/2024

|

Class 12 PHYSICS QUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

Let $v_{1}$ be the frequency of series limit of Lyman series, $v_{2}$ the frequency of the first line of Lyman series and $v_{3}$ the frequency of series limit of Balmer series. Then which of the following is correct ?
A $v_{1} - v_{2} = v_{3}$
B $v_{2} - v_{1} = v_{3}$
C $v_{3} = \frac{1}{2} (v_{1} + v_{2})$
D $v_{1} + v_{2} = v_{3}$
If $v_{1}$ is the frequency of the series limit of lyman seies, $v_{2}$ is the freqency of the first line of lyman series and $v_{3}$ is the fequecny of the series limit of the balmer series, then
A $v_{1} - v_{2} = v_{3}$
B $v_{1} = v_{2} - v_{3}$
C $\frac{1}{v_{2}} = \frac{1}{v_{1}} + \frac{1}{v_{3}}$
D $\frac{1}{v_{1}} = \frac{1}{v_{2}} + \frac{1}{v_{3}}$

NCERT FINGERTPS ENGLISH-PRACTICE PAPPER-Practice Papper 2

Home
Ask Doubt
Profile