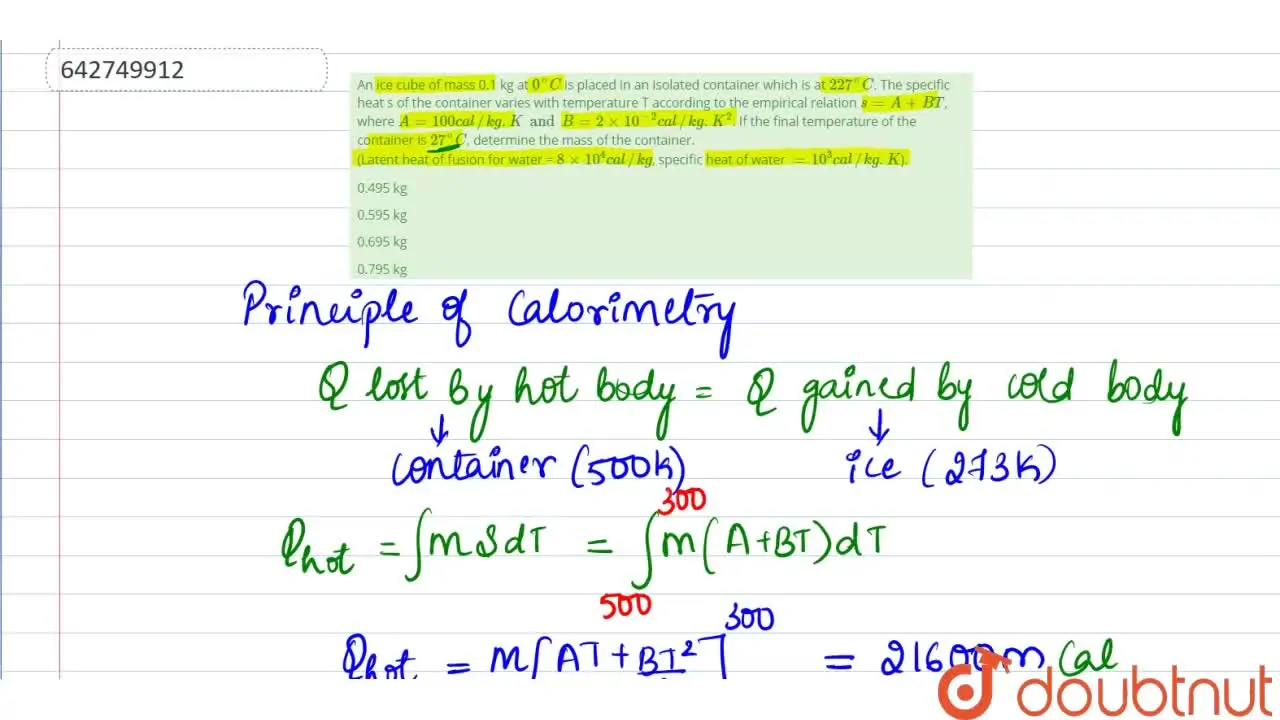
An ice cube of mass 0.1 kg at 0∘C is placed in an isolated container which is at 227∘C. The specific heat s of the container varies with temperature T according to the empirical relation s=A+BT, where A=100cal/kg.KandB=2×10−2cal/kg.K2. If the final temperature of the container is 27∘C, determine the mass of the container.
(Latent heat of fusion for water = 8×104cal/kg, specific heat of water =103cal/kg.K).
0.495 kg
0.595 kg
0.695 kg
0.795 kg
The correct Answer is:A
Step by step video, text & image solution for An ice cube of mass 0.1 kg at 0^@C is placed in an isolated container which is at 227^@C. The specific heat s of the container varies with temperature T according to the empirical relation s=A+BT, where A= 100 cal//kg.K and B = 2xx 10^-2 cal//kg.K^2. If the final temperature of the container is 27^@C, determine the mass of the container. (Latent heat of fusion for water = 8xx 10^4 cal//kg, specific heat of water =10^3 cal//kg.K). by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Related Playlists
Similar Questions
An ice cube of mass 0.1 kg at 0∘C is placed in an isolated container which is at 227∘C. The specific heat s of the container varies with temperature T according to the empirical relation s=A+BT, where A=100cal/kgKandB=2×(10−2)cal/kg. If the final temperature of the container is 27∘C, determine the mass of the container.
(Latent heat of fusion for water = 8×(104)cal/kg, specific heat of water =103cal/kg−K).View SolutionIf 10 g of ice at 0∘C is mixed with 10 g of water at 40∘C. The final mass of water in mixture is (Latent heat of fusion of ice = 80 cel/g, specific heat of water =1calg∘C)
View SolutionThe amount of heat required to raise the temperature of 75 kg of ice at 0oC to water at 10oC is (latent heat of fusion of ice is 80 cal/g, specific heat of water is 1 cal/goC)
View Solution1 g of steam at 100∘C and an equal mass of ice at 0∘C are mixed. The temperature of the mixture in steady state will be (latent heat of steam=540cal/g, latent heat of ice =80cal/g,specific heat of water =1cal/g∘C)
View Solution1 kg of ice at 0∘C is mixed with 1.5 kg of water at 45∘C [latent heat of fusion = 80 cal//gl. Then
View SolutionWhat will be the final temperature when 150 g of ice at 0∘C is mixed with 300 g of water at 50∘C. Specific heat of water =1cal/g/∘C. Latent heat of fusion of ice =80cal/g.
View SolutionA colorimeter contains 400g of water at a temperature of 5∘C. Then, 200g of water at a temperature of +10∘C and 400g of ice at a temperature of −60∘C are added. What is the final temperature of the contents of calorimeter?
Specific heat capacity of water −1000 cal/kg/K
Specific latent heat of fusion of ice =80×1000 cal/kg
Relative specific heat of ice =0.5View Solution50 g of ice at 0∘C is mixed with 50 g of water at 80∘C . The final temperature of the mixture is (latent heat of fusion of ice =80cal/g,sw=1cal/g∘C)
View SolutionFind the result of mixing 0.5 kg ice at 0∘C with 2 kg water at 30∘C. Given that latent heat of ice is L=3.36×105J/kg and specific heat of water is 4200J/kg/K.
View Solution120 g of ice at 0∘C is mixed with 100 g of water at 80∘C. Latent heat of fusion is 80 cal/g and specific heat of water is 1 cal/g−.∘C. The final temperature of the mixture is
View Solution