Two rods A and B of the same material and length have radii r1 and r2respective. When they are rigidly fixed at one end and twisted by the same torque applied at the other end, the ratio [the angle of twist at the end of Athe angle of twist at the end of B] equal to
r21r22
r31r32
r42r41
r41r42
The correct Answer is:C
To solve the problem, we need to find the ratio of the angles of twist for two rods A and B, which are subjected to the same torque. The angle of twist is given by the formula:
θ=2τLπr4η
where:
- θ is the angle of twist,
- τ is the applied torque,
- L is the length of the rod,
- r is the radius of the rod,
- η is the shear modulus of the material.
Step 1: Write the formula for the angle of twist for both rods
For rod A:
θA=2τLπr41η
For rod B:
θB=2τLπr42η
Step 2: Find the ratio of the angles of twist
We need to find the ratio θAθB:
θAθB=2τLπr41η2τLπr42η
Step 3: Simplify the ratio
The terms 2τL and πη cancel out:
θAθB=r42r41
Step 4: Write the final answer
Thus, the ratio of the angles of twist at the ends of rods A and B is:
θAθB=(r2r1)4
Final Result
The final result is:
θAθB=(r2r1)4
---
- θ is the angle of twist,
- τ is the applied torque,
- L is the length of the rod,
- r is the radius of the rod,
- η is the shear modulus of the material.
θA=2τLπr41η
θB=2τLπr42η
θAθB=(r2r1)4
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - HOTS
8 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - NCERT
8 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - ELASTIC MODULI
69 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - NCERT Exemplar
5 Videos
- MOTION IN A PLANE BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MOTION IN A PLANE EXERCISE - Assertion And Reason
15 Videos
Similar Questions
Knowledge Check
Two particles of equal masses are revolving in circular paths of radii, r1andr2 respectively with the same period . The ratio of their centripetal force is -
Ar1/r2B√r2/r1C(r1/r2)2D(r2/r1)2
The wires A and B shown in Fig. are made of the same material and have radii rA and rB, respectively. The block between them has a mass m. When the force F is mg/3, one of the wires breaks. Then
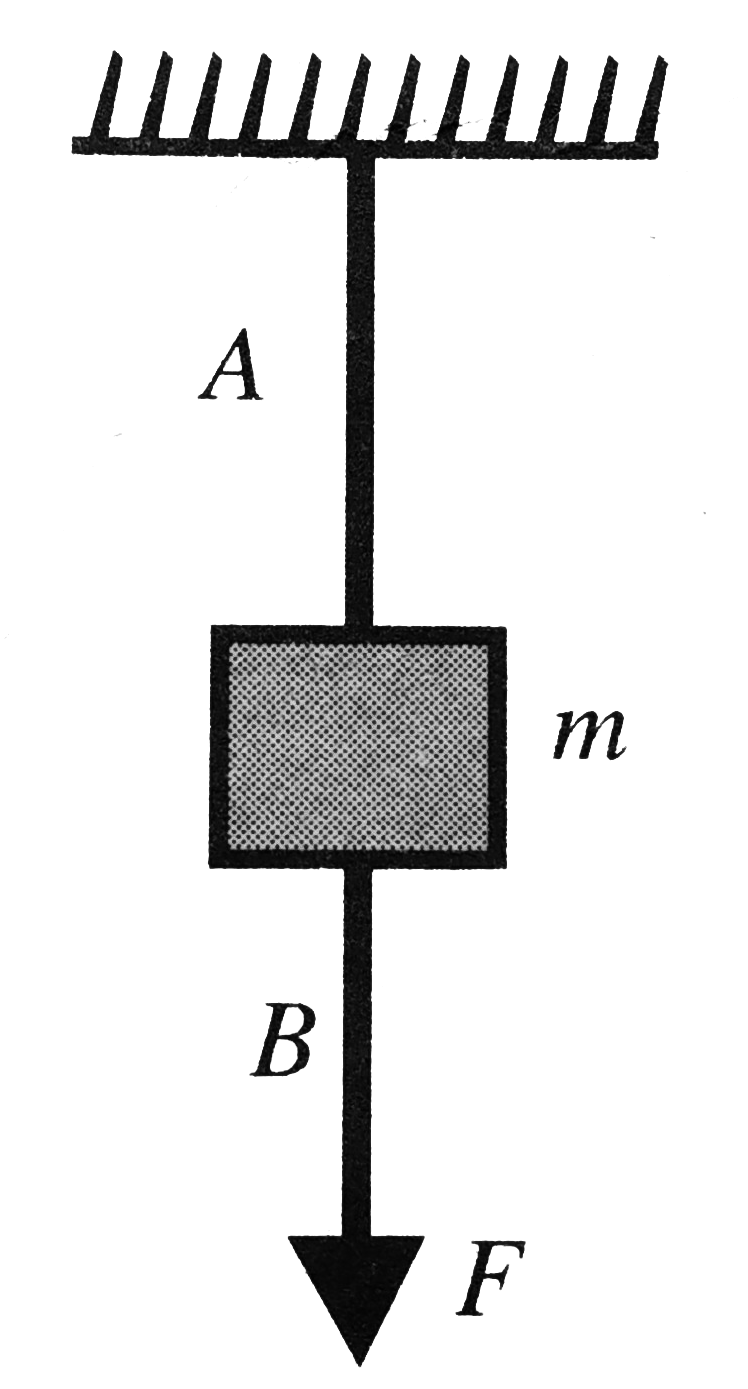
View SolutionThe wires A and B shown in Fig. are made of the same material and have radii rA and rB, respectively. The block between them has a mass m. When the force F is mg/3, one of the wires breaks. Then

View SolutionTwo wires of the same material (Young's modulus=Y) and same length L but radii R and 2R respectively are joined end to end and a weight w is suspended from the combination as shown in the figure. The elastic potential energy in the system is
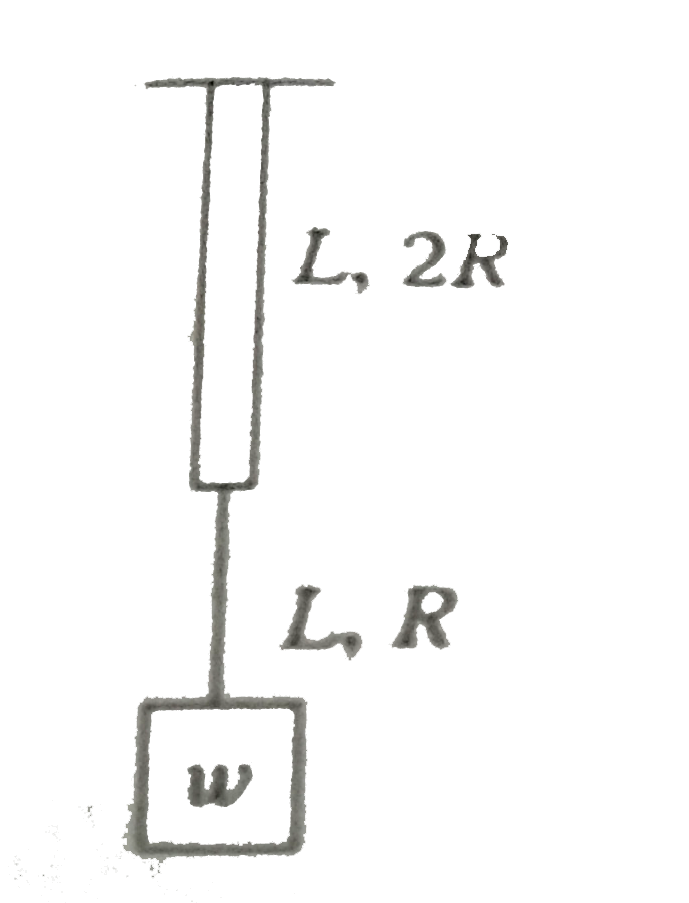
View SolutionTwo wires of the same material (Young's modulus=Y) and same length L but radii R and 2R respectively are joined end to end and a weight w is suspended from the combination as shown in the figure. The elastic potential energy in the system is

View SolutionTwo vibrating strings of the same material but lengths L and 2L have radii 2r and r respectively. They are stretched under the same tension. Both the string vibrate in their fundamental nodes, the one of length L with freuqency v1 and the other with frequency v2. the ratio v1/v2 is given by
View SolutionTwo wires of the same material have lengths in the ratio 1:2 and their radii are in the ratio 1:√2 If they are stretched by applying equal forces, the increase in their lengths will be in the ratio
View SolutionTwo wires of the same material have lengths in the ratio 1:2 and their radii are in the ratio 1:√2 If they are stretched by applying equal forces, the increase in their lengths will be in the ratio
View Solution
