A cork of mass 10 g is floating on water. The net force acting on the cork is
10N
10−3N
10−2N
zero
The correct Answer is:D
To solve the problem of finding the net force acting on a cork of mass 10 g floating on water, we can follow these steps:
1. Identify the Forces Acting on the Cork:
- The cork experiences two main forces:
- The gravitational force (weight) acting downwards, which is given by mg.
- The buoyant force (upthrust) acting upwards, which is equal to the weight of the water displaced by the cork.
2. Calculate the Weight of the Cork:
- The mass of the cork is given as 10 g. We need to convert this into kilograms for standard SI units:
m=10g=0.01kg
- The gravitational force (weight) can be calculated using the formula:
Fg=mg
where g (acceleration due to gravity) is approximately 9.81m/s2.
- Therefore,
Fg=0.01kg×9.81m/s2=0.0981N
3. Understand the Buoyant Force:
- According to Archimedes' principle, the buoyant force (upthrust) acting on the cork is equal to the weight of the water displaced by the cork. Since the cork is floating, this buoyant force equals the weight of the cork.
- Thus, the buoyant force Fb is also:
Fb=0.0981N
4. Determine the Net Force:
- The net force acting on the cork can be calculated by subtracting the upward buoyant force from the downward gravitational force:
Fnet=Fg−Fb
- Since Fg=Fb when the cork is floating:
Fnet=0.0981N−0.0981N=0N
5. Conclusion:
- The net force acting on the cork is 0N. This indicates that the cork is in equilibrium, with the forces balanced.
Final Answer:
The net force acting on the cork is 0 N.
- The cork experiences two main forces:
- The gravitational force (weight) acting downwards, which is given by mg.
- The buoyant force (upthrust) acting upwards, which is equal to the weight of the water displaced by the cork.
- The mass of the cork is given as 10 g. We need to convert this into kilograms for standard SI units:
m=10g=0.01kg
- The gravitational force (weight) can be calculated using the formula:
Fg=mg
where g (acceleration due to gravity) is approximately 9.81m/s2.
- Therefore,
Fg=0.01kg×9.81m/s2=0.0981N
- According to Archimedes' principle, the buoyant force (upthrust) acting on the cork is equal to the weight of the water displaced by the cork. Since the cork is floating, this buoyant force equals the weight of the cork.
- Thus, the buoyant force Fb is also:
Fb=0.0981N
- The net force acting on the cork can be calculated by subtracting the upward buoyant force from the downward gravitational force:
Fnet=Fg−Fb
- Since Fg=Fb when the cork is floating:
Fnet=0.0981N−0.0981N=0N
- The net force acting on the cork is 0N. This indicates that the cork is in equilibrium, with the forces balanced.
The net force acting on the cork is 0 N.
Topper's Solved these Questions
- LAWS OF MOTIONBook:NCERT FINGERTPS ENGLISHChapter:LAWS OF MOTIONExercise:HOTS
Explore 8 Videos
- LAWS OF MOTIONBook:NCERT FINGERTPS ENGLISHChapter:LAWS OF MOTIONExercise:Exemplar Problems
Explore 9 Videos
- KINETIC THEORYBook:NCERT FINGERTPS ENGLISHChapter:KINETIC THEORYExercise:Assertion And Reason
Explore 10 Videos
- MECHANICAL PROPERTIES OF FLUIDSBook:NCERT FINGERTPS ENGLISHChapter:MECHANICAL PROPERTIES OF FLUIDSExercise:NCERT Exemplar
Explore 5 Videos
Similar Questions
The net force acting is not zero on
View SolutionGive the magnitude and direction of the net force acting on
(a) a drop of rain falling down with a constant speed
(b) a cork of mass 10 g floating on water
(c) a kite skillfully held stationary in the sky
(d) a car moving with a constant velocity of 30kh/h on a rough road
(e) a high speed electron in space free from all gravitational objects and free of electric and magnetic fields.
View Solution
Knowledge Check
A stone of mass 1 kg is lying on the floor of a train which is accelerating with 1ms−2. The net force acting on the stone is
AzeroB1NC5ND10N
In Which of the following cases the net force acting on the body is not zero ?
View SolutionA mass M is suspended as shown in fig. The system is in equilibrium. Assume pulleys to be massless. K is the force constant of the spring.
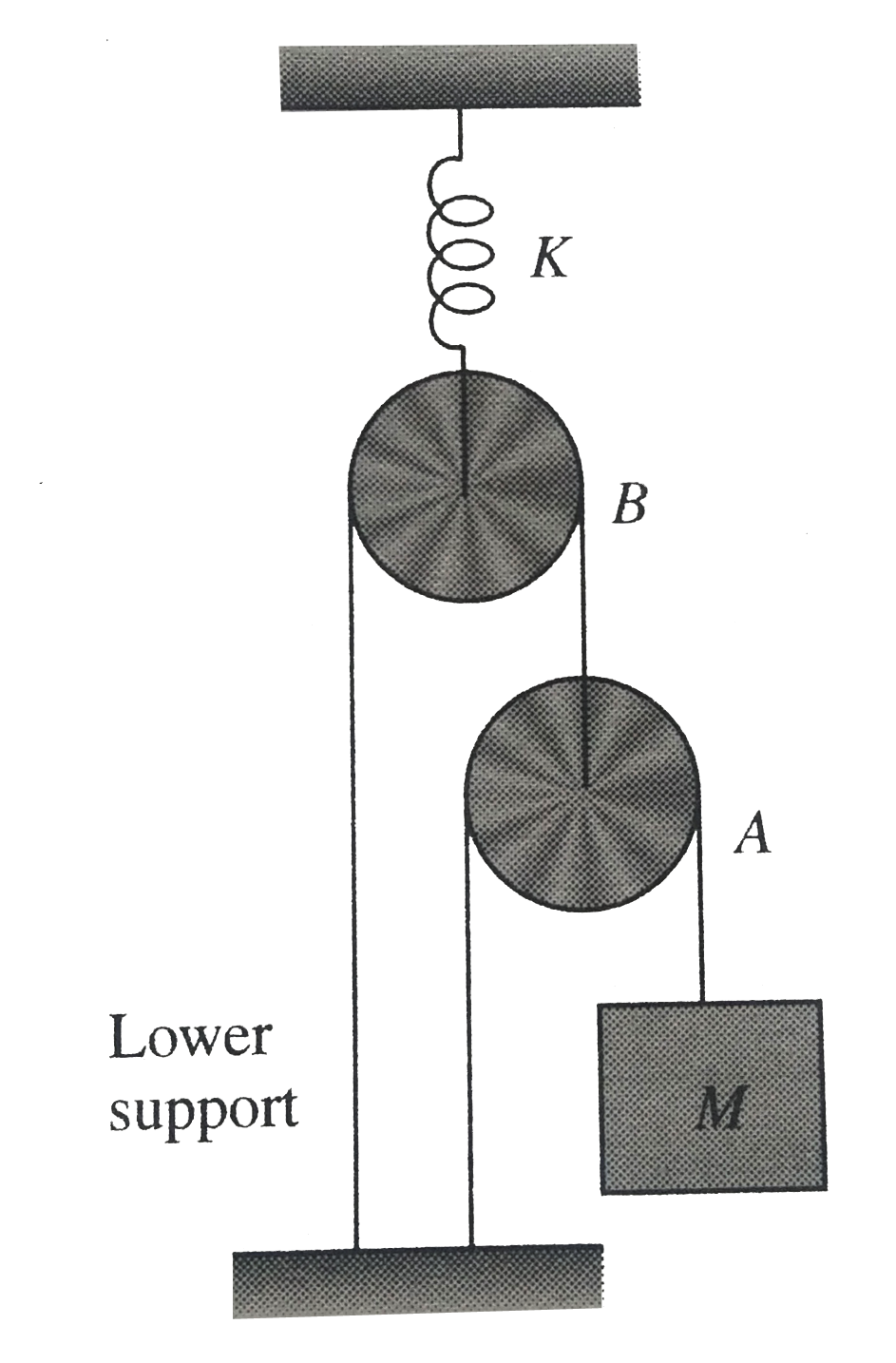
Find the net tension force acting on the lower support.
View SolutionA block of wood is floating on water with its dimensions 50cm×50cm×50cm inside water. Calculate the buoyant force acting on the block. Take g=9.8Nkg−1
View SolutionThe vessel shown in the figure contains water, take ρwater=1000kg/m2,g=m/s2). For this arrangement, answer the following questions:
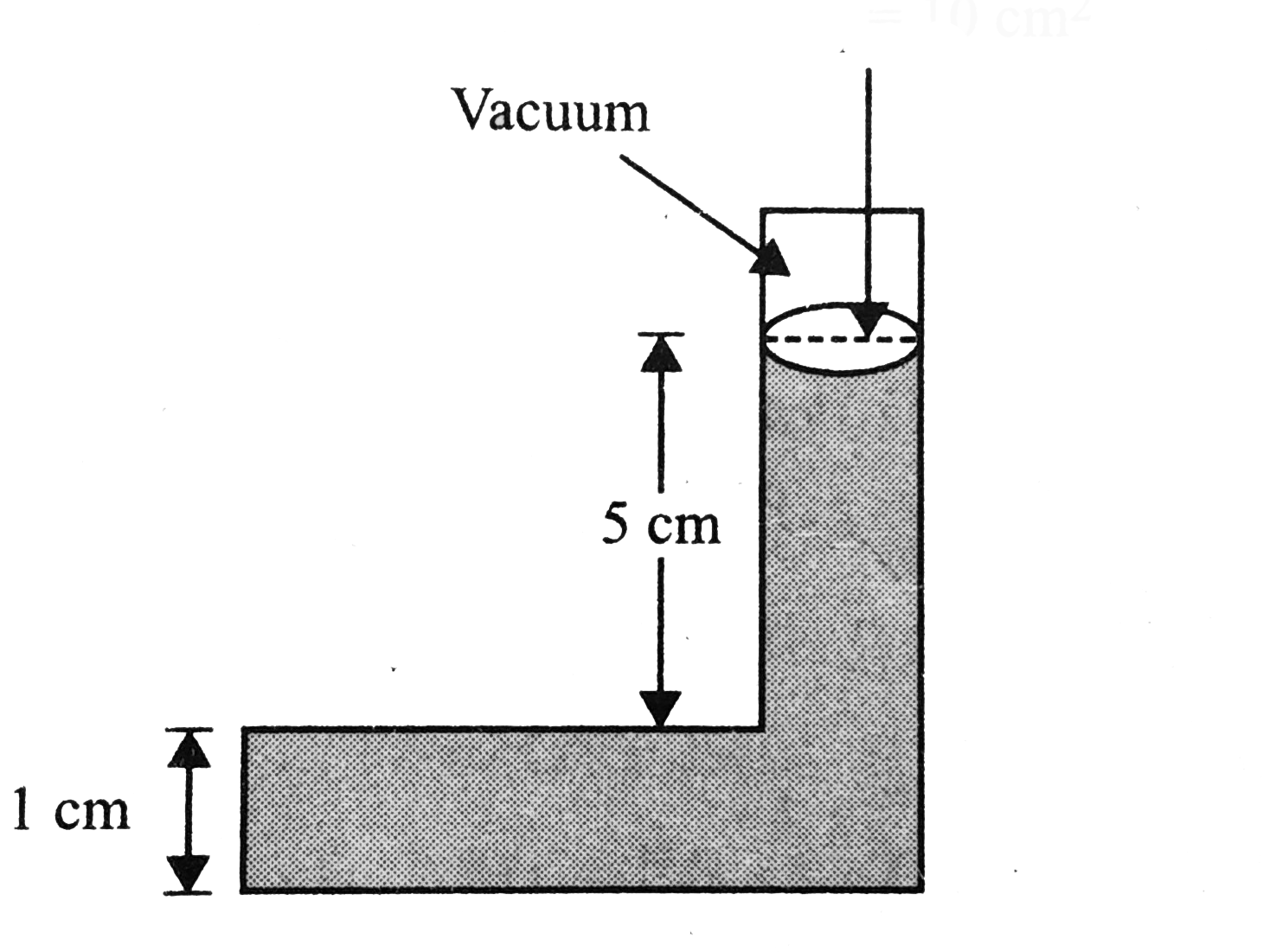
The net force acting on the base of the vessel is
View SolutionIf an object is moving with a constant acceleration, the net force acting on that body is
View SolutionA : For a body to be in rotational equilibrium the net torque acting on the body about any point is zero.
R : For net torque to be zero, net force should also be zero.
View SolutionStatement I: In fig., ground is smooth and masses of both the block are differents. The net force acting on each of the block is not same.
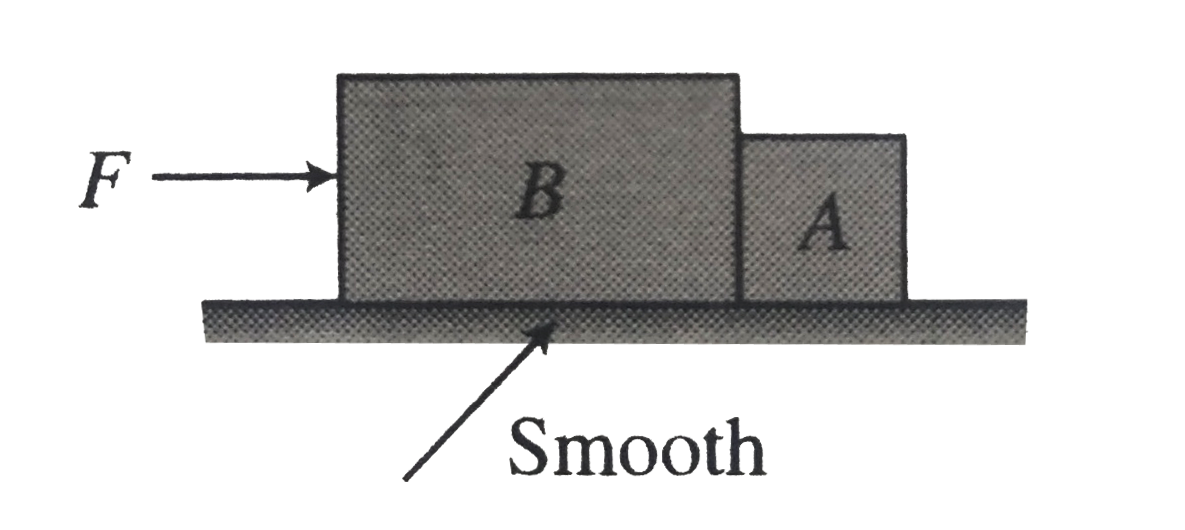
Statement II: Acceleration of both will be different.
View Solution