समीकरणों 2sin2x+sin22x=2 व sin2x+cos2x=tanx के उभ्यनिष्ठ मुल है।
x=(2n−1)π2
x=(2n+1)π4
x=(2n+1).π3
इनमे से कोई नही
The correct Answer is:B
Step by step video, text & image solution for समीकरणों 2 sin^(2) x + sin^(2) 2x =2 व sin 2 x + cos 2 x = tan x के उभ्यनिष्ठ मुल है। by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- त्रिकोणमितीय समीकरण एवं असमिकाएँ त्रिभुजों के गुण उँचाई एवं दुरी BOOK - ERRORLESS HINDICHAPTER - त्रिकोणमितीय समीकरण एवं असमिकाएँ त्रिभुजों के गुण उँचाई एवं दुरी EXERCISE - JEE Advanced
47 Videos
- त्रिकोणमितीय समीकरण एवं असमिकाएँ त्रिभुजों के गुण उँचाई एवं दुरी BOOK - ERRORLESS HINDICHAPTER - त्रिकोणमितीय समीकरण एवं असमिकाएँ त्रिभुजों के गुण उँचाई एवं दुरी EXERCISE - Solved Multiple Choice Question (ऊँचाई एवं दूरी)
35 Videos
- गणितीय तर्कशास्त्रBOOK - ERRORLESS HINDICHAPTER - गणितीय तर्कशास्त्रEXERCISE - Jee Advanced (Reasoning )
3 Videos
- त्रिकोणमितीय अनुपात, फलन एवं सर्वसमिकायेBOOK - ERRORLESS HINDICHAPTER - त्रिकोणमितीय अनुपात, फलन एवं सर्वसमिकायेEXERCISE - JEE Advanced (Matrix Match type questions)
5 Videos
Similar Questions
0<θ<π2 के लिए 6Σm=1cosec(θ+(m−1)π4)cosec(θ+mπ4)=4√2 का के हल निम्न है।
View Solutionभूमि के एक बिन्दु पर एक मीनार के शिखर का उन्त्रयन कोण 30∘ है यदि मीनार की और 20 मीटर चलने पर उन्यन कोण 60∘ हो जाता है तो मीनार की ऊँचाई हैं
View Solutionकथन - 1 : ~ (p ↔ ~ q) और p ↔ q तुल्यमान (equivalent) हैं।
कथन -2: ~ (p↔ ~q) एक पुनरुक्ति (tautology) है ।
View Solutionनिम्नलिखित कॉलम I को कॉलम II से मिलाये
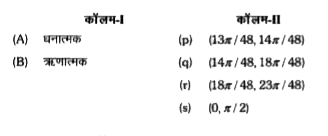
View Solution∫2sinx(3+sin2x)dxisequa<o 12ln∣∣∣2+sinx−cosx2−sinx+cosx∣∣∣−1√2tan−1(sinx+cosx√2)+c 12ln∣∣∣2+sinx−cosx2−sinx+cosx∣∣∣−12√2tan−1(sinx+c0sx√2)+c 14ln∣∣∣2+sinx−cosx2−sinx+cosx∣∣∣−1√2tan−1(sinx+c0sx√2)+c noneofthese
View SolutionLet f(x)=∣∣cos(x+x2)sin(x+x2)−cos(x+x2)sin(x−x2)cos(x−x2)sin(x−x2)sin2x0sin(2x2)∣∣ . Find the value of f′(0).
View SolutionCompute the following: [cos2xsin2xsin2xcos2x]+[sin2xcos2xcos2xsin2x]
View SolutionLet f(x)=∣∣ ∣ ∣∣cos(x+x2)sin(x+x2)−cos(x+x2)sin(x−x2)cos(x−x2)sin(x−x2)sin2x0sin(2x2)∣∣ ∣ ∣∣.
Find the value of f'(0).
View Solution(cos^(3) x- sin^(2) x)/(cos x - sin x)=(1)/(2) (2 + sin 2x)
View SolutionIf 2cosx≠2sin,x then the general solution of sin2x−cos2x=2sin2x is
View SolutionSimplify tan−1⎛⎜ ⎜⎝cos(x2)+sin(x2)cos(x2)−sin(x2)⎞⎟ ⎟⎠
View SolutionLet f(x)=∣∣ ∣ ∣∣cos(x+x2)sin(x+x2)−cos(x+x2)sin(x−x2)cos(x−x2)sin(x−x2)sin2x0sin(2x2)∣∣ ∣ ∣∣.
Find the value of f'(0).
View SolutionLet f(x)=∣∣ ∣ ∣∣cos(x+x2)sin(x+x2)−cos(x+x2)sin(x−x2)cos(x−x2)sin(x−x2)sin2x0sin(2x2)∣∣ ∣ ∣∣.
Find the value of f'(0).
View SolutionSolve
sin3x+sinx+2cosx=sin2x+2cos2x
View SolutionThe common roots of the equations 2sin2x+sin22x=2andsin2x+cos2x=tanx is
View Solution
