समघात समीकरणों के निकाय tx+(t+1)y+(t−1)z=0,(t+1)x+ty+(t+2)z=0
(t−1)x+(t+2)y+tz=0 का एक अशून्य हल है
t के सिर्फ तीन वास्तविक मानों के लिए
t के सिर्फ दो वास्तविक मानों के लिए
t के सिर्फ एक वास्तविक मान के लिए
t के अनन्त मानों के लिए,
The correct Answer is:C
Step by step video, text & image solution for समघात समीकरणों के निकाय tx+(t+1)y+(t-1)z=0,(t+1)x+ty+(t+2)z=0 (t-1)x+(t+2)y+tz=0 का एक अशून्य हल है by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- सारणिक तथा आव्यूह BOOK - ERRORLESS HINDICHAPTER - सारणिक तथा आव्यूह EXERCISE - Solved Multiple Choice Questions ( आव्यूह के प्रकार, आव्यूह का बीजगणित)
130 Videos
- सारणिक तथा आव्यूह BOOK - ERRORLESS HINDICHAPTER - सारणिक तथा आव्यूह EXERCISE - EXERCISE
262 Videos
- सारणिक तथा आव्यूह BOOK - ERRORLESS HINDICHAPTER - सारणिक तथा आव्यूह EXERCISE - Solved Multiple Choice Questions (सारणिक का प्रसार , सारणिक के रूप में समीकरण का हल एवं सारणिक के गुणधर्म)
150 Videos
- सरल रेखा BOOK - ERRORLESS HINDICHAPTER - सरल रेखा EXERCISE - JEE ADVANCE (Matrix Match type questions)
4 Videos
Similar Questions
यदि M=[1223] और M2−λM−I2=0 तब λ=
View Solution∣∣ ∣ ∣∣111cos(nx)cos(n+1)xcos(n+2)xsin(nx)sin(n+1)xsin(n+2)x∣∣ ∣ ∣∣ निर्भर नहीं करता है
View Solution∣∣ ∣ ∣∣1aa2−bc1bb2−ac1cc2−ab∣∣ ∣ ∣∣=
View Solution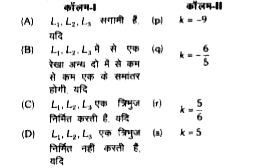
View SolutionIf y(t) is a solution of (1+t)dydt−ty=1andy(0)=−1 then show that y(1)=−12.
View SolutionA function y=f(x) is given by x=(1)/(1+t^(2)) and y=(1)/(t(1+t^(2))) for all t>0 then f is
View SolutionFind x, y, z, t if 2[xzyt]+3[1−102]=3[3546] .
View SolutionThe system of homogenous equations
tx+(t+1)y+(t−1)z=0, (t+1)x+ty+(t+2)z=0, (t−1)x+(t+2)y+tz=0 has a non trivial solution for
View Solutionयदि y(t) अवकल समीकरण (t+1)dydt−ty=1 का एक हल है तब y(0)=-1 व t=1 पर हल होगा-
View SolutionIf y(t) is a solution of (1+t)dydt−ty+1=0andy(0)=1, then : y(1)=…
View Solutiont के किस मान के लिए निम्नलिखित युग्मो का कोई हल नहीं होगा ?
(a) tx+y=1(t−1)x+2y=3
(b)x+ty+t=0x+(2−t)y=2
(c)3x+y=1(2t−1)x+(y−1)y=2t+1
View SolutionThe number of real values of t such that the system of homogeneous equations tx+(t+1)y+(t−1)z=0,(t+1)x+ty+(t+2)z=0,(t−1)x+(t−2)y+tz=0 has non trivial solutions is
View SolutionIf the system of homogeneous equations
tx+(t+1)y+(t−1)z=0
(t+1)x+ty+(t+2)z=0
(t−1)x+(t+2)y+tz=0 in x,y,z has a non-trivial solution, then t is a root of the equation
View Solutionयदि निकाय के समीकरणों x−ky−z=0,kx−y−z=0 तथा x+y−z=0 का एक अशून्य हल है, तो k का संभावित मान होगा
View SolutionIf y(t) is solution is (t+1)dydx−ty=1,y(0)=−1 At t=1, the soulution is :
View Solution
