The angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower, is 30∘. Find the height of the tower.
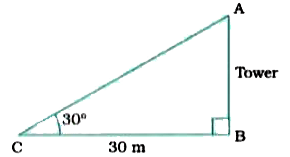 Solution in Gujrati
Solution in Gujrati
The correct Answer is:10√3m
Step by step video, text & image solution for The angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower, is 30^(@). Find the height of the tower. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SOME AAPLICATIONS OF TRIGONOMETRYBOOK - KUMAR PRAKASHANCHAPTER - SOME AAPLICATIONS OF TRIGONOMETRYEXERCISE - Test Your Skills
5 Videos
- SOME AAPLICATIONS OF TRIGONOMETRYBOOK - KUMAR PRAKASHANCHAPTER - SOME AAPLICATIONS OF TRIGONOMETRYEXERCISE - Practice Thoroughly
7 Videos
- SOME AAPLICATIONS OF TRIGONOMETRYBOOK - KUMAR PRAKASHANCHAPTER - SOME AAPLICATIONS OF TRIGONOMETRYEXERCISE - Other Important Examples
3 Videos
- REAL NUMBERS BOOK - KUMAR PRAKASHANCHAPTER - REAL NUMBERS EXERCISE - TEST YOUR SKILLS
26 Videos
- STATISTICSBOOK - KUMAR PRAKASHANCHAPTER - STATISTICSEXERCISE - Objective Questions (True or false)
5 Videos
Similar Questions
The angle of elevation of the top of a tower 30m high from the foot of another tower in the same plane is 60∘ and the angle of elevation of the top the second tower from the foot of the first tower is 30∘. Find the distance between the two towers and also find the height of the other tower.
View SolutionA jet plane is at a vertical height of h. The angles of depression of two tanks on the ground in the same line with the plane are αandβ(α>β). Prove that the distance between the tanks is h(tanα−tanβ)tanαtanβ
View SolutionA building stands on a horizontal plane and is surmounted by a vertical flag-stagg of height h. At a point on the plane, the angles of elevation of the bottom and the top of the flag-staff are αandβ respectively. Prove that the height of the building is htanαtanβ−tanα
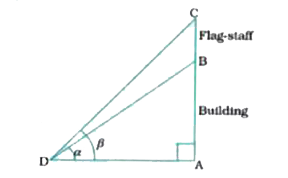
View SolutionShow that the square of any positive integer is of the form 4m or 4m + 1 for some integer m.
View SolutionFor any frequency distribution, mean is always greater than mode.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower, is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30 m away from the foot of the tower is 30∘. Find the height of the tower.
View SolutionThe angle elevation of the top of a tower from a point C on the ground. Which is 30 m away from the foot of the tower is 30∘ . Find the height of the tower.

View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower, is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point the ground, which is 30 m away from the foot of the tower, is 30∘. Find the height of the tower.
View SolutionThe angle elevation of the top of a tower from a point C on the ground. Which is 30 m away from the foot of the tower is 30∘ . Find the height of the tower.

View SolutionThe angle elevation of the top of a tower from a point C on the ground. Which is 30 m away from the foot of the tower is 30∘ . Find the height of the tower.

View SolutionFind the height of the tower when the top angle of the tower is 30 ° from a point on the ground 30m away from the base of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground which is 30 m away from the foot of the tower is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30 m away from the foot of the tower, is 30∘. Find the height of the tower.
View Solution
