In cyclic quadrilateral ABCD, ∠A=70∘and∠B+∠C=160∘. Then,∠B= ………
35∘
25∘
50∘
130∘
The correct Answer is:C
To solve the problem, we need to find the value of angle B in the cyclic quadrilateral ABCD, given that angle A = 70° and angle B + angle C = 160°.
1. Understanding Cyclic Quadrilaterals:
In a cyclic quadrilateral, the sum of the opposite angles is always 180°. Therefore, we can write the following equation:
Angle A+Angle C=180°
2. Substituting the Given Value:
We know that angle A = 70°. Substituting this into the equation gives:
70°+Angle C=180°
3. Solving for Angle C:
To find angle C, we can rearrange the equation:
Angle C=180°−70°=110°
4. Using the Given Information:
We are also given that angle B + angle C = 160°. We can substitute the value of angle C we just found:
Angle B+110°=160°
5. Solving for Angle B:
Rearranging this equation gives:
Angle B=160°−110°=50°
Final Answer:
Thus, the value of angle B is:
Angle B=50°
In a cyclic quadrilateral, the sum of the opposite angles is always 180°. Therefore, we can write the following equation:
Angle A+Angle C=180°
We know that angle A = 70°. Substituting this into the equation gives:
70°+Angle C=180°
To find angle C, we can rearrange the equation:
Angle C=180°−70°=110°
We are also given that angle B + angle C = 160°. We can substitute the value of angle C we just found:
Angle B+110°=160°
Rearranging this equation gives:
Angle B=160°−110°=50°
Thus, the value of angle B is:
Angle B=50°
Topper's Solved these Questions
- CIRCLES BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CIRCLES EXERCISE - SUM TO ENRICH REMAMBER
6 Videos
- CIRCLES BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CIRCLES EXERCISE - SKILL TESTING EXERCISE
11 Videos
- INTRODUCTION TO EUCLID.S GEOMETRYBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - INTRODUCTION TO EUCLID.S GEOMETRYEXERCISE - MULTIPLE CHOICE QUESTIONS
3 Videos
Similar Questions
Knowledge Check
In a quadrilateral ABCD, ∠D=60∘and∠C=100∘ . The bisectors of ∠Aand∠B meet at the point P. The measure of ∠APB is
A80∘B100∘C60∘D70∘PQRS is a quadrilateral which is formed by joining the mid-points of a quadrilateral ABCD, such that ∠A=75∘,∠B=95∘,∠C=110∘.If ∠PQR=90∘, what is the value of ∠PSR ?
A90∘B110∘C60∘D75∘In cyclic quadrilateral ABCD,∠A−∠C=20∘. Then,∠A= ..........
A20∘B80∘C100∘D50∘
If ABCD be a cyclic quadrilateral in which ∠A=4x∘,∠B=7x∘,∠C=5y∘,∠D=y∘, then x: y is
यदि ABCD एक चक्रिय चतुर्भुज हो जिसमें ∠A=4x∘,∠B=7x∘,∠C=5y∘,∠D=y∘, तो x: y कितना होगा ।
View Solution(B) Solve the following subquestions :
In cyclic quadrilateral ABCD, ∠A=65∘ then what is the mesure of ∠C ?
View SolutionIn a quadrilateral ABCD,∠D=60∘and∠C=100∘. The bisectors of ∠Aand∠B meet at the point P. The measure of ∠APB is
View SolutionPQRS is a quadrilateral which is formed by joining the mid-points of a quadrilateral ABCD, such that ∠A=75∘,∠B=95∘,∠C=110∘.If ∠PQR=90∘, what is the value of ∠PSR ?
View Solution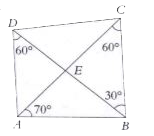
In the given figure ABCD is a quadrilateral ∠ADB=60∘,∠BAC=70∘,∠DBC=30∘,and∠ACB=60∘. Find ∠DAC
View Solution