Nucleus A decays to B with decay constant λ1 and B decuys to C with decay constants λ2. Initially at t=0, number of nuclei of A and B are 2N0 and N0 respectively. At some instant t=t0, number of nuclei of B stop changing. Find t0 if at this intant number of nuclei of B is 3N02Solution in Telugu
t0=1λ1ln4λ13λ2
t0=1λ2ln4λ13λ2
NA=3N02λ2λ1 to t=t0
NA=2N03λ2λ1 at t=t0
The correct Answer is:option 4
Step by step video, text & image solution for Nucleus A decays to B with decay constant lamda_(1) and B decuys to C with decay constants lamda_(2). Initially at t=0, number of nuclei of A and B are 2N_(0) and N_(0) respectively. At some instant t=t_(0), number of nuclei of B stop changing. Find t_(0) if at this intant number of nuclei of B is (3N_(0))/(2) by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- NUCLEAR PHYSICSBOOK - AAKASH SERIESCHAPTER - NUCLEAR PHYSICSEXERCISE - ADDITIONAL PRACTICE LEVEL-II (LECTURE SHEET (ADVANCED)) Linked Comprehension Type Questions Passage-I :)
2 Videos
- NUCLEAR PHYSICSBOOK - AAKASH SERIESCHAPTER - NUCLEAR PHYSICSEXERCISE - ADDITIONAL PRACTICE LEVEL-II (LECTURE SHEET (ADVANCED)) Linked Comprehension Type Questions Passage-II :)
2 Videos
- NUCLEAR PHYSICSBOOK - AAKASH SERIESCHAPTER - NUCLEAR PHYSICSEXERCISE - ADDITIONAL PRACTICE LEVEL-II (LECTURE SHEET (ADVANCED)) Straight Objective Type Questions )
10 Videos
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH SERIESCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - EXERCISE-III
45 Videos
- NUCLEIBOOK - AAKASH SERIESCHAPTER - NUCLEIEXERCISE - Practice Exercise
40 Videos
Similar Questions
If we deuterium nuclei get close enough to each other, the attraction of the strong nuclear force will fuse them to make an isotope of helium. This process releases a huge amount of energy. The range of nuclear force is 10−15 m. This is the principle behind the nuclear fusion reactor. The deuterium nuclei moves to fast that, it is not possible to contain them by physical walls. Therefore they are confined magnetically (Assume coulomb law to hold even at 10−18 m)
Two deuterium nuclei having same speed undergo a head on collision. Which of the following is closest to the minimum value of v (in km/sec) for which fusion occurs
View SolutionNuclei of radioactive element X. are being produced at a constant rute .K. and this element decays to a stable nucleus .Y. with decay constant .λ. with half lite ..t0... At time t=0, there are ..N..0.. number of nucle of the element .X. then
The number Nx of nuclei of .X. at time t=t0 is
View SolutionNucleus A decays to B with decay constant λ1 and B decuys to C with decay constants λ2. Initially at t=0, number of nuclei of A and B are 2N0 and N0 respectively. At some instant t=t0, number of nuclei of B stop changing. Find t0 if at this intant number of nuclei of B is 3N02
View SolutionA proton, a deuteron and an a particle having same momentum enter a uniform magnetic field at right angles to the field. Then the ratio of their angular momenta during their motion in the magnetic field is
View SolutionRadius of the nucleus of the atom with A=216 is (R0 =1.3fm)
View SolutionNuclei of a radioactive element X are being produced at a constant rate K and this element decays to a stable nucleus Y with a decay constant lambda and half-life T_(1//3) . At the time t=0 , there are N_(0) nuclei of the element X . The number N_(X) of nuclei of X at time t=T_(1//2) is .
View SolutionNuclei of a radioactive element X are being produced at a constant rate K and this element decays to a stable nucleus Y with a decay constant lambda and half-life T_(1//3) . At the time t=0 , there are N_(0) nuclei of the element X . The number N_(Y) of nuclei of Y at t=T_(1//2) is.
View SolutionConsider radioactive decay of A to B with which further decays either to X or Y , lambda_(1), lambda_(2) and lambda_(3) are decay constant for A to B decay, B to X decay and Bto Y decay respectively. At t=0 , the number of nuclei of A,B,X and Y are N_(0), N_(0) zero and zero respectively. N_(1),N_(2),N_(3) and N_(4) are the number of nuclei of A,B,X and Y at any instant t . The net rate of accumulation of B at any instant is
View SolutionConsider radioactive decay of A to B with which further decays either to X or Y, λ1,λ2 and λ3 are decay constant for A to B decay, B to X decay and Bto Y decay respectively. At t=0, the number of nuclei of A,B,X and Y are N0,N0 zero and zero respectively.
N1,N2,N3 and N4 are the number of nuclei of A,B,X and Y at any instant t.
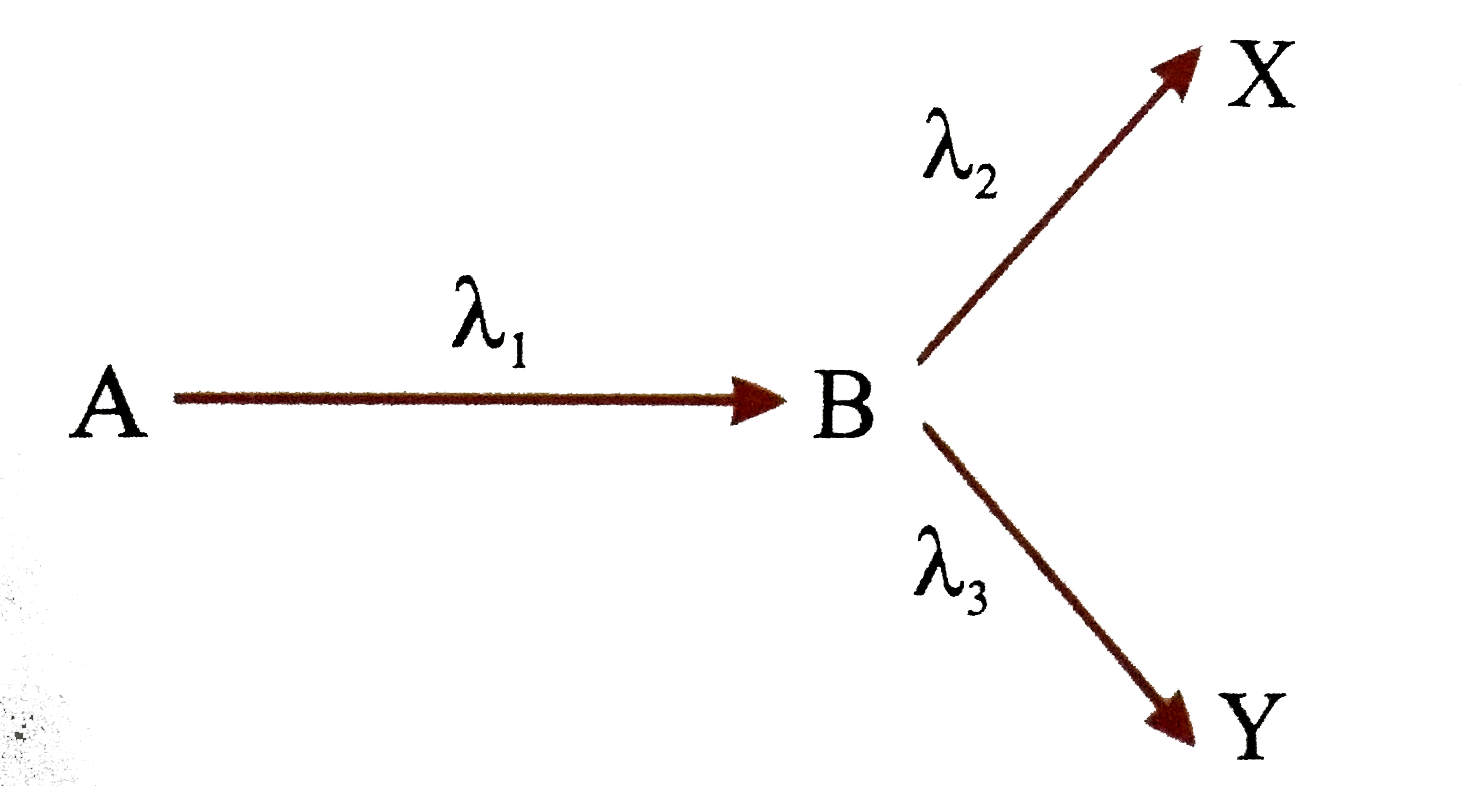
At t=∞, which of following is incorrect ?
View SolutionNucleus A decays to B with decay constant λ1 and B decays to C with decay constant λ2. Initially at t=0 number of nuclei of A and B are 2N0 and N0 respectively. At t=to, no. of nuclei of B is 3N02 and nuclei of B stop changing. Find t0?
View SolutionTwo radioactive materials A & B have decay constant 3lamda and 2lamda respectively. At t=0 the numbers of nuclei of A and B are 4N_(0) and 2N_(0) respectively then,
View SolutionA radioactive element A of decay constant lamda_(A) decays into another radioactive element B of decay constant lamda_(B) . Initially the number of active nuclei of A was N_(0) and B was absent in the sample. The maximum number of active nuclei of B is found at t=2. In 2//lamda_(A) . The maximum number of active nuclei of B is
View SolutionNucleus A decays into B with a decay constant λ1 and B further decays into C with a decay constant λ2 . Initially, at t = 0, the number of nuclei of A and B were 3N0 and N0 respectively. If at t = t0 number of nuclei of B becomes constant and equal to 2N0, then
View SolutionNucleus A decays to B with decay constant λ1 and B decuys to C with decay constants λ2. Initially at t=0, number of nuclei of A and B are 2N0 and N0 respectively. At some instant t=t0, number of nuclei of B stop changing. Find t0 if at this intant number of nuclei of B is 3N02
View SolutionNuclei of radioactive element X. are being produced at a constant rute .K. and this element decays to a stable nucleus .Y. with decay constant .lamda. with half lite ..t_(0).. . At time t=0, there are ..N.._(0).. number of nucle of the element .X. then The number N_(x) of nuclei of .X. at time t=t_(0) is
View Solution
