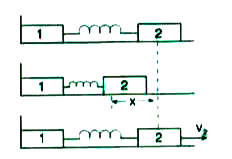
Two bars of masses .m1. and .m2. connected by a weightles spring of stiffness constant K as shown in figure, rest on a smooth horizontal plane. Bar 2 is shifted through a small distance x to the left and then released. Find the velocity of the centre of mass of the system after bar 1 breaks off the wall.
 Solution in Telugu
Solution in Telugu
Velocity of the bar 2 at the instant of break off will be obtained as 12m2v22=12kx2
v2=x√km2
The velocity of the bar 1 at that instant is zero (v1=0)
The velocity of the centre of mass is given by
Vc=(m1v1+m2v2)(m1+m2)
After the substitution of the value of v1 and v2, we get
Vc=x√km2(m1+m2)
Topper's Solved these Questions
- WORK POWER ENERGYBOOK - AAKASH SERIESCHAPTER - WORK POWER ENERGYEXERCISE - EXERCISE - 1A
117 Videos
- WORK POWER ENERGYBOOK - AAKASH SERIESCHAPTER - WORK POWER ENERGYEXERCISE - EXERCISE - 1B
39 Videos
- WORK -POWER-ENERGYBOOK - AAKASH SERIESCHAPTER - WORK -POWER-ENERGYEXERCISE - PRACTICE SHEET (ADVANCED) (STRAIGHT OBJECTIVE TYPE QUESTIONS)
36 Videos
- WORK, POWER & ENERGY BOOK - AAKASH SERIESCHAPTER - WORK, POWER & ENERGY EXERCISE - PRACTICE EXERCISE (PERFECTLY INELASTIC COLLISIONS)
5 Videos
Similar Questions
If l1,m1,n1 and l2,m2,n2 are the direction cosines of two vectors and θ is the angle between them, then the value of cos θ is
View Solution(A): A spring has potential energy, both when it is compressed or stretched.
(R) : In compressing or stretching, work is done on the spring against the restoring force.
View SolutionThe velocity of a particle of mass 10−2kg is given as →v=(2xˆu+3yˆj+4zˆk) m/sec (where x, y and z are in meter). The amount of work done by applied force in displacing a particle from (1m, 2m, 0) to (-1m, 3m, 1m) is …..
View SolutionA shot moving with a velocity 140ms−1 collides a wooden block and comes to rest in it. If mass of the block is 13 times the mass of the shot, velocity of the block is
View SolutionTwo bars of masses m_1 and m_2 connected by a weightless spring of stiffness k , rest on a smooth horizontal plane. Bar 2 is shifted by a small distance x_0 to the left and released. The veloicyt of the centre of mass of the system when bar 1 breaks off the wall is
View SolutionTwo blocks of masses m1 and m2, connected by a weightless spring of stiffness k rest on a smooth horizontal plane as shown in Fig. Block 2 is shifted a small distance x to the left and then released. Find the velocity of centre of mass of the system after block 1 breaks off the wall.

View SolutionTwo blocks of mass m1 and m2 (m1<m2) are connected with an ideal spring on a smooth horizontal surface as shown in figure. At t = 0 m1 is at rest and m2 is given a velocity v towards right. At this moment, spring is in its natural length. Then choose the correct alternative :

View SolutionTwo bars of masses m1 and m2 connected by a weightless spring of stiffness ϰ (figure) rest on a smooth horizontal plane.

Bar 2 is shifted a small distance x to the left and then released. Find the velocity of the centre of inertia of the system after bar 1 breaks off the wall.
View SolutionTwo bars of masses m_(1) and m_(2) connected by a non - deformed light spring rest on a horizontal plane. The coefficient of friction between the bars and the surface is equal to mu . What minimum constant force has to be appled in the horizontal direction to the bar of mass m_(1) in order to shift the other bar?
View SolutionTwo blocks of masses m_(1) and m_(2) are kept on a smooth horizontal table as shown in figure. Block of mass m_(1) (but not m_(2) is fastened to the spring. If now both the blocks are pushed to the left so that the spring is compressed a distance d. The amplitude of oscillatin of block of mass m_(1) after the system is released is
View SolutionTwo bars of masses m_(1) and m_(2) , connected by light underformed horizontal spring are lying on a uniformly rough horizontal surface, having coefficient of friction mu . The minimum constant force that has to be applied horizontal to the bar m_(1) along the length of the spring, in order to just shift the other bar m_(2) is
View SolutionTwo masses m1 and m2 are connected by a spring of force constant k and are placed on a frictionless horizontal surface. Initially the spring is stretched through a distance x0, when the system is released from rest. Find the distance moved by two masses before they again comes to rest.
View SolutionTwo masses m1 and m2 are connected by a spring of force constant k and are placed on a frictionless horizontal surface. Initially the spring is stretched through a distance x0, when the system is released from rest. Find the distance moved by two masses before they again comes to rest.
View SolutionTwo bars of masses .m_(1). and m_(2) . connected by a weightless spring of stiffness constant K as shown in figure, rest on a smooth horizontal plane. Bar 2 is shifted through a small distance x to the left and then released. Find the velocity of the centre of mass of the system after bar 1 breaks off the wall.
View SolutionTwo masses m_(1) and m_(2) are connected by a spring of force constant k and are placed on a frictionless horizontal surface. Initially the spring is stretched through a distance x_(0) , when the system is released from rest. Find the distance moved by two masses before they again comes to rest.
View Solution
