A steel wire of length im, mass 0.1kg and uniform cross sectional area 10−6m2 is rigidly fixed at both ends. The temperature of the wire is lowered by 20∘C. If the transverse waves are set up plucking the string in the middle, calculate the frequency of the fundamental mode of vibration. (Y=2×1011N/m2,a=1.21×10−5/∘C)Solution in Telugu
22 Hz
33 Hz
44 Hz
11 Hz
The correct Answer is:D
Step by step video, text & image solution for A steel wire of length im, mass 0.1kg and uniform cross sectional area 10^(-6) m^(2) is rigidly fixed at both ends. The temperature of the wire is lowered by 20^(@)C. If the transverse waves are set up plucking the string in the middle, calculate the frequency of the fundamental mode of vibration. (Y=2xx10^(11)N//m^(2), a=1.21xx10^(-5)//^(@)C) by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- WAVESBOOK - AAKASH SERIESCHAPTER - WAVESEXERCISE - EXERCISE-III (Velocity of Sound :)
4 Videos
- WAVESBOOK - AAKASH SERIESCHAPTER - WAVESEXERCISE - EXERCISE-III (Pipes :)
5 Videos
- WAVESBOOK - AAKASH SERIESCHAPTER - WAVESEXERCISE - EXERCISE-III (Wave Equations & Basics)
7 Videos
- WAVE OPTICSBOOK - AAKASH SERIESCHAPTER - WAVE OPTICSEXERCISE - PROBLEMS (LEVEL - II)
20 Videos
- WAVES OPTICSBOOK - AAKASH SERIESCHAPTER - WAVES OPTICSEXERCISE - EXERCISE -III (POLARISITION)
10 Videos
Similar Questions
Two monoatomic ideal gases 1 and 2 of molecules masses m1andm2respectively are enclosed in separate container kept at the same temperature. The ratio of the speed of sound in gas I to that in gas '2' is given by
View SolutionAn open pipe is suddenly closed at one end as a result, frequency of third harmonic of the closed pipe is found to be higher by 100 Hz than the fundamental frequency of the open pipe. The fundamental frequency of the open pipe is
View SolutionA transverse wave of amplitude 0.5m, wavelength 1m and frequency 2 Hz is propagating in a string in the negative x direction. The equation of this wave is
View SolutionIn Young's double slit experiment the y co-ordinates of central maxima and 10th maxima are 2 cm and 5 cm respectively. When the YDSE apparatus is immersed in a liquid of refractive index 1.5. Find the corresponding y co-ordinates.
View SolutionA ray of light is incident on the surface of a glass plate of refractive index √3 at the polarising angle . The angle of refraction of the ray is
View SolutionA steel wire of length 1m , mass 0.1kg and uniform cross-sectional area 10^(-6)m^(2) is rigidly fixed at both ends. The temperature of the wire is lowered by 20^(@)C . If transverse calculate the frequency of the fundamental mode of vibration. Given for steel Y = 2 xx 10^(11)N//m^(2) alpha = 1.21 xx 10^(-5) per ^(@)C
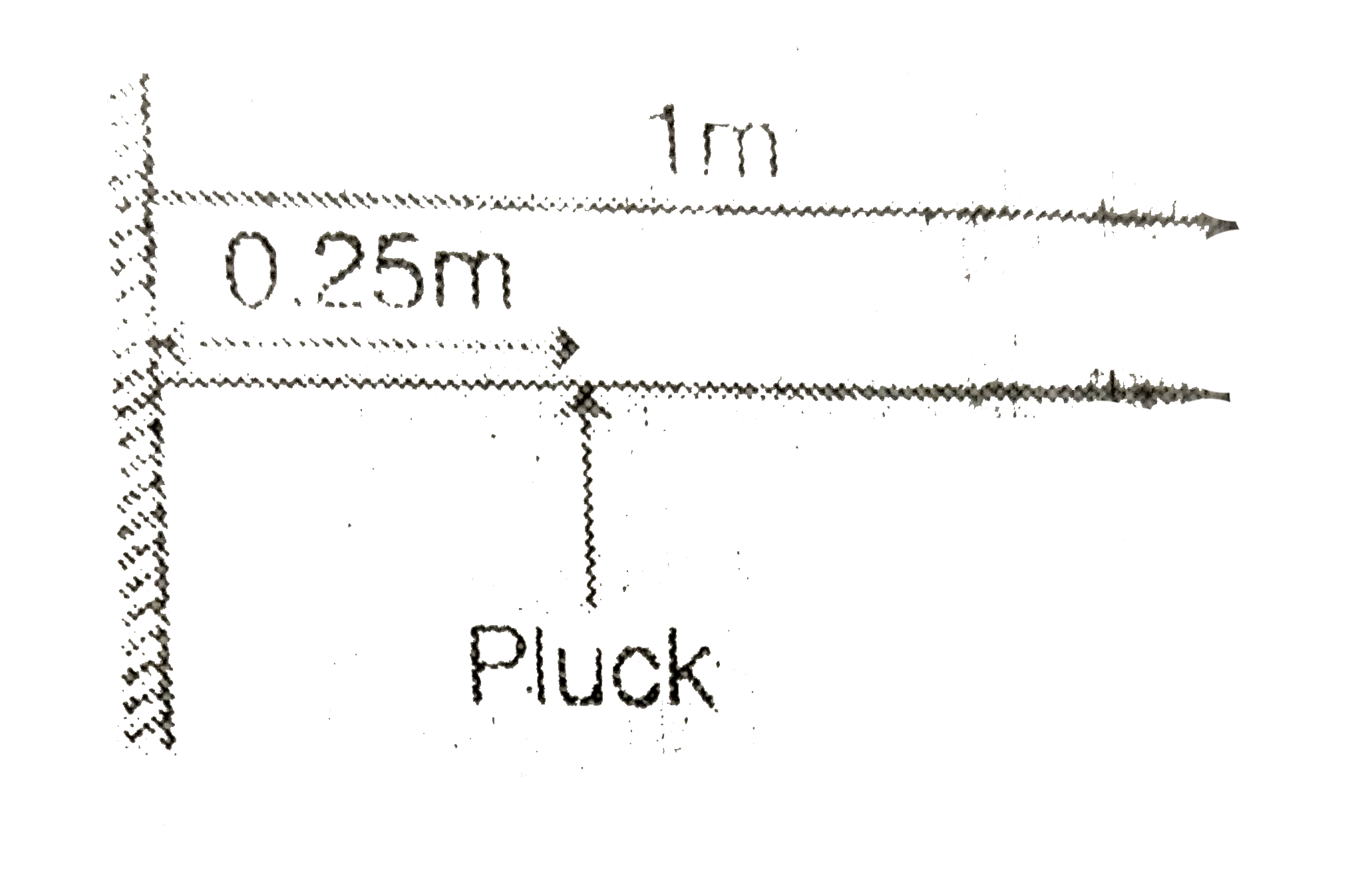
View SolutionA steel wire is rigidly fixed at both ends its length mass and cross sectional area are 1m, 0.1 kg and 106m2 respectively then the temperature of the wire is lowered by 20∘C if the transverse waves are setup by plucking the wire at 0.25m from one end and assuming that wire vibrates with minimum number of loops possible for such a case. Find the frequency of vibration (in Hz). [coefficient of linear expansion of steel =1.21×10−6/.∘C and young's modulus =2×1011/N/m2]
View SolutionA steel wire of length 20 cm and uniform cross-section 1mm^(2) is tied rigidly at both the ends. If the temperature of the wire is altered from 40^(@)C to 20^(@)C , the change in tension. [Given coefficient of linear expansion of steel is 1.1xx10^(5) .^(@)C^(-1) and Young's modulus for steel is 2.0xx10^(11) Nm^(-2) ]
View SolutionA steel wire of length 1 m and mass 0.1 kg and having a uniform cross-sectional area of 10^(-6) m^(2) is rigidly fixed at both ends. The temperature of the wire is lowered by 20^(@)C . If the wire is vibrating in fundamental mode, find the frequency (in Hz). (Y_(steel)=2xx 10^(11) N//m^(2),alpha_(steel)=1.21 xx 10^(-5)//.^(@)C) .
View SolutionA steel wire is rigidly fixed at both ends. Its length mass and cross-sectionl area are 1m, 0.1kg and 10−8m2 respectively. Tension in the wire is produced by lowering the temperature by 20∘C. If the transerverse waves are some up by plucking the wire at 0.25m from one end and assuming that the wire viberates with minimum number of loops possble for such a case. The frequency of viberation (in Hz) is found to be. 1.11. Find the value of K. Given α=1.21×10−5.∘C−1.Y−2×1011N/m2
View Solutionस्टील का एक तार जिसकी लम्बाई 1 मीटर, द्रव्यमान 0.1 किग्रा तथा एकसमान परिच्छेद-क्षेत्रफल 10−6 मीटर2 है, दोनों सिरों पर दृढ़तापूर्वक बँधा है। तार का ताप 20∘C कम कर दिया जाता है। यदि तार को मध्य में कर्षित करके (by plucking) अनुप्रस्थ तरंगें उत्पन्न की जायें, तो कम्पन की मूल विधा की आवृत्ति की गणना कीजिए। स्टील का यंग-प्रत्यास्थता गुणांक =2×1011 न्यूटन/मीटर2, स्टील का रेखीय प्रसार गुणांक =1.21×10−5 प्रति∘C.
View SolutionA steel wire of length 2.5 m and area of cross section 1.25mm2, when it is stretched by a force of 40 N and Y for steel is 2×1011N/m2. The extension produced in a steel wire is
View SolutionA steel wire of length 0.20m and uniform cross section 10^(-6)m^(2) is tied rigidly at both ends. The temperature of the wire is changed from 40^(@) C to 20^(@)C . Calculate the thermal tension in the wire given alpha =1.1. xx 10^(-5)//.^(@)C and Y=2xx10^(11)Nm^(-2)
View SolutionA steel wire of length 1 m, mass 0.1 kg and uniform cross sectional area 10^(-6)m^(2) is rigidly fixed at two ends. The temperature of the wire is lowered by 20^(@) C . If transverse waves are now set up by lucking the string at the middle, calculate the frequency of the fundamental tone. Givne, for steel, co-efficient of linear expansion = 1.21 xx 10^(-5_(@))C^(1) and Young's modulus = 2 xx 10 ^(11) N* m^(-2) .
View SolutionA steel wire of length 20 cm and cross - section 1 mm^(2) is tied rigidly at both ends at room temperature 30^(@)C . If the temperature falls 10^(@)C , what will be the change in tension ? Given that Y = 2xx10^(11)N//m^(2) and alpha = 1.1xx10^(-5)//^(@)C
View Solution
