Which is greater μs or μk?
To determine which is greater between the coefficient of static friction (μ_s) and the coefficient of kinetic friction (μ_k), we can analyze the definitions and the physical principles behind these coefficients.
1. Definition of Coefficients:
- The coefficient of static friction (μ_s) is defined as the ratio of the maximum static frictional force (F_s) that must be overcome to start moving an object at rest to the normal force (N) acting on the object.
- The coefficient of kinetic friction (μ_k) is defined as the ratio of the kinetic frictional force (F_k) acting on a moving object to the normal force (N).
μs=FsNandμk=FkN
2. Understanding Friction Forces:
- Static friction acts on an object that is not moving, and it can vary up to a maximum value (F_s) before the object starts to move.
- Kinetic friction acts on an object that is already in motion and is generally constant for a given pair of surfaces.
3. Physical Interaction:
- The reason μ_s is greater than μ_k can be attributed to the nature of the interactions between the surfaces in contact.
- When two surfaces are at rest relative to each other, the microscopic irregularities and adhesive forces between the surfaces can create a stronger frictional force.
- Once the object starts moving, these interactions are reduced, leading to a lower frictional force, hence a lower coefficient of kinetic friction.
4. Conclusion:
- Based on the definitions and the physical interactions, it is established that:
μs>μk
- Therefore, the coefficient of static friction (μ_s) is greater than the coefficient of kinetic friction (μ_k).
- The coefficient of static friction (μ_s) is defined as the ratio of the maximum static frictional force (F_s) that must be overcome to start moving an object at rest to the normal force (N) acting on the object.
- The coefficient of kinetic friction (μ_k) is defined as the ratio of the kinetic frictional force (F_k) acting on a moving object to the normal force (N).
- Static friction acts on an object that is not moving, and it can vary up to a maximum value (F_s) before the object starts to move.
- Kinetic friction acts on an object that is already in motion and is generally constant for a given pair of surfaces.
- The reason μ_s is greater than μ_k can be attributed to the nature of the interactions between the surfaces in contact.
- When two surfaces are at rest relative to each other, the microscopic irregularities and adhesive forces between the surfaces can create a stronger frictional force.
- Once the object starts moving, these interactions are reduced, leading to a lower frictional force, hence a lower coefficient of kinetic friction.
- Based on the definitions and the physical interactions, it is established that:
μs>μk
- Therefore, the coefficient of static friction (μ_s) is greater than the coefficient of kinetic friction (μ_k).
Topper's Solved these Questions
Similar Questions
A particle is at rest on a rough horizontal plane . The plane is slowly titled until the particle starts to move and is then kept fixed . If the static and kinetic coefficients of friction are μs and μk(<μs) respectively , show that the velocity of the particle after it has travelled distance d is given by :
v= ⎷2(μs−μk)gd(1+μ2s)1/2
View SolutionA block of mass m rests on a rough horizontal plane having coefficient of kinetic friction μk and coefficient of static friction μs. The spring is in its natural, when a constant force of magnitude P=5μkmg4 starts acting on the block. The spring force F is a function of extension x as F=kx2.( Where k is spring constant )
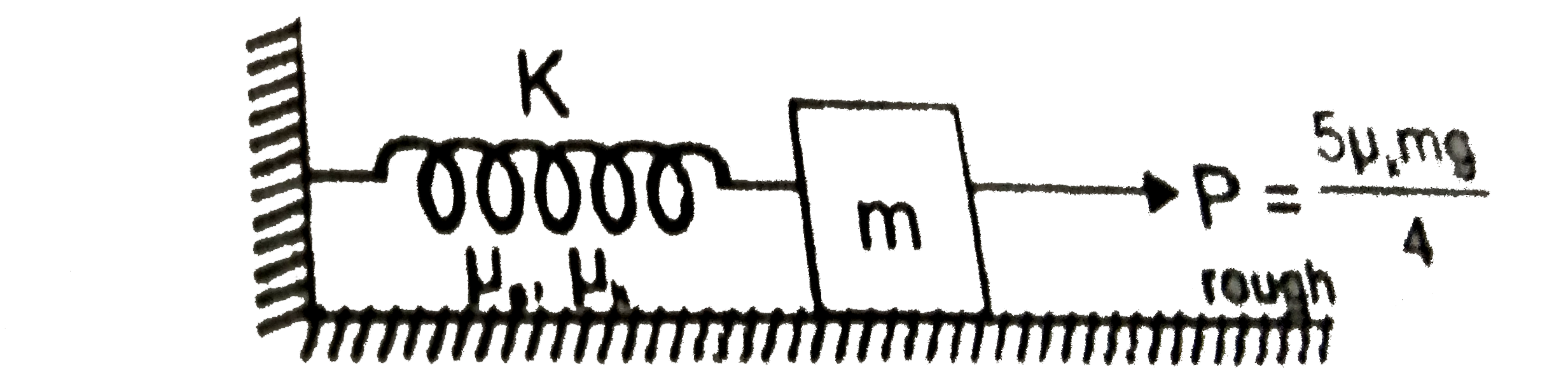
(a) Comment on the relation between μs and μk for the motion to start.
(b) Find the maximum extension in the spring ( Assume the force P is sufficient make the block move ).
View Solution
Knowledge Check
Which sample contains greater number of nuclei ?
a 5.00−μCi sample of .240Pu (half-life 6560y)
or a4.45−μCi sample of .243Am (half-life 7370y).A.240PuB.243AmCEqual in bothDNone of theseAssetion: Carbon dioxide has greater value of root mean square velocity μrms than carbon monoxide.
Reason: μrms is inversely proportional to molar mass.AIf both assertion and reason are true and the reason is the correct explanation of the assertion.BIf both assertion and reason are true but reason is not the correct explanation of the assertion.CIf assertion is true but reason is false.DIf assertion is false but reason is true.A sufficiently long plane of mass 4kg is placed on a smooth horizontal surfaces A small block of mass 2kg is placed over the plank and is beging acted upon by a time verying horizontal force F=(0.5t) where F is in newton and t is in second as shown in figure. The coefficient of friction slipping the plank and the block is given is μs=μk=μ time t=12s the relative slipping between the plank and the block is just likely to accor

The coefficient of friction μ is equal toA0.10B0.15C0.20D0.30
The magnetic moment vectors μsandμl associated with the intrinsic spin angular momentum →S and orbital angular momentum →l respectively, of an electron are predicated by quantum theory (and verified experimentally to a hing accuracy ) to be given by :
μs=−(em)→S,μI=−(e2m)→I
Which of these relations is in accordance with the result expected classically ? Outline the derivation of the classical result .
View SolutionRepeat the above problem, if instead of μ, we are given μs and μk,where μs=0.6 and μk=0.4.
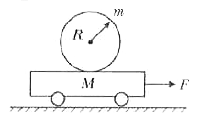
View SolutionA sphere of mass m and radius R is kept on a trolley of mass Mas shown in the figure. The coefficient of static and kinetic friction between the sphere and the trolley are μsandμk respectively. The maximum horizontal force F that can be applied to the trolley for which the solid sphere does not slip is
View SolutionIn YDSE if a slab whose refractive index can be varied is placed in front of one of the slits. Then, the variation of resultant intensity at mid-point of screen with μ will be best represented by (mu is greater than or equal to 1)
View SolutionWhich is true for rolling friction (μr), static friction (μs) and kinetic friction (μk) ?
View Solution
