When a projectile is projected with velocity (v) making an angle $θ$ with the horizontal direction, then maximum horizontal range is…………………………… .

Video Solution

|

Answer

Step by step video & image solution for When a projectile is projected with velocity (v) making an angle theta with the horizontal direction, then maximum horizontal range is…………………………… . by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.

Updated on:21/07/2023

Class 11 PHYSICS PROJECTILE MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
When a body is thrown with a velocity u making an angle $θ$ with the horizontal plane, the maximum distance covered by it in horizontal direction is
A $\frac{u^{2} sin θ}{g}$
B $\frac{u^{2} sin 2 θ}{2 g}$
C $\frac{u^{2} sin 2 θ}{g}$
D $\frac{u^{2} cos 2 θ}{g}$
Question 2 - Select One
A projectile is thrown with velocity $u$ making an angle $θ$ with the horizontal. Its time of flight on the horizontal ground is 4 second. The projectile is moving at angle of $45^{\circ}$ with the horizontal just one second after the projection. Hence the angle $θ$ is (take $g = 10 m / s^{2}$ )
A ${tan}^{- 1} (4)$
B $60^{\circ}$
C $53^{\circ}$
D ${tan}^{- 1} (2)$
Question 3 - Select One or More
A projectile is projected with a velocity $v_{0}$ at an angle $θ$ with the horizontal as shown in figure. The angular momentum of particle about the origin:

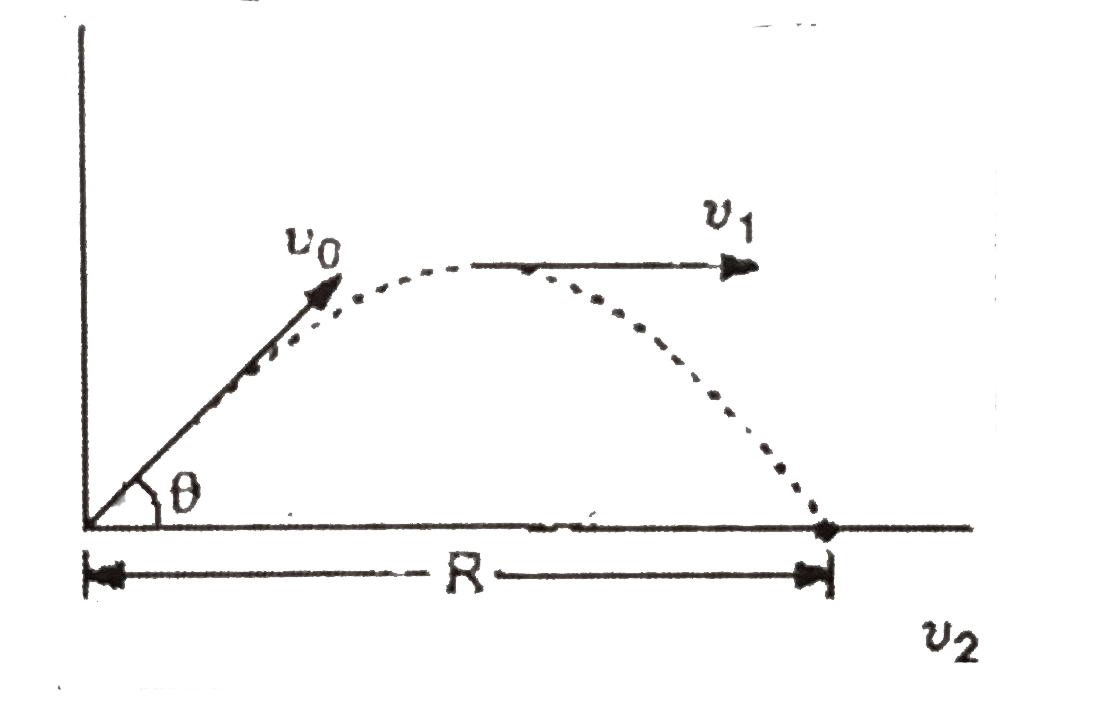Ais zero when particle is at the origin
Bis $\frac{- m v^{3} {sin}^{2} θ cos θ}{2 g} ˆ k$ when particle is at the highest point of trajectory
Cis $\frac{- 2 m v^{2} {sin}^{2} θ cos θ}{g} ˆ k$ when particle is just about to hit ground
Ddownward force of gravity exerts a torque in $- z$ direction.

SL ARORA-Motion in a Plane-Excercise

Home
Ask Doubt
Profile