Find the first excitation potential of He+ ion (a)Find the ionization potential of Li++ ion
The correct Answer is:A, B, D
To solve the given problem, we need to find the first excitation potential of the He+ ion and the ionization potential of the Li++ ion.
Step 1: Find the first excitation potential of He+ ion
1. Understand the formula for potential energy of hydrogen-like atoms:
The potential energy \( V \) of a hydrogen-like atom in the \( n \)th state is given by:
\(
V = -\frac{13.6 \, Z^2}{n^2} \text{ eV}
\)
where \( Z \) is the atomic number.
2. Identify the transition for excitation:
For the first excitation of He+, the transition is from \( n = 1 \) to \( n = 2 \).
3. Calculate the potential energy for \( n = 1 \):
\(
V_1 = -\frac{13.6 \times 2^2}{1^2} = -\frac{13.6 \times 4}{1} = -54.4 \text{ eV}
\)
4. Calculate the potential energy for \( n = 2 \):
\(
V_2 = -\frac{13.6 \times 2^2}{2^2} = -\frac{13.6 \times 4}{4} = -13.6 \text{ eV}
\)
5. Find the first excitation potential:
The first excitation potential is the difference in potential energy between these two states:
\(
\text{Excitation Potential} = V_2 - V_1 = -13.6 - (-54.4) = 40.8 \text{ eV}
\)
Step 2: Find the ionization potential of Li++ ion
1. Use the same formula for ionization potential:
The ionization potential for hydrogen-like atoms is also given by:
\(
\text{Ionization Potential} = -V_1 = \frac{13.6 \, Z^2}{1^2}
\)
2. Identify \( Z \) for Li++:
For Li++, \( Z = 3 \).
3. Calculate the ionization potential:
\(
\text{Ionization Potential} = 13.6 \times 3^2 = 13.6 \times 9 = 122.4 \text{ eV}
\)
Final Answers:
- The first excitation potential of He+ is 40.8 eV.
- The ionization potential of Li++ is 122.4 eV.
---
The potential energy \( V \) of a hydrogen-like atom in the \( n \)th state is given by:
\(
V = -\frac{13.6 \, Z^2}{n^2} \text{ eV}
\)
where \( Z \) is the atomic number.
For the first excitation of He+, the transition is from \( n = 1 \) to \( n = 2 \).
\(
V_1 = -\frac{13.6 \times 2^2}{1^2} = -\frac{13.6 \times 4}{1} = -54.4 \text{ eV}
\)
\(
V_2 = -\frac{13.6 \times 2^2}{2^2} = -\frac{13.6 \times 4}{4} = -13.6 \text{ eV}
\)
The first excitation potential is the difference in potential energy between these two states:
\(
\text{Excitation Potential} = V_2 - V_1 = -13.6 - (-54.4) = 40.8 \text{ eV}
\)
The ionization potential for hydrogen-like atoms is also given by:
\(
\text{Ionization Potential} = -V_1 = \frac{13.6 \, Z^2}{1^2}
\)
For Li++, \( Z = 3 \).
\(
\text{Ionization Potential} = 13.6 \times 3^2 = 13.6 \times 9 = 122.4 \text{ eV}
\)
- The first excitation potential of He+ is 40.8 eV.
- The ionization potential of Li++ is 122.4 eV.
Topper's Solved these Questions
- BOHR'S MODEL AND PHYSICS OF THE ATOMBOOK - HC VERMA ENGLISHCHAPTER - BOHR'S MODEL AND PHYSICS OF THE ATOMEXERCISE - Short Answer
10 Videos
- BOHR'S MODEL AND PHYSICS OF THE ATOMBOOK - HC VERMA ENGLISHCHAPTER - BOHR'S MODEL AND PHYSICS OF THE ATOMEXERCISE - Obejective - II
5 Videos
- ALTERNATING CURRENTBOOK - HC VERMA ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Short answer
14 Videos
- CAPACITORSBOOK - HC VERMA ENGLISHCHAPTER - CAPACITORSEXERCISE - short answer
7 Videos
Similar Questions
First ionization potential of Be and B will be :
View SolutionThe energy level excitation potential of a hydrogen -like atom is shown in Figure
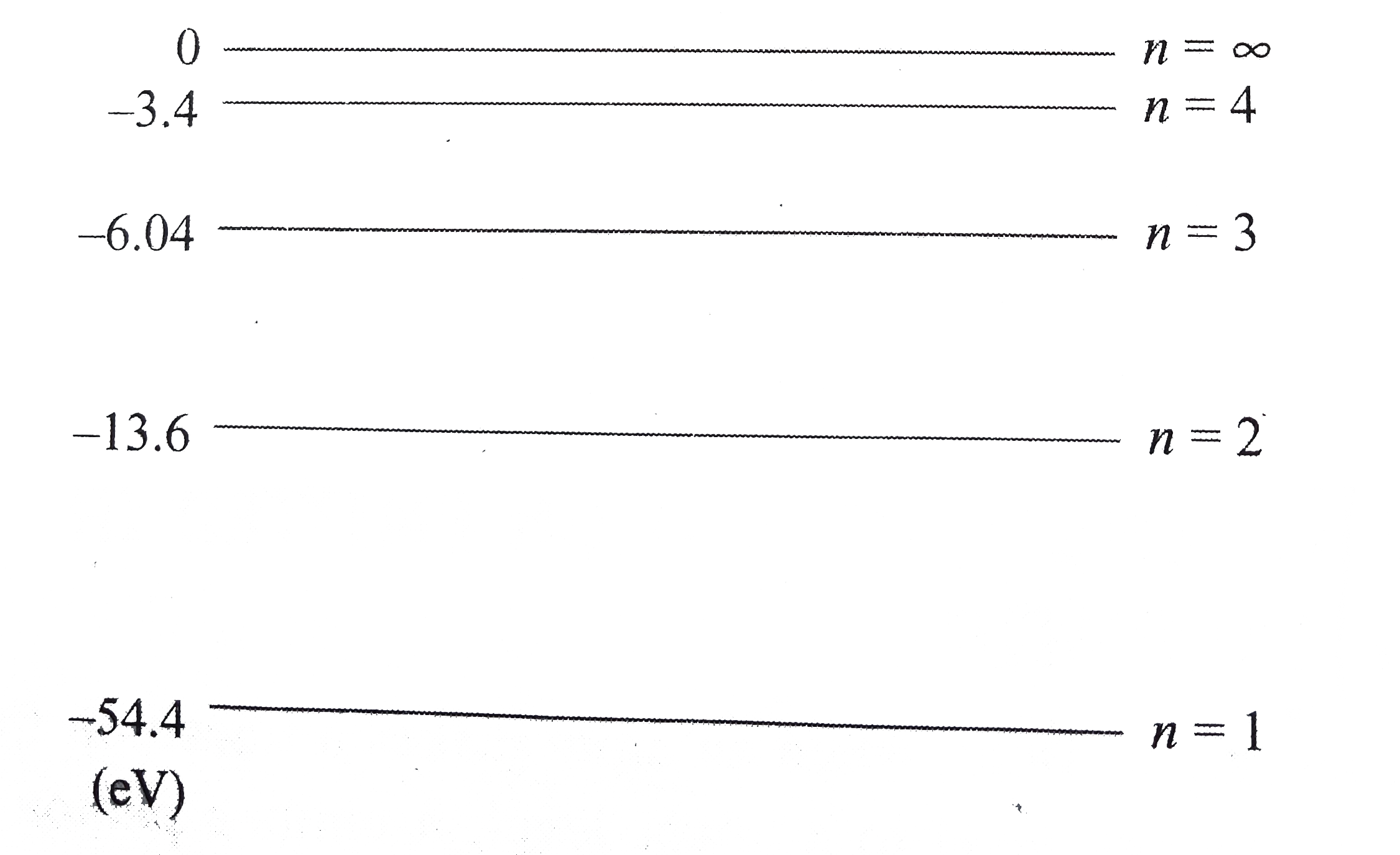
a Find the value of Z
b If initially the atom is in the ground state ,then
(i) determine its excitation potential, and
m (ii) determine its ionization potential.
c Can it absorbe a photom of 42eV?
d Can it absorbe a photom of 56eV?
e Calculate the radius of its first Bohr orbit.
f Calculate the kinectic and potential energy of an electron in the first orbit.
View Solution
Knowledge Check
If the ionisation potential for hydrogen atom is 13.6 eV, then the ionisation potential for He+ ion should be
A27.2 eVB54.4 eVC6.8 eVD13.6 eV.
Define the following term - Ionization potential
View SolutionWhich has maximum first ionization potential?
View SolutionThe first ionization potential (eV) of N and P respectively are
View SolutionIonization energy of He+ ion at minimum energy position is
View SolutionIf first excitation potential of a hydrogen-like atom is V electron volt, then the ionization energy of this atom will be:
View SolutionThe ionisation potentials of Li and K are 5.4 and 4.3 eV respectively. The ionization potential of Na will be:
View SolutionThe second ionization potential of elements is invariable higher than first ionization potential because:
View Solution
