suppose the ends of the coil in the previous problem are connected to a resistane of 100Ω. Neglecting the resistance of the coil, find the heat produced in the circuit in one minute.
To solve the problem of finding the heat produced in the circuit in one minute when the ends of a coil are connected to a resistance of 100Ω, we can follow these steps:
Step 1: Understand the Formula for Heat
The heat produced in a resistor can be calculated using the formula:
H=I2Rt
where:
- H is the heat produced,
- I is the current through the resistor,
- R is the resistance,
- t is the time in seconds.
Step 2: Calculate the Electromotive Force (EMF)
The induced EMF (E) in the coil can be calculated using Faraday's law of electromagnetic induction. The formula for the induced EMF when a coil is rotated in a magnetic field is:
E=−dΦdt
where Φ is the magnetic flux given by:
Φ=BAcos(ωt)
Here, B is the magnetic field strength, A is the area of the coil, and ω is the angular frequency.
Step 3: Determine the Area of the Coil
The area A of a circular coil is given by:
A=πr2
Given that the radius r=5cm=0.05m:
A=π(0.05)2=π×0.0025≈0.00785m2
Step 4: Calculate Angular Frequency
The angular speed ω can be calculated from the revolutions per minute (RPM):
ω=2π×(RPM)/60
Given that the coil rotates at 80 RPM:
ω=2π×8060=8π3rad/s
Step 5: Calculate the Induced EMF
Using the formula for EMF:
E=BAω
Substituting the values:
- B=0.1010T
- A≈0.00785m2
- ω=8π3rad/s
Calculating E:
E=0.1010×0.00785×8π3≈0.1010×0.00785×8.3776≈0.00067V
Step 6: Calculate the Current
Using Ohm's law, the current I can be calculated as:
I=ER
Substituting R=100Ω:
I=0.00067100≈6.7×10−6A
Step 7: Calculate the Heat Produced in One Minute
Now substituting I, R, and t=60s into the heat formula:
H=I2Rt=(6.7×10−6)2×100×60
Calculating H:
H=(4.489×10−11)×100×60≈2.6934×10−9J
Final Answer
The heat produced in the circuit in one minute is approximately:
H≈2.69×10−9J
The heat produced in a resistor can be calculated using the formula:
H=I2Rt
where:
- H is the heat produced,
- I is the current through the resistor,
- R is the resistance,
- t is the time in seconds.
The induced EMF (E) in the coil can be calculated using Faraday's law of electromagnetic induction. The formula for the induced EMF when a coil is rotated in a magnetic field is:
E=−dΦdt
where Φ is the magnetic flux given by:
Φ=BAcos(ωt)
Here, B is the magnetic field strength, A is the area of the coil, and ω is the angular frequency.
The area A of a circular coil is given by:
A=πr2
Given that the radius r=5cm=0.05m:
A=π(0.05)2=π×0.0025≈0.00785m2
The angular speed ω can be calculated from the revolutions per minute (RPM):
ω=2π×(RPM)/60
Given that the coil rotates at 80 RPM:
ω=2π×8060=8π3rad/s
Using the formula for EMF:
E=BAω
Substituting the values:
- B=0.1010T
- A≈0.00785m2
- ω=8π3rad/s
E=0.1010×0.00785×8π3≈0.1010×0.00785×8.3776≈0.00067V
Using Ohm's law, the current I can be calculated as:
I=ER
Substituting R=100Ω:
I=0.00067100≈6.7×10−6A
Now substituting I, R, and t=60s into the heat formula:
H=I2Rt=(6.7×10−6)2×100×60
Calculating H:
H=(4.489×10−11)×100×60≈2.6934×10−9J
The heat produced in the circuit in one minute is approximately:
H≈2.69×10−9J
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - HC VERMA ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Questions for short answer
9 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - HC VERMA ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Objective 2
10 Videos
- ELECTRIC FIELD AND POTENTIALBOOK - HC VERMA ENGLISHCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - Objective 2
7 Videos
- ELECTROMAGNETIC WAVESBOOK - HC VERMA ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Exercise
8 Videos
Similar Questions
The wire star of the previous problem is connected to the circuit at points P and C. Find the equivalent resistance.
View SolutionWhat will be the motion of the conductor of the previous problem, if a resistor of resistance R is connected into the circuit instead of the capacitor? Neglect the resistance of the conductors.
View SolutionFind the total heat produced in the loop of the previous problem during the interval 0 to 30 s if the resistance of the loop is 4.5 mOmega.
View SolutionA coil of self inductance 2.5 H and resistance 20Ω is connected to a battery of emf 120 V having internal resistance of 5Ω. Find :
The current in the circuit in steady state.
View SolutionSuppose the resistance of the coil in the previous problem is 25Ω. Assume that the coil moves with uniform velocity during its removal and restoration. Find the thermal energy developed in the coil during (a) its removal, (b) its restoration and (c) its motion.
View SolutionSixteen resistor, each resistane 16Ω are connected in the circuit as shown in fig. the net resistance between A and B is
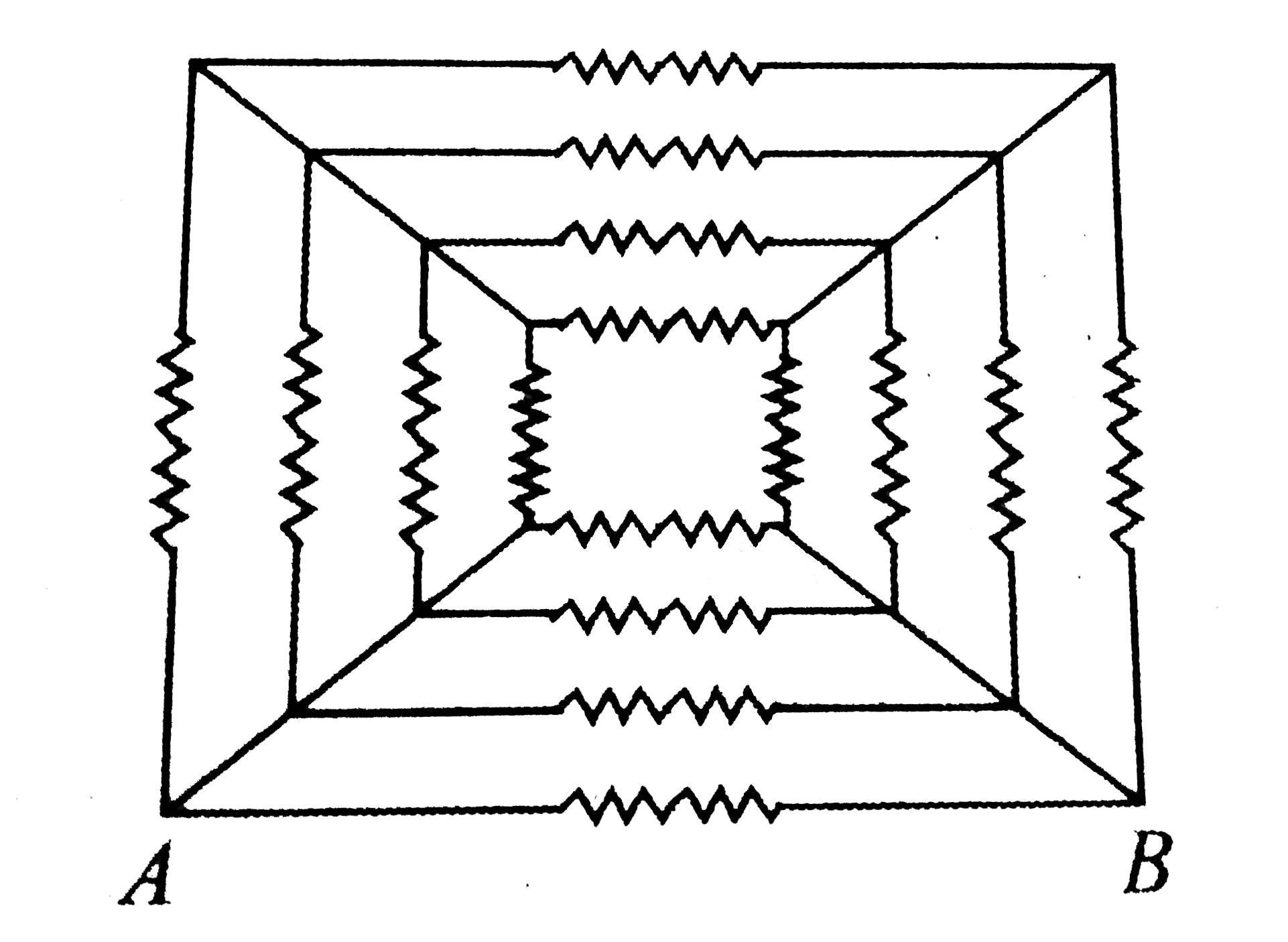
View SolutionA coil of self inductance 2.5 H and resistance 20Ω is connected to a battery of emf 120 V having internal resistance of 5Ω. Find :
The time constant of the circuit
View SolutionA 1250 W heater operates at 115V. What is the resistance of the heating coil?
View SolutionA 1250 W heater operates at 115V. What is the resistance of the heating coil?
View SolutionWhen a coil is connected to a 100 V D.C. supply, the current is 2 A. When the same coil is connected to A.C. source E=100√2sinωt, the current is 1 A. Find the inductive reactance used in the circuit.
View Solution
