Three equal charges, each having a magnitude of. 2.0×10−6C, are placed at the thre corners of a right. angled triangle of sides 3 cm, 4cm and 5 cm. find the force (in magnitude) on the charge at the right angled
To solve the problem of finding the force on the charge at the right angle of a right-angled triangle with three equal charges, we will follow these steps:
Step 1: Identify the Charges and Their Positions
Let the charges be:
- Q1=Q2=Q3=2.0×10−6C
- Place Q1 at the vertex of the triangle at (0, 0), Q2 at (3 cm, 0), and Q3 at (3 cm, 4 cm).
Step 2: Calculate the Forces Acting on the Charge at the Right Angle
We need to find the forces acting on Q2 (the charge at the right angle).
Force F21 (Force on Q2 due to Q1):
Using Coulomb's law:
F21=14πϵ0⋅Q1Q2R212
Where R12=3cm=0.03m:
F21=9×109N m2/C2⋅(2.0×10−6C)2(0.03m)2
Calculating:
F21=9×109⋅4×10−120.0009=36×10−30.0009=40N
Force F23 (Force on Q2 due to Q3):
Using Coulomb's law again:
F23=14πϵ0⋅Q2Q3R223
Where R23=4cm=0.04m:
F23=9×109⋅(2.0×10−6)2(0.04)2
Calculating:
F23=9×109⋅4×10−120.0016=36×10−30.0016=22.5N
Step 3: Calculate the Resultant Force on the Charge at the Right Angle
The forces F21 and F23 are perpendicular to each other, so we can use the Pythagorean theorem to find the resultant force F:
F=√F221+F223
Calculating:
F=√(40)2+(22.5)2=√1600+506.25=√2106.25≈45.9N
Final Answer
The magnitude of the force on the charge at the right angle is approximately 45.9N.
---
Let the charges be:
- Q1=Q2=Q3=2.0×10−6C
- Place Q1 at the vertex of the triangle at (0, 0), Q2 at (3 cm, 0), and Q3 at (3 cm, 4 cm).
We need to find the forces acting on Q2 (the charge at the right angle).
Using Coulomb's law:
F21=14πϵ0⋅Q1Q2R212
Where R12=3cm=0.03m:
F21=9×109N m2/C2⋅(2.0×10−6C)2(0.03m)2
Calculating:
F21=9×109⋅4×10−120.0009=36×10−30.0009=40N
Using Coulomb's law again:
F23=14πϵ0⋅Q2Q3R223
Where R23=4cm=0.04m:
F23=9×109⋅(2.0×10−6)2(0.04)2
Calculating:
F23=9×109⋅4×10−120.0016=36×10−30.0016=22.5N
The forces F21 and F23 are perpendicular to each other, so we can use the Pythagorean theorem to find the resultant force F:
F=√F221+F223
Calculating:
F=√(40)2+(22.5)2=√1600+506.25=√2106.25≈45.9N
The magnitude of the force on the charge at the right angle is approximately 45.9N.
Topper's Solved these Questions
- ELECTRIC FIELD AND POTENTIALBOOK - HC VERMA ENGLISHCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - Exercises
75 Videos
- ELECTRIC FIELD AND POTENTIALBOOK - HC VERMA ENGLISHCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - question for short answer
14 Videos
- ELECTRIC FIELD AND POTENTIALBOOK - HC VERMA ENGLISHCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - Objective 2
7 Videos
- ELECTRIC CURRENT THROUGH GASESBOOK - HC VERMA ENGLISHCHAPTER - ELECTRIC CURRENT THROUGH GASESEXERCISE - Short answer
6 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - HC VERMA ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Questions for short answer
9 Videos
Similar Questions
Three identical charges, each having a value 1.0×10−8C, are placed at the corners of an equilateral triangle of side 20 cm find the electric field and potential at the centre of the triangle.
View SolutionThree equal charges, 2.0×10−6 C each, are held fixed at the three corners of a square of side 5 cm. find the Coulomb force experienced by one of the charges due to the rest two.
View SolutionThree equal charge q0 each are placed at three corners of an equilateral triangle of side 'a'. Find out force acting on one of the charge due to other two charges ?
View SolutionThree point particles of masses 1.0 kg 2 kg and 3 kg are placed at three comers of a right angle triangle of sides 4.0 cm , 3.0 cm and 5.0 cm as shown in the figure . The centre of mass of the system is at point :
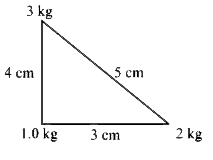
View SolutionThree point particles of masses 1.0 kg 2 kg and 3 kg are placed at three comers of a right angle triangle of sides 4.0 cm , 3.0 cm and 5.0 cm as shown in the figure . The centre of mass of the system is at point :

View SolutionThe perimeter of a right angled triangle is 60 cm. Its hypotenuse is 25 cm. Find the area of the triangle.
View SolutionFour equal charges 2.0×10−6C each are fixed at the four corners of a squae of side 5 cm. Find the coulomba force eperienced by one of the charges due to the rest three.
View SolutionIf the difference between the sides of a right angled triangle is 3 cm and its area is 54 cm2, find its perimeter.
View SolutionThree points charges of 1C,2Cand3C are placed at the corners of an equilateral triangle of side 100 cm. Find the work done to move these charges to the corners of a similar equilateral triangle of side 50 cm.
View SolutionThree points charges of 1C,2Cand3C are placed at the corners of an equilateral triangle of side 100 cm. Find the work done to move these charges to the corners of a similar equilateral triangle of side 50 cm.
View Solution
