Consider the wave y=(5mm)sin[(1cm−1)x−(60s−1)t]. Find the amplitude, b. the wave number, c the wavelength d. the frequency e. the time period and f. the wave velocity.
To solve the problem step by step, we will analyze the given wave equation and extract the required parameters.
The wave equation given is:
y=(5mm)sin((1cm−1)x−(60s−1)t)
Step 1: Find the Amplitude
The amplitude A is the coefficient in front of the sine function in the wave equation.
Solution:
A=5mm
Step 2: Find the Wave Number
The wave number k is the coefficient of x in the argument of the sine function.
Solution:
k=1cm−1
Step 3: Find the Wavelength
The wavelength λ can be calculated using the relationship between wave number and wavelength:
k=2πλ
Rearranging gives:
λ=2πk
Solution:
λ=2π1=2πcm
Step 4: Find the Frequency
The angular frequency ω is the coefficient of t in the argument of the sine function. The frequency ν can be calculated using the formula:
ν=ω2π
Solution:
ν=602π=30πHz
Step 5: Find the Time Period
The time period T is the reciprocal of the frequency:
T=1ν
Solution:
T=130π=π30s
Step 6: Find the Wave Velocity
The wave velocity v can be calculated using the formula:
v=νλ
Solution:
v=(30π)(2π)=60cm/s
Summary of Results:
- Amplitude A=5mm
- Wave number k=1cm−1
- Wavelength λ=2πcm
- Frequency ν=30πHz
- Time period T=π30s
- Wave velocity v=60cm/s
y=(5mm)sin((1cm−1)x−(60s−1)t)
The amplitude A is the coefficient in front of the sine function in the wave equation.
A=5mm
The wave number k is the coefficient of x in the argument of the sine function.
k=1cm−1
The wavelength λ can be calculated using the relationship between wave number and wavelength:
k=2πλ
Rearranging gives:
λ=2πk
λ=2π1=2πcm
The angular frequency ω is the coefficient of t in the argument of the sine function. The frequency ν can be calculated using the formula:
ν=ω2π
ν=602π=30πHz
The time period T is the reciprocal of the frequency:
T=1ν
T=130π=π30s
The wave velocity v can be calculated using the formula:
v=νλ
v=(30π)(2π)=60cm/s
- Amplitude A=5mm
- Wave number k=1cm−1
- Wavelength λ=2πcm
- Frequency ν=30πHz
- Time period T=π30s
- Wave velocity v=60cm/s
Topper's Solved these Questions
- WAVE MOTION AND WAVES ON A STRINGBook:HC VERMA ENGLISHChapter:WAVE MOTION AND WAVES ON A STRINGExercise:Worked out Examples
Explore 10 Videos
- WAVE MOTION AND WAVES ON A STRINGBook:HC VERMA ENGLISHChapter:WAVE MOTION AND WAVES ON A STRINGExercise:Objective -1
Explore 20 Videos
- THE FORCESBook:HC VERMA ENGLISHChapter:THE FORCESExercise:Questions for short Answer
Explore 9 Videos
- WORK AND ENERGYBook:HC VERMA ENGLISHChapter:WORK AND ENERGYExercise:Question for Short Answer
Explore 16 Videos
Similar Questions
Knowledge Check
The equation of a progressive wave is given by y = 5 sin(100πt−0.4πx) where y and x are in m and t is in s.
(1) The amplitude of the wave is 5 m.
(2) The wavelength of the wave is 5 m.
(3) The frequency of the wave is 50 Hz.
( 4) The velocity of the wave is 250 m s−1
Which of the following statements are correct?A(I), (2) and (3)B(2) and (3)C(1) and (4)DAll are correct
The equation of a wave travelling on a string is y=(0.10mm)sin[3.14m−1)x+(314s−1)t]. (a) In which direction does the wave travel ? (b) Find the wave speed, the wavelength and the frequency of the wave. (c) What is the maximum displacement and the maximum speed of a portion of the string ?
View SolutionFigure shows a plot of the transverse displacements of the particles of a string at t = 0 through which a travelling wave is passing in the positive x-direction. The wave speed is 20cms−1. Find (a) the amplitude, (b) the wavelength, (c) the wave number and (d) the frequency of the wave.
 .
.
View SolutionThe equation of a wave travelling on a string is y=(0.10mm)sin[(31.4m−1)x+(314s−1)t]
(a) In which direction does the travel?
(b) Find the wave speed, the wavelength and the frequency of the wave.
( c ) What is the maximum displacement and the maximum speed of a portion of the string?
View SolutionFigure shows a plot of the transverse displacement of the particle of a string at t=0 through which a travelling wave is passing in the positive in the positive x-direction. The wave speed is 20cm/s. Find (a) the amplitude (b) the wavelength (c) the wave number and (d) the frequency of the wave.
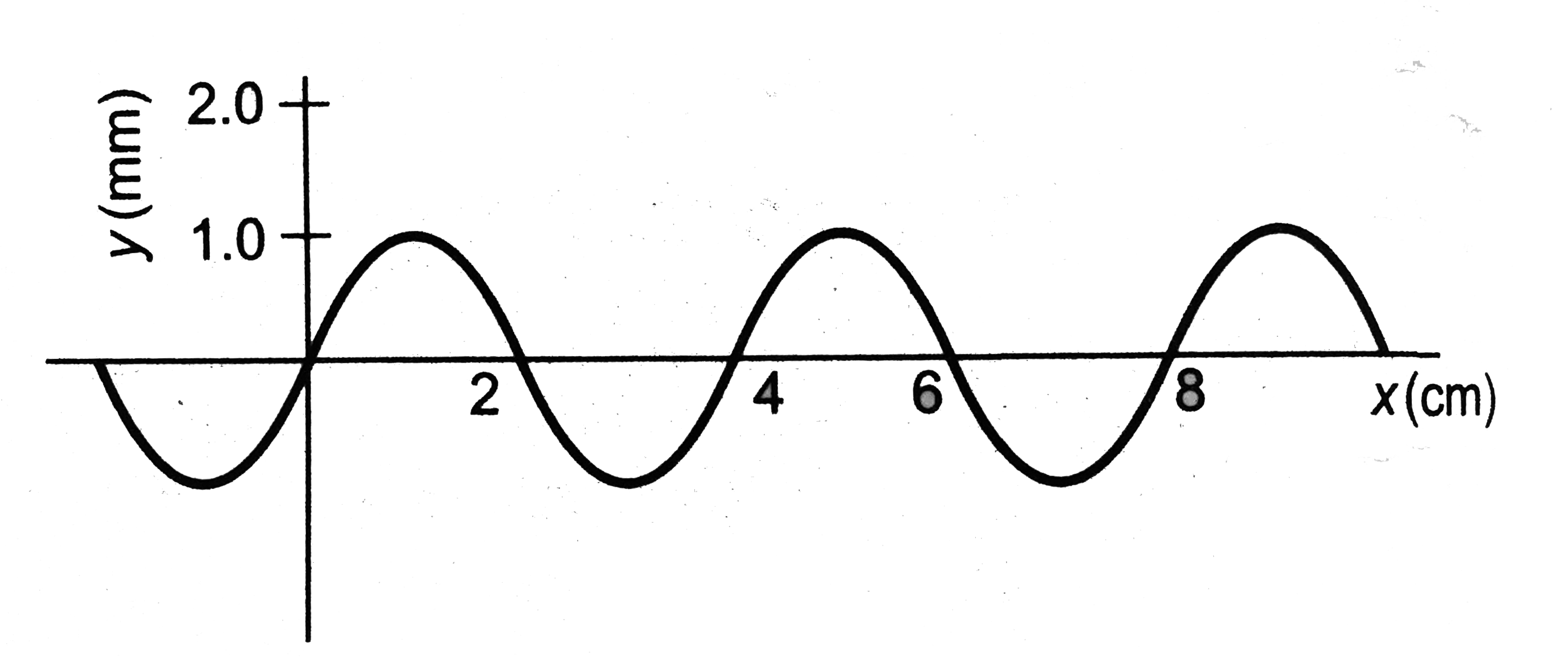
View SolutionThe displacement of a particle of a string carrying a travelling wave is given by y=(3.0cm)sin6.28(0.50x−50t), where x is in centimetre and t in second Find (a) the amplitude, (b) the wavelength, (c ) the frequency and (d) the speed of the wave.
View SolutionThe equation of a progressive wave is y=1.5sin(328t−1.27x). Where y and x are in cm and t is in second. Calcualte the amplitude, frequency, time period and wavelength of the wave.
View SolutionThe equation of progressive wave is given by y=10sin[300π(t−x480)]
where x and y are in metre and t is in second. Calculate the amplitude frequency time period and wavelength of the wave.
View Solution